NCERT Solutions for Class 7 Maths Chapter 10 Algebraic Expressions

Algebraic expressions play a crucial role in various mathematical fields. From calculating the area of a circle to calculating the prices of multiple items within a specific budget, algebra is everywhere in our daily lives. None of the higher mathematics is possible without these algebraic expressions. Algebraic expressions are a combination of constants and variables combined by arithmetic operations. The NCERT Solutions of this chapter help students understand and work on simplifying and solving algebraic expressions using arithmetic operations. These solutions are very useful for the students to practice more problems and evaluate themselves, aiding in exam preparation.

As these solutions are prepared by subject matter experts at Careers360, it is one of the most accurate study materials. These solutions give conceptual clarity encouraging students to practice more problems in this chapter. To access the solutions for each chapter of Class 7 Maths, click on NCERT Solutions for Class 7 Maths.

NCERT Solutions for Maths Chapter 10 Algebraic Expressions Class 7 - Important Points

Variable: A symbol denoted by letters (such as x, y, z, p, q, r, etc.) that can vary, representing an unknown quantity or value.

How Are Expressions Formed?

Algebraic expressions are formed by combining variables and constants using mathematical operations (Addition, Subtraction, Multiplication, and Division).

Terms and Factors of An Expression:

Terms are components of expressions formed by combining variables and constants.

The term includes the sign, so we say "added" without specifying addition or subtraction.

In the diagram, factors are shown with dotted lines and terms with continuous lines.

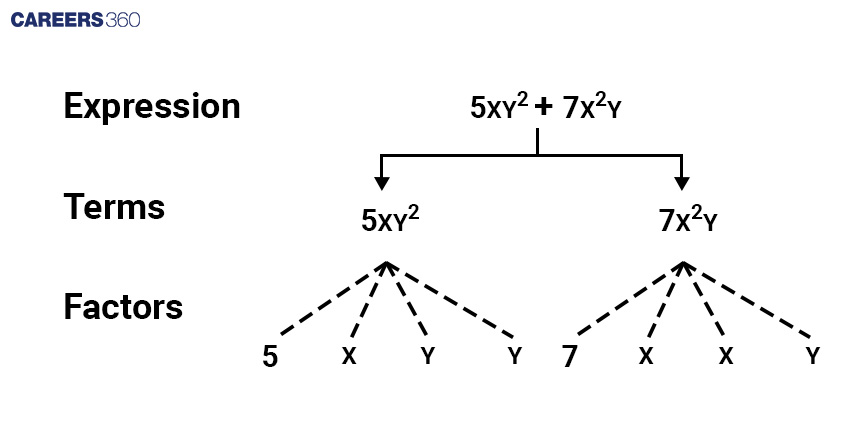
Coefficient: The coefficient is the numerical factor of a term in an expression.

Polynomial: An expression with one or more algebraic terms is called a polynomial.

Types of Polynomials

An expression with one algebraic term is called a monomial.

An expression with two algebraic terms is called a binomial.

An expression with three algebraic terms is called a trinomial.

NCERT Solutions for Maths Chapter 10 Class 7 - Downlpoad PDF

Download PDF

NCERT Solutions for Class 7 Maths Algebraic Expressions - Exercise

Question: 1(i) Get the algebraic expressions in the following cases using variables, constants and arithmetic operations.

Subtraction of $z$ from $y$ .

Answer: Subtraction of z from y: $y-z$

Question: 1(ii) Get the algebraic expressions in the following cases using variables, constants and arithmetic operations.

One-half of the sum of numbers $x$ and $y$.

Answer: Sum of numbers x and y = x + y

One-half of the sum of numbers x and y

$=\frac{x+y}{2}$

Question: 1(iii) Get the algebraic expressions in the following cases using variables, constants and arithmetic operations.

The number $z$ is multiplied by itself.

Answer:

The number $z$ multiplied by itself $=z\times z=z^2$

Question: 1(iv) Get the algebraic expressions in the following cases using variables, constants and arithmetic operations.

One-fourth of the product of numbers $p$ and $q$.

Answer: Product of the numbers p and q $=p\times q=pq$

One-fourth of the product of numbers p and q

$=\frac{pq}{4}$

Question: 1(v) Get the algebraic expressions in the following cases using variables, constants and arithmetic operations.

Numbers $x$ and $y$ are both squared and added.

Answer: Number x squared = $x^2$

Number y squared = $y^2$

Numbers x and y both squared and added = $x^2+y^2$

Question: 1(vi) Get the algebraic expressions in the following cases using variables, constants and arithmetic operations.

Number 5 is added to three times the product of numbers $m$ and $n$.

Answer: Product of numbers m and n $=m\times n=mn$

Number 5 added to three times the product of numbers $m$ and $n$ = $=3\times mn+5=3mn+5$

Question:1(vii) Get the algebraic expressions in the following cases using variables, constants and arithmetic operations.

Product of numbers $y$ and $z$ subtracted from $10$.

Answer: Product of numbers y and z $=y\times z=yz$

Product of numbers y and z subtracted from 10 $=10-yz$

Question: 1(viii) Get the algebraic expressions in the following cases using variables, constants and arithmetic operations.

The sum of numbers $a$ and $b$ subtracted from their product.

Answer: Sum of numbers a and b = a + b

Product of the numbers a and b $=a\times b=ab$

Sum of numbers $a$ and $b$ subtracted from their product $=ab-(a+b)=ab-a-b$ .

Question:2(i) Identify the terms and their factors in the following expressions

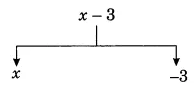
Show the terms and factors by tree diagrams. (a) $x-3$

Answer: Expression : x - 3

Terms in the above expression: x and -3

Tree diagram for the given expression

Question: 2 (i) Identify the terms and their factors in the following expressions

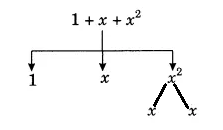
Show the terms and factors by tree diagrams (b) $1+x+x^2$

Answer: Expression: $1+x+x^2$

Terms in the above expression: $1,x$ and $x^2$

Factors of x2: x and x

Tree diagram for the given expression

Question:2(i) Identify the terms and their factors in the following expressions

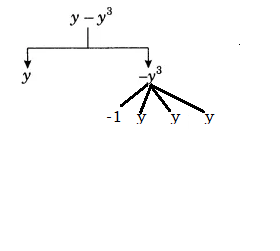
Show the terms and factors by tree diagrams (c) $y-y^3$

Answer: Expression: y - y3

Terms in the above expression: y and -y3

Factors of -y3: -1, y, y and y

Tree diagram for the given expression

Question: 2 (i) Identify the terms and their factors in the following expressions

Show the terms and factors by tree diagrams. (d) $5x{y}^2+7x^{2}y$

Answer: Expression: 5xy2 + 7x2 y

Terms in the above expression: 5xy2 and 7x2 y

Factors of 5xy2 : 5, x, y and y

Factors of 7x2 y: 7, x, x and y

Tree diagram for the given expression

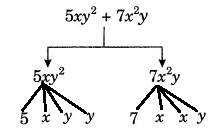
Question: 2 (i) Identify the terms and their factors in the following expressions

Show the terms and factors by tree diagrams (e) $-ab+2a{b}^2-3a^2$

Answer:

Expression: -ab + 2ab2 - 3a2

Terms in the above expression: -ab, 2ab2 and -3a2

Factors of -ab: -1, a and b

Factors of 2ab2: 2, a, b and b

Factors of -3a2: -1, 3, a and a

Tree diagram for the given expression

Question: 2 (ii) Identify terms and factors in the expressions given below:

(a) $-4x+5$

Answer: Expression: -4x + 5

Terms in the above expression: -4x and 5

Factors of -4x: -1, 4 and x

Factors of 5: 5

Question: 2 (ii) Identify terms and factors in the expressions given below:

(b) $-4x+5y$

Answer: Expression: -4x + 5y

Terms in the above expression: -4x and 5y

Factors of -4x: -1, 4 and x

Factors of 5y: 5 and y

Question: 2 (ii) Identify terms and factors in the expressions given below:

(c) $5y+3y^2$

Answer: Expression: 5y + 3y2

Terms in the above expression: 5y and 3y2

Factors of 5y: 5 and y

Factors of 3y2 : 3, y and y

Question: 2 (ii) Identify terms and factors in the expressions given below:

(d) $xy+2x^2{y}^2$

Answer: Expression: xy + 2x2 y2

Terms in the above expression: xy and 2x2 y2

Factors of xy: x and y

Factors of 2x2 y2 : 2, x, x, y and y

Question: 2 (ii) Identify terms and factors in the expressions given below:

(e) $pq+q$

Answer: Expression: pq + q

Terms in the above expression: pq and q

Factors of pq: p and q

Factors of q: q

Question: 2 (ii) Identify terms and factors in the expressions given below:

(f) $1.2ab-2.4b+3.6a$

Answer: Expression: 1.2ab - 2.4b + 3.6a

Tems in the above expression: 1.2ab, -2.4b and 3.6a

Factors of 1.2ab: 1.2, a and b

Factors of -2.4b: -1, 2.4 and b

Factors of 3.6a: 3.6 and a

Question: 2( ii) Identify terms and factors in the expressions given below:

(g) $\frac{3}{4}x+\frac{1}{4}$

Answer: Expression: $\frac{3}{4}x+\frac{1}{4}$

Terms in the above expression: $\frac{3}{4}x$ and $\frac{1}{4}$

Factors of $\frac{3}{4}x$ : $\frac{3}{4}$ and x

Factors of $\frac{1}{4}$ : $\frac{1}{4}$

Question: 2 (ii) Identify terms and factors in the expressions given below:

(h) $0.1p^2+0.2q^2$

Answer: Expression: 0.1p2 + 0.2q2

Terms in the above expression: 0.1p2 and 0.2q2

Factors of 0.1p2: 0.1, p and p

Factors of 0.2q2: 0.2, q and q

Question:3(i) Identify the numerical coefficients of terms (other than constants) in the following expressions:

$5-3t^2$

Answer: Expression: 5 - 3t2

Terms in the above expression: 5 and -3t2

Coefficient of -3t2 : -3

Question:3(ii) Identify the numerical coefficients of terms (other than constants) in the following expressions:

$1+t+t^2+t^3$

Answer: Expression: 1 + t + t2 + t3

Terms in the above expression: 1, t, t2 and t3

The coefficient of t is 1

Coefficient of t2 is 1

Coefficient of t3 is 1

Question: 3(iii) Identify the numerical coefficients of terms (other than constants) in the following expressions:

$x+2xy+3y$

Answer: Expression: x + 2xy + 3y

Terms in the above expression: x, 2xy and 3y

Coefficient of x: 1

Coefficient of 2xy: 2

Coefficient of 3y: 3

Question: 3(iv) Identify the numerical coefficients of terms (other than constants) in the following expressions:

$100m+1000n$

Answer: Expression: 100m + 1000n

Terms in the above expression: 100m and 1000n

Coefficient of 100m: 100

Coefficient of 1000n: 1000

Question: 3(v) Identify the numerical coefficients of terms (other than constants) in the following expressions:

$-p^2{q}^2+7pq$

Answer: Expression: -p2 q2 + 7pq

Terms in the above expression: -p2 q2 and 7pq

Coefficient of -p2 q2 : -1

Coefficient of 7pq: 7

Question:3(vi) Identify the numerical coefficients of terms (other than constants) in the following expressions:

$1.2a+0.8b$

Answer: Expression: 1.2a + 0.8b

Terms in the above expression: 1.2a and 0.8b

Coefficient of 1.2a: 1.2

Coefficient of 0.8b: 0.8

Question:3(vii) Identify the numerical coefficients of terms (other than constants) in the following expressions:

$3.14r^2$

Answer: Expression: 3.14r2

Terms in the above expression: 3.14r2

Coefficient of 3.14r2 is 3.14

Question: 3(viii) Identify the numerical coefficients of terms (other than constants) in the following expressions:

$2(l+b)$

Answer: Expression: 2(l + b) = 2l + 2b

Terms in the above expression: 2l and 2b

Coefficient of 2l: 2

Coefficient of 2b: 2

Question: 3(ix) Identify the numerical coefficients of terms (other than constants) in the following expressions:

$0.1y+0.01y^2$

Answer: Expression: 0.1y + 0.01y2

Terms in the above expression: 0.1y and 0.01y2

Coefficient of 0.1y is 0.1

Coefficient of 0.01y2 is 0.01

Question: 4 (a) Identify terms that contain x and give the coefficient of x.

(i) $y^{2}x+y$

Answer: Expression: y2 x + y

Terms with x: y2 x

Coefficient of x in y 2 x: y 2

Question:4(a) Identify terms that contain x and give the coefficient of x.

(ii) $13y^2-8yx$

Answer: Expression: 13y2 - 8yx

Terms with x: -8yx

Coefficient of x in -8yx: -8y

Question: 4 (a) Identify terms that contain x and give the coefficient of x.

(iii) $x+y+2$

Answer: Expression: x + y + 2

Terms with x: x

Coefficient of x in x: 1

Question: 4 (a) Identify terms that contain x and give the coefficient of x.

(iv) $5+z+zx$

Answer: Expression: 5 + z + zx

Terms with x: zx

Coefficient of x in zx: z

Question: 4 (a) Identify terms that contain x and give the coefficient of x.

(v) $1+x+xy$

Answer: Expression: 1 + x + xy

Terms with x: x and xy

Coefficient of x in x: 1

Coefficient of x in xy: y

Question:4(a) Identify terms that contain x and give the coefficient of x.

(vi) $12x{y}^2+25$

Answer: Expression: 12xy2 + 5

Terms with x: 12xy2

Coefficient of x in 12xy2 : 12y2

Question:4(a) Identify terms that contain x and give the coefficient of x.

(vii) $7x+x{y}^2$

Answer: Expression: 7x + xy2

Terms with x: 7x and xy2

Coefficient of x in 7x: 7

Coefficient of x in xy2 : y2

Question: 4 (b) Identify terms which contain $y^2$ and give the coefficient of $y^2$.

(i) $8-x{y}^2$

Answer: Expression: 8 - xy2

Terms with y2 : -xy2

Coefficient of y2 in -xy2 : -x

Question: 4 (b) Identify terms which contain $y^2$ and give the coefficient of $y^2$.

(ii) $5y^2+7x$

Answer: Expression: 5y2 + 7x

Terms with y2: 5y2

Coefficient of y2 in 5y2 : 5

Question: 4 (b) Identify terms which contain $y^2$ and give the coefficient of $y^2$.

(iii) $2x^{2}y-15x{y}^2+7y^2$

Answer: Expression: 2x2 y -15xy2 + 7y2

Terms with y2 : -15xy2 and 7y2

Coefficient of y2 in -15xy2 : -15x

Coefficient of y2 in 7y2 : 7

Question:5 Classify into monomials, binomials and trinomials.

(i) 4y – 7z

(ii) y2

(iii) x + y – xy

(iv) 100

(v) ab – a – b

(vi) 5 – 3t

(vii) 4p2 q – 4pq2

(viii) 7mn

(ix) z2 – 3z + 8

(x) a2 + b2

(xi) z2 + z

(xii) 1 + x + x2

Answer: (i) 4y – 7z

Binomial

(ii) y2

Monomial

(iii) x + y – xy

Trinomial

(iv) 100

Monomial

(v) ab – a – b

Trinomial

(vi) 5 – 3t

Binomial

(vii) 4p2 q – 4pq2

Binomial

(viii) 7mn

Monomial

(ix) z2 – 3z + 8

Trinomial

(x) a2 + b2

Binomial

(xi) z2 + z

Binomial

(xii) 1 + x + x2

Trinomial

Question:6(i) State whether a given pair of terms is of like or unlike terms.

$1,100$

Answer: (i) $1,100$ are like terms.

Question:6(ii) State whether a given pair of terms is of like or unlike terms.

$-7x,\frac{5}{2}x$

Answer: (ii) $-7x,\frac{5}{2}x$ are Like terms

Question:6(iii) State whether a given pair of terms is of like or unlike terms.

$-29x,-29y$

Answer: Since y and x are unlike terms

Question: 6(iv) State whether a given pair of terms is of like or unlike terms.

$14xy,42yx$

Answer: Like terms, since both the terms contain xy and only the coefficient is different

Question: 6(v) State whether a given pair of terms is of like or unlike terms.

$4m^{2}p,4m{p}^2$

Answer: Unlike since $m^{2}pandm{p}^2$ are different

Question: 6(vi) State whether a given pair of terms is of like or unlike terms.

$12xz,12x^2{z}^2$

Answer: Unlike since $xzand{x}^{2}z$ are unlike terms

Question:7(a) Identify like terms in the following:

$-x{y}^2,-4y{x}^2,8x^2,2x{y}^2,7y,-11x^2,-100x,-11yx,20x^{2}y,-6x^2,y,2xy,3x$

Answer: Like terms are

(i) -xy2 and 2xy2

(ii) -4x2 y and 20x2 y

(iii) 8x2 , -11x2 and -6x2

(iv) 7y and y

(v) -100x and 3x

(vi) -11yx and 2xy

Question:7(b) Identify like terms in the following:

$10pq,7p,8q,-p^2{q}^2,-7qp,-100q,-23,12q^2{p}^2,-5p^2,$
$41,2405p,78qp,13p^{2}q,q{p}^2,701p^2$

Answer: Like terms are

(i) 10pq, -7qp and 78qp

(ii) 7p and 2405p

(iii) 8q and -100q

(iv) -p2 q2 and 12q2 p2

(vii) -23 and 41

(viii) -5p2 and 701p2

(ix) 13p2 q and qp2

Question: 1(i) If $m=2,$ find the value of:

$m-2$

Answer: (i) m - 2

= 2 - 2

= 0

If m = 2, the value of m - 2 = 0

Question: 1(ii) If $m=2,$ find the value of:

$3m-5$

Answer:

$$\begin{gathered} 3m-5 \\ =3\times 2-5 \\ =6-5 \\ =1 \end{gathered}$$

If m = 2, the value of 3m - 5 = 1

Question:1(iii) If $m=2,$ find the value of:

$9-5m$

Answer:

$$\begin{gathered} 9-5m \\ =9-5\times 2 \\ =9-10 \\ =-1 \end{gathered}$$

If m = 2, the value of 9 - 5m = -1

Question: 1(iv) If $m=2,$ find the value of:

$3m^2-2m-7$

Answer:

$$\begin{gathered} 3m^2-2m-7 \\ =3\times 2^2-2\times 2-7 \\ =12-4-7 \\ =1 \end{gathered}$$

If m = 2 the value of 3m2 - 2m - 7 = 1

Question: 1(v) If find the value of: (v) If $m=2,$ find the value of:

$\frac{5m}{2}-4$

Answer:

$\frac{5m}{2}-4$

$=\frac{5\times 2}{2}-4$

= 5 - 4

= 1

If m = 2 the value of $\frac{5m}{2}-4=1$

Question: 2(i) If $p=-2,$ find the value of:

$4p+7$

Answer:

$$\begin{gathered} 4p+7 \\ =4\times (-2)+7 \\ =-8+7 \\ =-1 \end{gathered}$$

If p = -2 the value of 4p + 7 = -1

Question: 2(ii) If $p=-2,$ find the value of:

$-3p^2+4p+7$

Answer:

$-3p^2+4p+7$
$=-3x(-2{)}^2+4x(-2)+7$
$=-12-8+7$
$=-13$

If p = -2 the value of -3p2 + 4p + 7 = -13

Question: 2(iii) If $p=-2$ , find the value of:

$-2p^3-3p^2+4p+7$

Answer:

$-2p3-3p2+4p+7$
$=-2\times (-2{)}^3-3\times (-2{)}^2+4\times (-2)+7$
$=16-12-8+7$
$=3$

If p = -2 the value of -2p 3 - 3p 2 + 4p + 7 = 3

Question: 3(i) Find the value of the following expressions, when $x=-1$ :

$2x-7$

Answer:

$$\begin{gathered} 2x-7 \\ =2\times (-1)-7 \\ =-2-7 \\ =-9 \end{gathered}$$

If x = -1 the value of 2x - 7 = -9

Question: 3(ii) Find the value of the following expressions, when

$x=-1:$

$-x+2$

Answer:

-x + 2

= -( -1 ) + 2

= 1 + 2

= 3

If x = -1 the value of -x + 2 = 3

Question: 3(iii) Find the value of the following expressions, when $x=-1$ :

$x^2+2x+1$

Answer:

${x}^2+2x+1$
$=(-1{)}^2+2\times (-1)+1$
$=1-2+2$
$=0$

If x = -1 the value of x2 + 2x + 1 = 0

Question:3 (iv) Find the value of the following expressions when $x=-1$ :

$2x^2-x-2$

Answer:

$2x^2-x-2$
$=2\times (-1{)}^2-(-1)-2$
$$\begin{gathered} =2+1-2 \\ =1 \end{gathered}$$

So the value at x=-1 is 1

Question: 4(i) If $a=2,b=-2,$ find the value of:

$a^2+b^2$

Answer:

a2 + b2

= ( 2 )2 + ( -2 )2

= 4 + 4

= 8

If a = 2 and b = -2 the value of a2 + b2 = 8

Question: 4(ii) If $a=2,b=-2,$ find the value of:

$a^2+ab+b^2$

Answer:

${a}^2+ab+b^2$
$=2^2+2\times (-2)+(-2{)}^2$
$$\begin{gathered} =4-4+4 \\ =4 \end{gathered}$$

If a = 2 and b = -2 the value of a2 + ab + b2 = 4

Question: 4(iii) If $a=2,b=-2,$ find the value of

$a^2-b^2$

Answer:

a2 - b2

= 22 - ( -2 )2

= 4 - 4

= 0

If a = 2 and b = -2 the value of a2 - b2 = 0

Question: 5(i) When $a=0,b=-1,$ find the value of the given expressions:

$2a+2b$

Answer:

$$\begin{gathered} 2a+2b \\ =2\times 0+2\times (-1) \\ =0-2 \\ =-2 \end{gathered}$$

When a = 0 and b = -1 the value of the given expression 2a + 2b = -2

Question: 5(ii) When $a=0,b=-1,$ find the value of the given expressions:

$2a^2+b^2+1$

Answer:

$2a^2+b^2+1$
$=2\times 0^2+(-1)2+1$
$$\begin{gathered} =0+1+1 \\ =2 \end{gathered}$$

When a = 0 and b = -1 the value of the given expression 2a2 + b2 + 1 = 2

Question: 5(iii) When $a=0,b=-1$ , find the value of the given expressions:

$2a^{2}b+2a{b}^2+ab$

Answer:

$2a^{2}b+2a{b}^2+ab$
$=2\times 0^2\times (-1)+2\times 0\times (-1{)}^2+0\times (-1)$

$$\begin{gathered} =0+0+0 \\ =0 \end{gathered}$$

When a = 0 and b = -1 the value of the given expression 2a2 b + 2ab2 + ab = 0

Question:5 (iv) When $a=0,b=-1,$ find the value of the given expressions:

$a^2+ab+2$

Answer:

$$\begin{gathered} {a}^2+ab+2 \\ =0^2+0\times (-1)+2 \end{gathered}$$

$$\begin{gathered} =0+0+2 \\ =2 \end{gathered}$$

When a = 0 and b = -1 the value of the given expression a2 + ab + 2 = 2

Question: 6(i) Simplify the expressions and find the value if $x$ is equal to $2$

$x+7+4(x-5)$

Answer:

$x+7+4(x-5)$
$=x+7+4x-20$

$$\begin{gathered} =5x-13 \\ =5\times 2-13 \end{gathered}$$

$$\begin{gathered} =10-13 \\ =-3 \end{gathered}$$

If x is equal to 2 the value of x + 7 + 4( x - 5 ) = -3

Question: 6(ii) Simplify the expressions and find the value if $x$ is equal to $2$

$3(x+2)+5x-7$

Answer:

$3(x+2)+5x-7$
$=3x+6+5x-7$

$$\begin{gathered} =8x-1 \\ =8\times (2)-1 \end{gathered}$$

$$\begin{gathered} =16-1 \\ =15 \end{gathered}$$

If x is equal to 2 the value of 3( x + 2 ) + 5x - 7 = 15

Question: 6(iii) Simplify the expressions and find the value if $x$ is equal to $2$

$6x+5(x-2)$

Answer:

$6x+5(x-2)$
$=6x+5x-10$

$$\begin{gathered} =11x-10 \\ =11\times 2-10 \end{gathered}$$

$$\begin{gathered} =22-10 \\ =12 \end{gathered}$$

If x is equal to 2 the value of 6x + 5( x - 2 ) = 12

Question: 6(iv) Simplify the expressions and find the value if $x$ is equal to $2$

$4(2x-1)+3x+11$

Answer:

$$\begin{gathered} 4(2x-1)+3x+11 \\ =8x-4+3x+11 \end{gathered}$$

$$\begin{gathered} =11x+7 \\ =11\times 2+7 \end{gathered}$$

$$\begin{gathered} =22+7 \\ =29 \end{gathered}$$

If x is equal to 2 the value of 4( 2x - 1 ) + 3x + 11 = 29

Question: 7 Simplify these expressions and find their values if

$x=3,a=-1,b=-2.$

(i) $3x-5-x+9$

(ii) $2-8x+4x+4$

(iii) $3a+5-8a+1$

(iv) $10-3b-4-5b$

(v) $2a-2b-4-5+a$

Answer:

The expression is simplified as follows and also obtained their values

(i)

$$\begin{gathered} 3x-5-x+9 \\ =2x+4 \end{gathered}$$

$$\begin{gathered} =2\times 3+4 \\ =10 \end{gathered}$$

(ii)

$$\begin{gathered} 2-8x+4x+4 \\ =6-4x \end{gathered}$$

$$\begin{gathered} =6-4\times 3 \\ =-6 \end{gathered}$$

(iii)

$$\begin{gathered} 3a+5-8a+1 \\ =-5a+6 \end{gathered}$$

$$\begin{gathered} =-5\times (-1)+6 \\ =5+6 \\ =11 \end{gathered}$$

(iv)

$$\begin{gathered} 10-3b-4-5b \\ =6-8b \end{gathered}$$

$$\begin{gathered} =6-8\times (-2) \\ =6+16 \\ =22 \end{gathered}$$

(v)

$$\begin{gathered} 2a-2b-4-5+a \\ =3a-2b-9 \end{gathered}$$

$=3\times (-1)-2\times (-2)-9$
$$\begin{gathered} =-3+4-9 \\ =-8 \end{gathered}$$

Question: 8 (i) If $z=10,$ find the value of

$z^3-3(z-10)$

Answer:

${z}^3-3(z-10)$
$=z^3-3z+30$

$={10}^3-3\times 10+30$
$$\begin{gathered} =1000-30+30 \\ =1000 \end{gathered}$$

If z = 10 the value of z3 - 3( z - 10 ) = 1000

Question: 8 (ii) If $p=-10,$ find the value of

$p^2-2p-100$

Answer:

${p}^2-2p-100$
$=(-10{)}^2-2\times (-10)-100$

$$\begin{gathered} =100+20-100 \\ =20 \end{gathered}$$

If p = -10 the value of p2 - 2p - 100 = 20

Question: 9 What should be the value of a if the value of $2x^2+x-a$ equals to $5$, when

$x=0?$

Answer:

$2x^2+x-a=5$
$2\times 0^2+0-a=5$
$-a=5$
$a=-5$

Therefore, for a = -5, when the value of x=0

Algebraic Expressions Class 7 Chapter 10-Topics

• How Are Expressions Formed?
• Terms Of An Expression
• Like And Unlike Terms
• Monomials, Binomials, Trinomials And Polynomials
• Addition And Subtraction Of Algebraic Expressions
• Finding The Value Of An Expression
• Using Algebraic Expressions – Formulas And Rules

NCERT Solutions for Class 7 Maths Chapter 10 Algebraic Expressions - Points to Remember

• A variable is a symbol denoted by alphabets representing an unknown quantity
• Algebraic Expressions are combinations of variables and constants connected by arithmetic operations
• An expression with one or more algebraic terms is called a polynomial
• An expression with -
  One algebraic term is monomial
  Two algebraic terms are binomial
  Three algebraic terms are trinomial

NCERT Solutions for Class 7 Maths Chapter Wise

NCERT Solutions for Class 7 Subject Wise

The NCERT Solutions for Class 7 subject-wise is one of the essential study materials that helps students to practice more problems in each subject of Class 7. Click on the link below to check out the subject-wise solutions.

NCERT Books and NCERT Syllabus

• NCERT Syllabus Class 7 Maths
• NCERT Books Class 7
• NCERT Syllabus Class 7