CBSE Class 10: How Quadratic Equations Can Help You Make A Shot In Basketball

Quadratic equations are more than just mathematical concepts; they are the hidden architects behind the mesmerising art of making the shot during a basketball game. As you step into the world of quadratic equations in your CBSE Class 10 mathematics curriculum, you'll find these equations to be fundamental building blocks that unveil the science behind a simple basketball shot. This article will be your guide to unravelling the connection between quadratic equations and the elegant trajectory of a basketball in flight.

Understanding Quadratic Equations: The Basics

Before we delve into the world of basketball, let's revisit the basics of quadratic equations. A quadratic equation is a second-order polynomial equation containing a single variable 'x'. It typically looks like this: ax² + bx + c = 0

Here, 'x' is the variable, and a, b, and c are constants. It's important to note that 'a' should not be equal to 0. The solutions to this equation are the values of 'x' that satisfy the equation and make it equal to zero. These solutions are known as the "roots" of the quadratic equation.

Toolkit For Solving Equations

Solving quadratic equations is like solving a puzzle. Sometimes, the equation can be easily factored in, while other times, you need a more sophisticated approach like completing the square and quadratic formula. Let's discuss these three techniques in detail.

>>> Factoring is a technique that involves breaking down a quadratic equation into simpler factors. It's like taking apart a Lego structure to understand its individual pieces. When an equation is factorable, it means you can write it as a product of two binomials (expressions with two terms). Here's how it works:

Consider a quadratic equation: ax² + bx + c = 0

• Identify a, b, and c from the equation.

• Look for two numbers that multiply to 'a * c' and add up to 'b'.

• Express the middle term ('bx') using the two numbers you found in the previous step.

• Factor by grouping and set each factor equal to zero.

Let's see an example:

Equation: x² + 5x + 6 = 0

• a = 1, b = 5, and c = 6.

• Numbers that multiply to '1 * 6' and add up to '5' are '2' and '3'.

• Rewrite '5x' as '2x + 3x'.

• Factor: (x + 2)(x + 3) = 0

Now, set each factor equal to zero:

x + 2 = 0 --> x = -2

x + 3 = 0 --> x = -3

So, the solutions are x = -2 and x = -3.

>>> Completing The Square is like transforming a quadratic equation into a perfect square trinomial. It's a bit more intricate, but it's a powerful technique. Here's how it's done:

• Start with the quadratic equation in the form: ax² + bx + c = 0

• Divide the entire equation by 'a' to make the coefficient of 'x²' equal to 1.

• Move the constant term ('c/a') to the other side of the equation.

• Add and subtract the square of half of the coefficient of the 'x' term ('b/2a').

The equation will then be in the form: (x + b/2a)² = d

Solve for 'x' to find the solutions.

Let's go through an example:

Equation: x² - 6x + 8 = 0

• Divide by 'a': x² - 6x + 8 = 0

• Move constant term: x² - 6x = -8

• Add and subtract ('-6/2 * 1')² = 9

x² - 6x + 9 = -8 + 9

(x - 3)² = 1

Now, take the square root of both sides:

x - 3 = √1

x - 3 = ±1

Solutions: x = 3 ± 1 that is x = 4 and x = 2

>>> Quadratic Formula is your trusty toolkit for finding the roots of a quadratic equation when it isn't easily factorable.

The formula goes as follows: x = (-b ± √(b² - 4ac)) / 2a

In this formula, a, b, and c are the coefficients from the original quadratic equation. The "±" symbol represents the two possible solutions, and the square root calculates the discriminant, which gives us insights into the nature of the roots.

Also Check - Smart Shopping CBSE Class 8: Comparing Quantities For Better Deals

Discriminant and Root Classification

The discriminant (Δ) of a quadratic equation plays a crucial role in categorising its roots. The formula to calculate the discriminant is Δ = b² - 4ac

Based on the value of Δ, we can determine the nature of the roots:

• If Δ > 0, the equation has two distinct real roots.

• If Δ = 0, the equation has a single real root (a repeated root).

• If Δ < 0, the equation has two complex conjugate roots.

Understanding the discriminant helps us predict the behaviour of the equation without actually solving it.

Quadratic Equation Graphs

Graphs are powerful visual representations that provide insights into the behaviour of quadratic equations. By analysing the shape and key points of these graphs, we can understand how different coefficients and constants in quadratic equations affect their curves. Let's explore the graphs of quadratic equations in various cases.

Case 1 - When 'a' is Positive: When 'a' is positive, the graph of the quadratic equation opens upwards. This means the parabola has a minimum point. The larger the value of 'a', the narrower the parabola.

Example: y = x²

Case 2 - When 'a' is Negative: When 'a' is negative, the graph of the quadratic equation opens downwards. The parabola has a maximum point. Again, the smaller the value of 'a', the wider the parabola.

Example: y = -x²

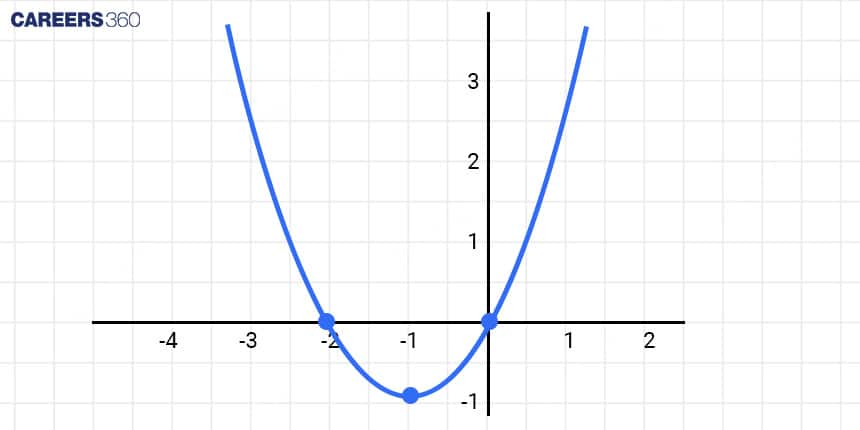
Case 3 - When 'b' is Positive or Negative: The coefficient 'b' determines the horizontal shift of the parabola. If 'b' is positive, the parabola shifts to the left, and if 'b' is negative, it shifts to the right.

Examples:

• Positive 'b': y = x² - 2x

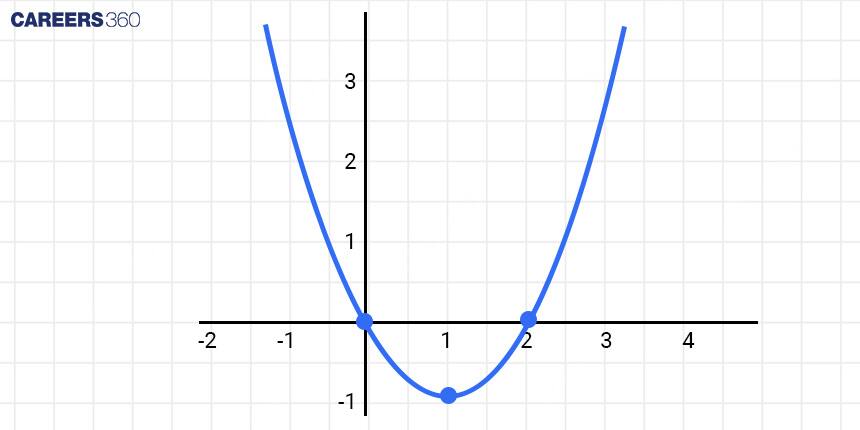
• Negative 'b': y = x² + 2x

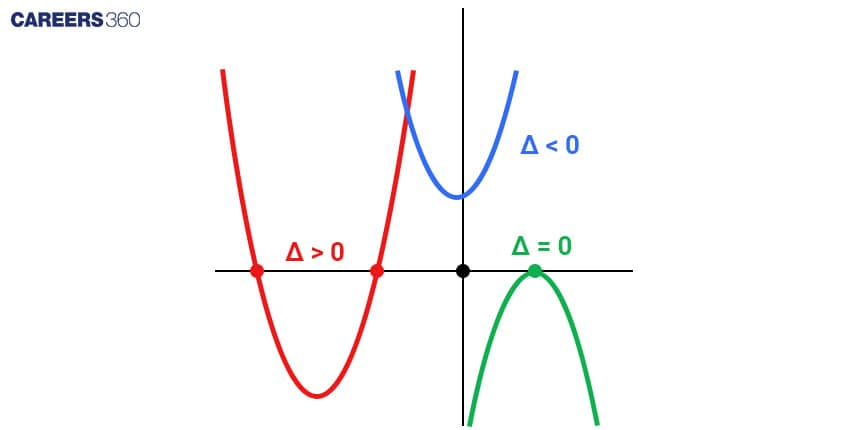
Case 4 - Discriminant and Nature of Roots: The discriminant (Δ) of a quadratic equation affects the number and nature of its roots. We can visualise this on the graph.

Δ > 0: Two real and distinct roots. The parabola intersects the x-axis at two points.

Δ = 0: One real repeated root. The parabola touches the x-axis at one point.

Δ < 0: Two complex conjugate roots. The parabola does not intersect the x-axis.

From Equations to Basketball: The Trajectory of a Shot

Now, let's transition from mathematics to the basketball court. When a basketball player takes a shot, the ball's path follows a curved trajectory. This trajectory is a result of the initial velocity of the shot and the force of gravity pulling the ball downwards. This curving path is aptly described by quadratic equations.

Mathematics enables us to break down the elements of a basketball shot.

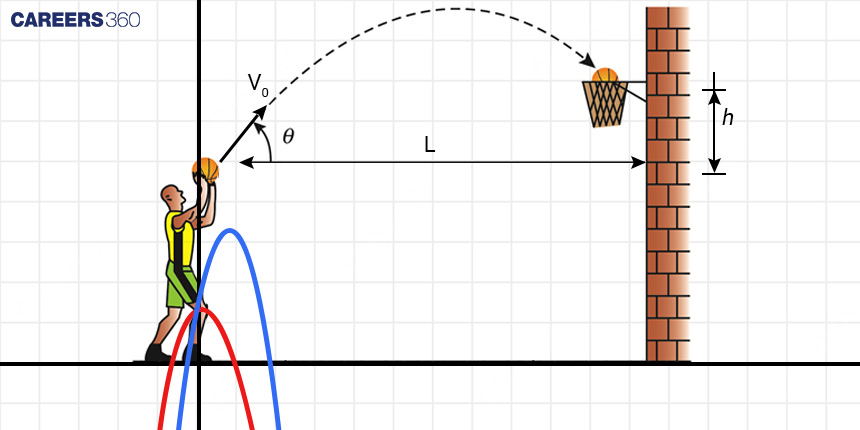
We can observe that the parabola is downward open, therefore ‘a’ should be negative. and vertical position 'y' of the ball over time 't' can be modelled using the equation:

y = -1/2gt² + v₀sinθ t + h₀

x = v₀cosθ t

y = xtanθ -gx²/(2(v₀cos θ)²)+h₀

Other form

y= -16t²+v₀sinθt + h₀

Here, 'v₀' represents the initial velocity of the shot, h₀ is the initial height of the ball, and g is the acceleration due to gravity. This equation captures the essence of the ball's trajectory, allowing us to analyse its behaviour.

Also Check - Why You Should Give Mock Tests Even For Banking Exams?

The Quest for the Perfect Shot

A basketball player's ultimate goal is to nail the perfect shot – a shot that sails through the hoop with finesse. Achieving this requires understanding the interplay between the initial velocity 'v' and the angle of release. Quadratic equations hold the key to determining the parabolic arc that the ball will follow.

Different angles and velocities lead to various trajectories. By tinkering with these variables, players can fine-tune their shots. Quadratic equations help them comprehend the mechanics behind their shots and make informed decisions to enhance their accuracy.

Consider a basketball player who is aiming for a hoop placed at a height of 15 feet. The player of height 5 feet, wants to shoot the ball at an angle of 45 degrees and an initial velocity of 50 feet per second. How far from the hoop should the player stand to make the shot?

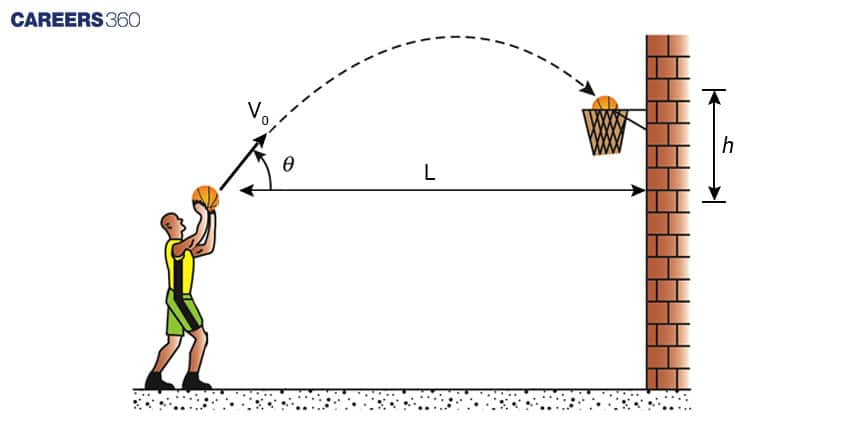
let's break down the key components of the problem:

• Initial angle of release (θ): 45 degrees

• Initial velocity (v0): 50 feet per second

• Height of the hoop (h): 15 feet - 5 feet = 10 feet (above the person)

• Acceleration due to gravity (g): 32 feet per second squared (negative since it's acting downward)

Quadratic equation for basketball written as

y = -16t² + v₀sinθt +h₀

After time t ball will be in basket so

15 = -16t² + 50 sin45 t + 5

-16t² + 50/√2 t - 10 = 0

-16t² + 35.21 t - 10 = 0

Using the formula x = (-b ± √(b² - 4ac)) / 2a

t = (-35.21 ± √(35.212 - 4(-16)(-10))) / {2(-16)}

t = (-35.21 ± 24.49)/(-32)

= 1.87 sec (time can not be negative)

x = v₀cosθ t

x = 50 cos 45 (1.87)

65.85 feet

So, the basketball player should stand approximately 65.85 feet from the hoop to make a successful shot with an angle of 45 degrees and an initial velocity of 50 feet per second.

Understanding quadratic equations empowers players to adapt to different court positions, distances, and angles. Armed with this knowledge, players can adjust their shots dynamically, improving their overall accuracy and consistency.