NCERT Solutions for Exercise 11.2 Class 9 Maths Chapter 11 - Constructions
NCERT Solutions for Class 9 Maths chapter 11 exercise 11.2 introduces us with the construction of triangles till now we have learned about how to construct the bisector of a given angle, and the perpendicular bisector of a given line segment must be constructed. and to create a 60-degree angle at the starting point of a given ray.NCERT solutions for Class 9 Maths chapter 11 exercise 11.2 is based on the construction of triangles using the prior knowledge of exercise 11.1 Class 9 Maths and the congruence of triangles as covered in chapter seventh Class 9. All the construction in the NCERT solutions for Class 9 Maths chapter 11 exercise 11.2 is carried out with the help of compass and ruler but we can use a protector to re-check if construction is accurate. Actually, it is very helpful as it cross-examines the whole construction. Along with Class 9 Maths chapter 11 exercise 11.2, the following exercises are also present.
This Story also Contains
- Constructions Class 9 Maths Chapter 11 Excercise: 11.2
- Q1 Construct a triangle ABC in which $BC = 7cm$ , $\angle B = 75^\circ$ and $AB + AC = 13 cm$ .
- More About NCERT Solutions for Class 9 Maths Exercise 11.2
- Benefits of NCERT Solutions for Class 9 Maths Exercise 11.2
- NCERT Solutions of Class 10 Subject Wise
- NCERT Solutions for Class 9 Maths
Constructions Class 9 Maths Chapter 11 Excercise: 11.2
Q1 Construct a triangle ABC in which $BC = 7cm$ , $\angle B = 75^\circ$ and $AB + AC = 13 cm$ .
Answer:
The steps of construction are as follows:
Step 1: Draw a line segment BC of 7cm length. Taking the help of protractor make an $\angle XBC = 75^{\circ}$ .
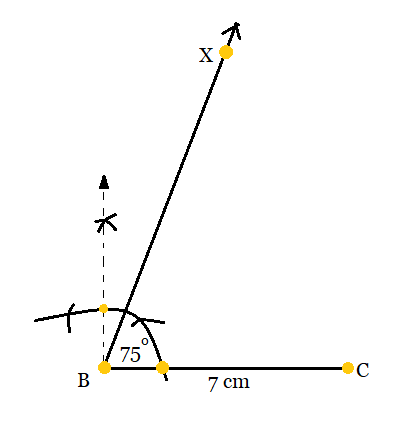
Step 2: Now, cut a line segment BD having 13 cm on BX which is $(AB+AC).$
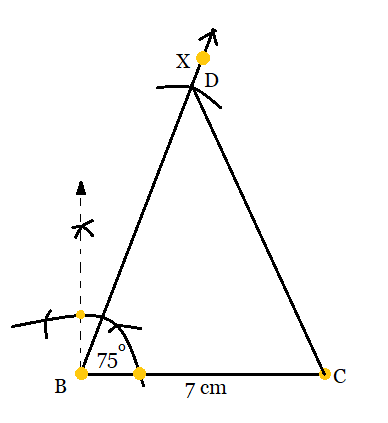
Step 3: Now, join CD.
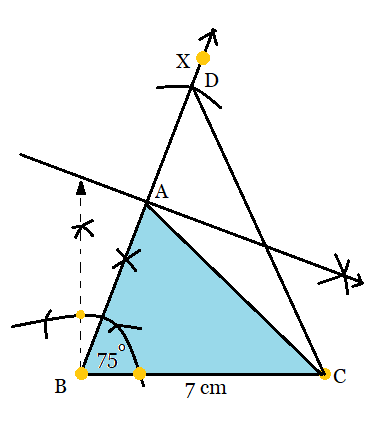
Step 4: Draw a perpendicular bisector of CD to intersect BD at a point A. Join AC. Then ABC is the required triangle
Hence, the required triangle is ABC.
Q2 Construct a triangle ABC in which $BC = 8cm$ , $\angle B = 45^\circ$ ° and $AB - AC = 3.5cm$
Answer:
The steps of construction to be followed:
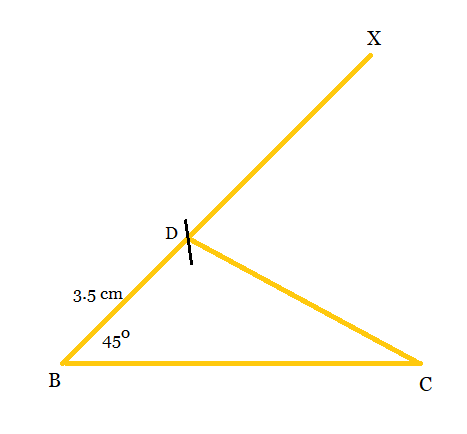
Step 1: Draw a line segment BC = 8cm and make an angle of $45^{\circ}$ at point B i.e., $\angle XBC.$
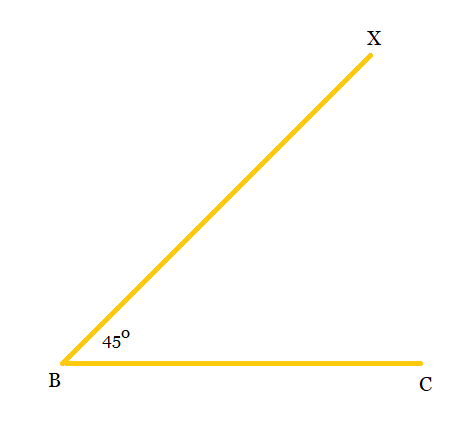
Step 2: Now, cut the line segment BD = 3.5 cm on ray BX. i.e. $(AB-AC)$ .
Step 3: Join CD and draw a perpendicular bisector of CD i.e., PQ.
Step 4: Let the perpendicular bisector of CD intersects BX at point A. Then,
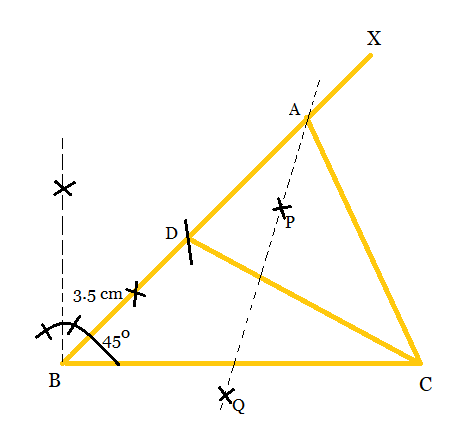
Step 5: Join AC, to get the required triangle $\triangle ABC.$
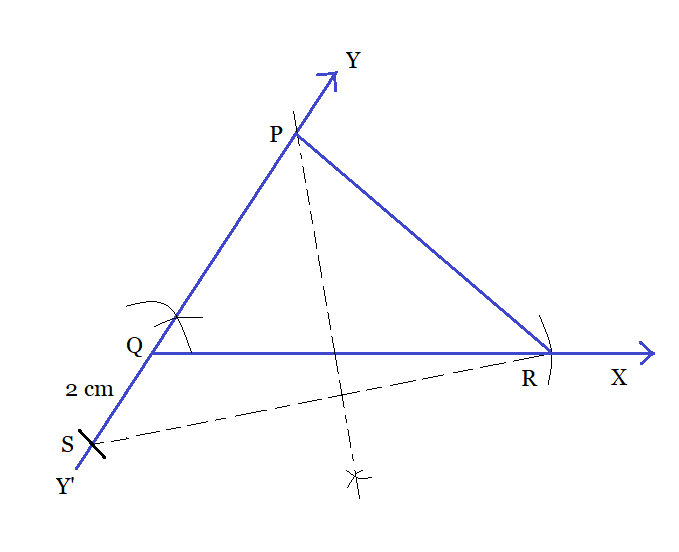
Q3 Construct a triangle PQR in which $QR = 6cm$ , $\angle Q = 60 ^\circ$ and $PR -PQ = 2cm$ .
Answer:
The steps of construction to be followed:
Step 1: Draw a ray QX and cut off a line segment QR which is equal to 6 cm in length.
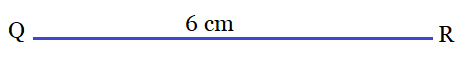
Step 2: With an angle of $60^{\circ}$ with QR, construct a ray QY and extend it to form a line YQY'.
Step 3: Now, cut off a line segment QS equal to 2 cm from QY' and join RS.
Y
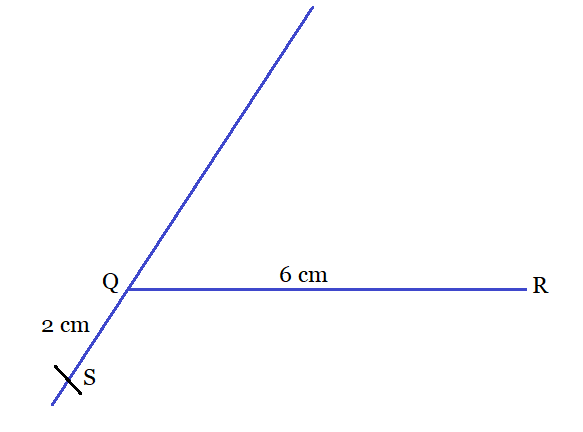
Y'
Step 4: Draw a perpendicular bisector of RS which intersects QY at a point P.
Step 5: Join PR to get the required triangle $\triangle PQR.$
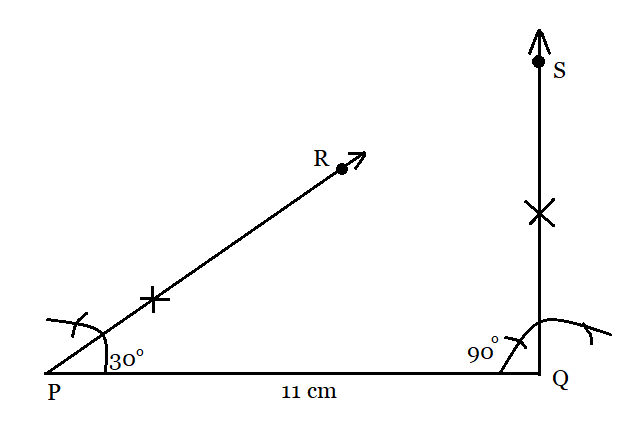
Answer:
The steps of construction to be followed:
Step 1: For given $XY+YZ+ZX = 11 cm$ , a line segment $PQ =11 cm$ is drawn.
Step 2: At points, P and Q angles of $\angle RPQ = 30^{\circ}$ and $\angle SQP =90^{\circ}$ are constructed respectively.
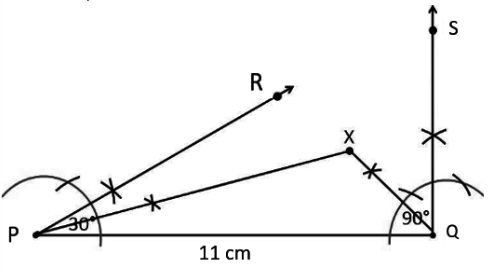
Step 3: Now, bisects the angle RPQ and SQP. The bisectors of these angles intersect each other at a point X.
Step 4: Construct the perpendicular bisector of PX and QX, name them as TU and WV respectively.
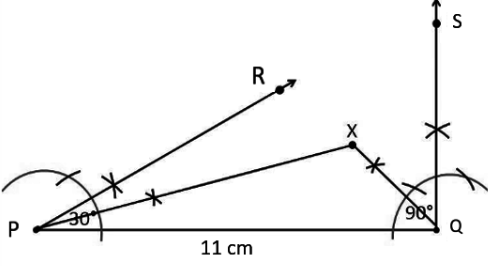
Step 5: Let the bisector TU intersect PQ at Y and bisector WV intersect PQ at Z. Then XY and ZY are joined.
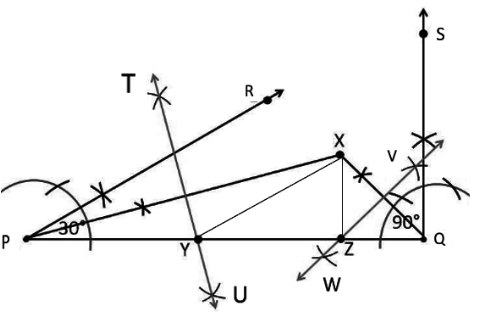
Therefore, $\triangle XYZ$ is the required triangle.
Q5 Construct a right triangle whose base is 12cm and sum of its hypotenuse and other side is 18 cm.
Answer:
The steps of construction to follow:
Step 1: Draw a ray BX and Cut off a line segment $BC=12cm$ from the ray.

Step 2: Now, construct an angle $\angle XBY = 90^{\circ}$ .

Step 3: Cut off a line segment BD of length 18 cm on BY. Then join the CD.
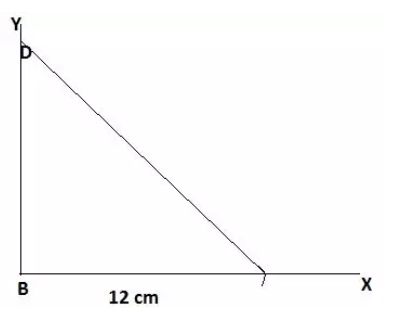
Step 4: Now, construct a perpendicular bisector of CD which intersects BD at A and AC is joined.
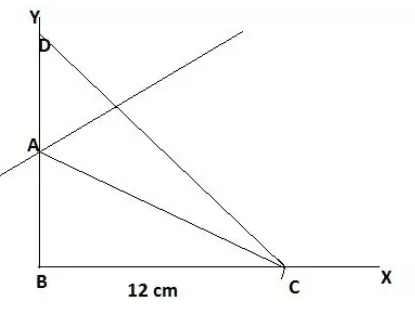

Thus, the constructed triangle is ABC.
More About NCERT Solutions for Class 9 Maths Exercise 11.2
Class 9 Maths chapter 11 exercise 2 covers the construction of triangles with the various different components . These constructions include : Construction of creating a triangle given its base, a base angle, and the difference of the other two sides, and constructing a triangle given its base, a base angle, and the sum of the other two sides Given the perimeter and two base angles of a triangle, build it.. There are always more than one way to solve a construction question so there are many alternative methods for construction methods but the final result should be the same . . There should be at least three parts of a triangle that have to be given in question for constructing it but not all combinations of three parts are sufficient for the purpose. For example, if two sides and an angle (not the included angle) are given, then it is not always possible to construct such a triangle uniquely.so there should be three consecutive parts of triangle should be given.
Also Read| Constructions Class 9 Notes
Benefits of NCERT Solutions for Class 9 Maths Exercise 11.2
Class 9 Maths chapter 11 exercise 11.2 covers the topic of construction of triangle in three different way as provided in Class 9 Maths chapter 11 exercise 11.2
NCERT Class 9 Maths chapter 11 exercise 11.2 , will be helpful in Class 11 Maths chapter 11 construction.
Construction is a very important topic of an entrance exam JEE Main (joint entrance exam - 2) known as B-arch
Construction is base engineering in physics and mechatronic
Also See:
NCERT Solutions of Class 10 Subject Wise
Popular Questions
Courses After 12th
Applications for Admissions are open.
This ebook serves as a valuable study guide for NEET 2025 exam.
NEET Previous 10 Year Questions
Get nowThis e-book offers NEET PYQ and serves as an indispensable NEET study material.
JEE Main Important Physics formulas
Get nowAs per latest syllabus. Physics formulas, equations, & laws of class 11 & 12th chapters
JEE Main Important Chemistry formulas
Get nowAs per latest syllabus. Chemistry formulas, equations, & laws of class 11 & 12th chapters
JEE Main high scoring chapters and topics
Get nowAs per latest 2024 syllabus. Study 40% syllabus and score upto 100% marks in JEE
JEE Main Important Mathematics Formulas
Get nowAs per latest syllabus. Maths formulas, equations, & theorems of class 11 & 12th chapters
