ALLEN Coaching
ApplyRegister for ALLEN Scholarship Test & get up to 90% Scholarship
Have you wondered how butterflies have identical wings on both sides or how some logos or other shapes have identical parts??? All this is because of the concept called symmetry. From butterflies, flowers, buildings, and some patterns to even the human body, symmetry is everywhere around us. This chapter about symmetry helps students understand what symmetry is and how shapes and designs are divided into identical halves. Symmetry has widespread applications in fields like Geometry, Art, Computer Graphics, Architecture, etc. The NCERT Solutions for this chapter provide the students with a deep understanding of the concepts of symmetry, its properties and its applications.
The solutions in this article are designed by subject matter experts of Careers360, making sure that the solutions are highly accurate and reliable. These solutions help the students to identify different types of symmetry and order of symmetry with high conceptual clarity. The NCERT Solutions for Class 7 Maths provides the solution for all the chapters of Class Maths solved by the subject matter experts.
Line Symmetry:
If it can be divided into two identical parts by a line, there will be a line of symmetry.
Regular polygons have equal angles and equal sides so they have multiple lines of symmetry. The table given below shows the number of lines of symmetry in regular polygons.
Regular Polygons | Regular Hexagon | Regular Pentagon | Square | Equilateral Triangle |
Number of Lines of Symmetry | 6 | 5 | 4 | 3 |
The angle of rotation in a regular polygon =
Rotational Symmetry: When we rotate an object, if it looks exactly the same, we say that it has rotational symmetry.
Centre of rotation: That fixed point about which the object rotates.
Angles of rotation: The angle by which the object rotates.
Order of Rotational Symmetry:
The number of times an object looks exactly the same in a complete turn
(360°) is called the order of rotational symmetry.
Some objects have only line of symmetry ( like letter E), some objects have only rotational symmetry ( like the letter S), and some have both symmetries ( like the letter H).
Formulas for Reflection:
Reflection in the x-axis , ( X , Y ) → ( X , -Y )
Reflection in the y-axis , ( X , Y ) → ( -X , Y )
NCERT Solutions for Class 7 Maths Chapter 12 Symmetry Exercise 12.1 Page Number: 189-191 Number of Questions: 10 |
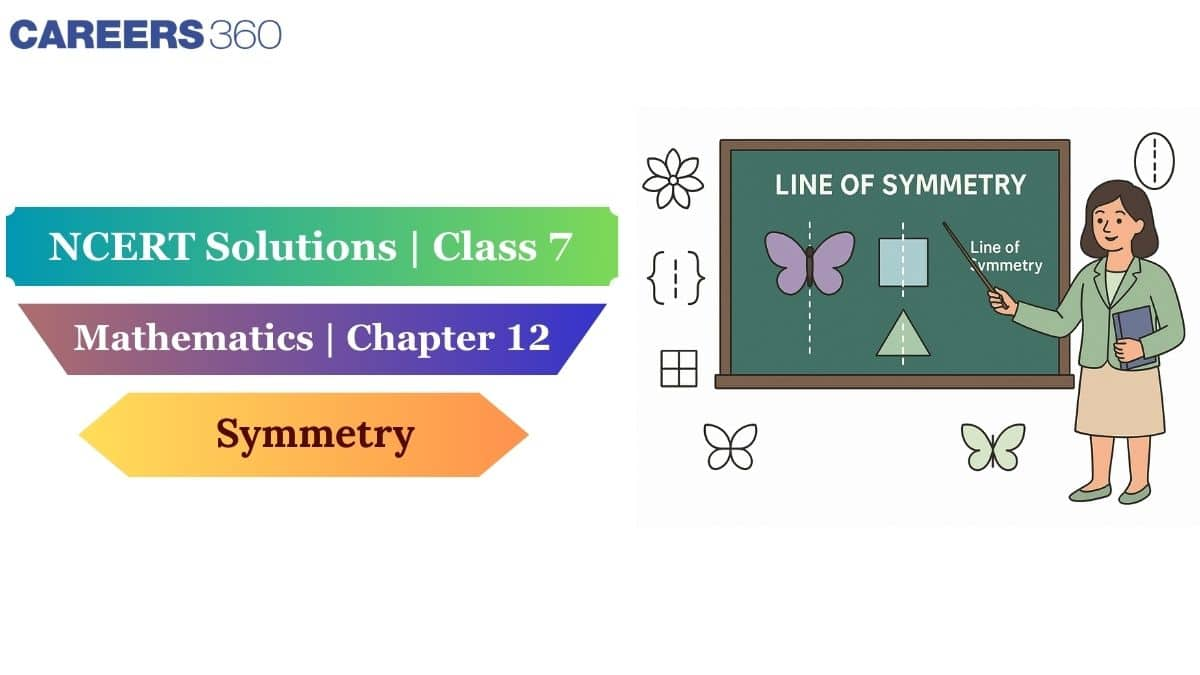
Question: 1 (a) Copy the figures with punched holes and find the axes of symmetry for the following:
Answer:

The axes of symmetry are as shown :

Question: 1 (b) Copy the figures with punched holes and find the axes of symmetry for the following:
Answer:

The axes of symmetry are as shown :

Question: 1 (c) Copy the figures with punched holes and find the axes of symmetry for the following:
Answer:

The axes of symmetry are as shown :

Question: 1 (d) Copy the figures with punched holes and find the axes of symmetry for the following:
Answer:

The axes of symmetry are as shown :

Question: 1 (e) Copy the figures with punched holes and find the axes of symmetry for the following:
Answer:

The axes of symmetry are as shown :

Question: 1 (f) Copy the figures with punched holes and find the axes of symmetry for the following:
Answer:

The axes of symmetry are as shown :

Question: 1 (g) Copy the figures with punched holes and find the axes of symmetry for the following:
Answer:

The axes of symmetry are as shown :

Question: 1 (h) Copy the figures with punched holes and find the axes of symmetry for the following:
Answer:

The axes of symmetry are as shown :

Question: 1 (i) Copy the figures with punched holes and find the axes of symmetry for the following:
Answer:

The axes of symmetry are as shown :

Question: 1 (j) Copy the figures with punched holes and find the axes of symmetry for the following:
Answer:

The axes of symmetry are as shown :

Question: 1 (k) Copy the figures with punched holes and find the axes of symmetry for the following:
Answer:

The axes of symmetry are as shown :

Question: 1 (l) Copy the figures with punched holes and find the axes of symmetry for the following:

Answer:

The axes of symmetry are as shown :

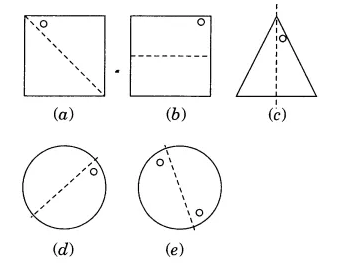
Question: 2 Given the line(s) of symmetry, find the other hole(s):
Answer: The other holes from the symmetry are as shown :
Answer:
The complete figures are as shown :
(a) square (b)triangle (c)rhombus
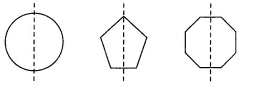
(c) circle (d) pentagon (e) Octagon
Identify multiple lines of symmetry, if any, in each of the following figures:
Answer:
The lines of symmetry of figures are:
(a)There are 3 lines of symmetry. Thus, it has multiple lines of symmetry.
(b) There are 2 lines of symmetry. Thus, it has multiple lines of symmetry.
(c)There are 3 lines of symmetry. Thus, it has multiple lines of symmetry.
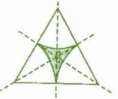
(d)There are 2 lines of symmetry. Thus, it has multiple lines of symmetry.
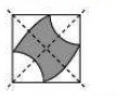
(e)There are 4 lines of symmetry. Thus, it has multiple lines of symmetry.
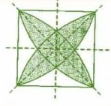
(f)There is 1 line of symmetry.
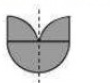
(g)There are 4 lines of symmetry. Thus, it has multiple lines of symmetry.
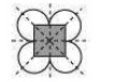
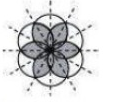
(h)There are 6 lines of symmetry. Thus, it has multiple lines of symmetry.
Question: 5 Copy the figure given here. Take any one diagonal as a line of symmetry and shade a few more squares to make the figure symmetric about a diagonal. Is there more than one way to do that? Will the figure be symmetric about both diagonals?
Answer: The figure with symmetry may be as shown :
The figure with symmetry may be as shown :
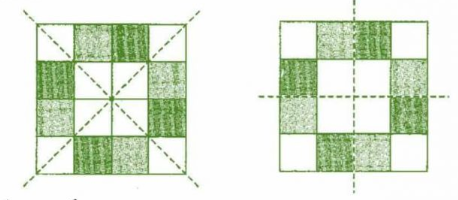
Yes, there is more than one way.
Yes, the figure is symmetric about both the diagonals
Yes, there is more than one way.
Yes, the figure is symmetric about both the diagonals
Question: 6 Copy the diagram and complete each shape to be symmetric about the mirror line(s):
Answer: The complete shapes symmetric about the mirror line(s) are :

Question: 7 State the number of lines of symmetry for the following figures:
(a) An equilateral triangle (b) An isosceles triangle (c) A scalene triangle
(d) A square (e) A rectangle (f) A rhombus
(g) A parallelogram (h) A quadrilateral (i) A regular hexagon
(j) A circle
Answer:
(a) An equilateral triangle
The number of lines of symmetry = 3
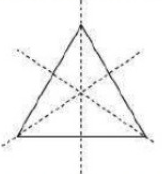
(b) An isosceles triangle
The number of lines of symmetry = 1
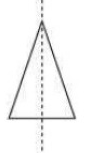
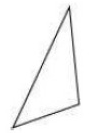
(c) A scalene triangle
The number of lines of symmetry = 0
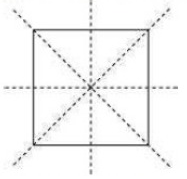
(d) A square
The number of lines of symmetry = 4
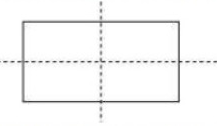
(e) A rectangle
The number of lines of symmetry = 2
(f) A rhombus
The number of lines of symmetry = 2
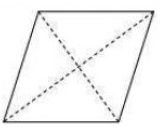
(g) A parallelogram
The number of lines of symmetry = 0

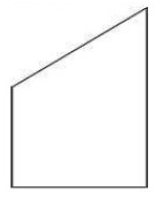
(h) A quadrilateral
The number of lines of symmetry = 0
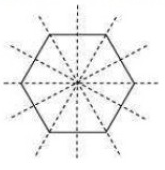
(i) A regular hexagon
The number of lines of symmetry = 6
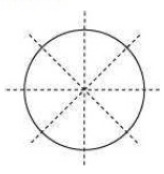
(j) A circle
The number of lines of symmetry = infinite
(a) a vertical mirror
(b) a horizontal mirror
(c) Both horizontal and vertical mirrors
Answer: (a) a vertical mirror: A, H, I, M, O, T, U, V, W, Xand Y
(b) horizontal mirror: B, C, D, E, H, I, O and X
(c) both horizontal and vertical mirrors: H, I, O and X.
Question: 9 Give three examples of shapes with no line of symmetry.
Answer: The three examples of shapes with no line of symmetry are :
1. Quadrilateral
2. Scalene triangle
3. Parallelogram
Question: 10 (a) What other name can you give to the line of symmetry of an isosceles triangle?
Answer: The line of symmetry of an isosceles triangle is median or altitude.
Question: 10 (b) What other name can you give to the line of symmetry of
a circle?
Answer: The other name we can give to the line of symmetry of a circle is the diameter.
NCERT Solutions for Class 7 Maths Chapter 12 Symmetry Exercise 12.2 Page Number: 195 Number of Questions: 2 |
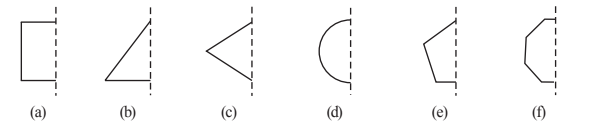
Question: 1 Which of the following figures have rotational symmetry of order more than 1:
Answer: Among the above-given shapes, (a),(b), (d),(e) and (f) have more than one rotational symmetry.
This is because, in these figures, a complete turn, more than 1 number of times, an object looks exactly the same.
Question: 2 Give the order of rotational symmetry for each figure:
Answer: (a) The given figure has a rotational symmetry of about
(b) The given figure has rotational symmetry about
(c) The given figure has rotational symmetry about
(d) The given figure has rotational symmetry about
(e) The given figure has rotational symmetry about
(f) The given figure has rotational symmetry about
(g) The given figure has rotational symmetry about
(h) The given figure has rotational symmetry about
NCERT Solutions for Class 7 Maths Chapter 12 Symmetry Exercise 12.3 Page Number: 196 Number of Questions: 7 |
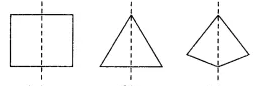
Question: 1 Name any two figures that have both line symmetry and rotational symmetry
Answer: The two figures that have both line symmetry and rotational symmetry are :
(i) Equilateral triangle
(ii) Regular hexagon
Question: 2 (i) Draw, wherever possible, a rough sketch of
a triangle with both line and rotational symmetries of order more than 1.
Answer:
Line of symmetry is shown below :
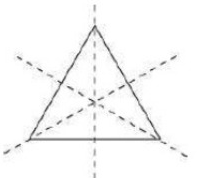
The rotational symmetry is shown below :
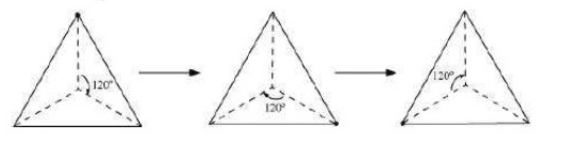
Question: 2 (ii) Draw, wherever possible, a rough sketch of
a triangle with only line symmetry and no rotational symmetry of order more than 1.
Answer: A triangle with only line symmetry and no rotational symmetry of order more than 1 is an isosceles triangle.
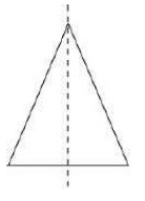
Question: 2 (iii) Draw, wherever possible, a rough sketch of
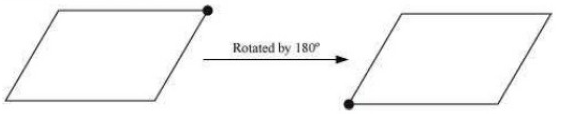
a quadrilateral with a rotational symmetry of order more than 1 but not a line symmetry
Answer: A quadrilateral with a rotational symmetry of order more than 1 but not a line symmetry is a parallelogram.
Question: 2 (iv) Draw, wherever possible, a rough sketch of
a quadrilateral with line symmetry but not a rotational symmetry of order more than 1.
Answer: A quadrilateral with line symmetry but not a rotational symmetry of order more than 1 is a kite.
Question: 3 If a figure has two or more lines of symmetry, should it have rotational symmetry of order more than 1?
Answer: Yes. If a figure has two or more lines of symmetry, then it should have rotational symmetry of order more than 1.
Question: 4 Fill in the blanks:
Answer: The given table is completed as shown:
Question: 5 Name the quadrilaterals which have both line and rotational symmetry of order more than 1.
Answer: The quadrilaterals which have both line and rotational symmetry of order more than 1 are :
1. Rectangle
2. Square
3. Rhombus
Answer: After rotating by
Question: 7 Can we have a rotational symmetry of order more than 1 whose angle of rotation is:
Answer: We can observe that the angle of rotation is the factor of
(i)
(ii)
The angle of rotation in a regular polygon =
A half-turn = Rotation by
A quarter-turn = Rotation by
Reflection in the x-axis , ( X , Y ) → ( X , -Y )
Reflection in the y-axis , ( X , Y ) → ( -X , Y )
The NCERT Subject Wise Solutions for Class 7 is one of the important and essential study materials as it contains step-by-step solutions with conceptual clarity for all the chapters in each subject.

Register for ALLEN Scholarship Test & get up to 90% Scholarship

Get up to 90% Scholarship on Offline NEET/JEE coaching from top Institutes

This ebook serves as a valuable study guide for NEET 2025 exam.

This e-book offers NEET PYQ and serves as an indispensable NEET study material.
As per latest 2024 syllabus. Physics formulas, equations, & laws of class 11 & 12th chapters
As per latest 2024 syllabus. Chemistry formulas, equations, & laws of class 11 & 12th chapters