• School
  • Engineering and Architecture
  • Management and Business Administration
  • Medicine and Allied Sciences
  • Law
  • Animation and Design
  • Media, Mass Communication and Journalism
  • Finance & Accounts
  • Computer Application and IT
  • Pharmacy
  • Hospitality and Tourism
  • Competition
  • Study Abroad
  • Arts, Commerce & Sciences
  • Learn
  • Online Courses and Certifications
NCERT Solutions for Class 7 Maths Chapter 9 Rational Numbers - Download PDF

NCERT Solutions for Class 7 Maths Chapter 9 Rational Numbers - Download PDF

Edited By Ravindra Pindel | Updated on Feb 07, 2024 06:06 PM IST

NCERT Solutions for Class 7 Maths Chapter 9 Rational Numbers: In earlier classes, you have studied whole numbers, natural numbers, integer numbers. In this article, you will get CBSE NCERT rational numbers class 7 solutions. Fractions number is a rational number that contains only positive integers whereas the rational number contains positive and negative integers. All fractions are rational numbers but all rational numbers are not fractions.

This Story also Contains
  1. NCERT Solutions for Maths Chapter 9 Rational Numbers Class 7 - Important Formulae
  2. NCERT Solutions for Class 7 Maths Chapter 9 Rational Numbers - Important Points
  3. NCERT Solutions for Maths Chapter 9 Rational Numbers Class 7
  4. NCERT Solutions for Class 7 Maths Chapter 9 Rational Numbers (Intext Questions and Exercise)
  5. NCERT Solutions for Maths Chapter 9 Rational Numbers Class 7 Topic 9.3
  6. NCERT rational numbers class 7 solutions Topic 9.4
  7. NCERT Solutions for Class 7 Maths Chapter 9 Topic 9.6
  8. NCERT Solutions for Maths Chapter 9 Rational Numbers Class 7 Topic 9.8
  9. NCERT Solutions for Class 7 Chapter 9 Rational Numbers Maths Exercise 9.1
  10. NCERT Solutions for Chapter 9 Maths Class 7 Rational Numbers Topic 9.9.1
  11. NCERT Solutions for Chapter 9 Maths Class 7 Rational Numbers Topic 9.9.2
  12. NCERT Solutions for Class 7th Math Chapter 9 Rational Numbers Topic 9.9.3
  13. NCERT Solutions for Class 7th Math Chapter 9 Rational Numbers Topic 9.9.4
  14. NCERT Solutions for Class 7th Math Chapter 9 Rational Numbers Exercise: 9.2
  15. Rational Numbers Class 7 Maths Chapter 9-Topics

Once you go through NCERT Solutions for Class 7 you will get more clarity of the concepts. You must refer to the NCERT Syllabus for Class 7 Maths for better understanding. There are 14 questions in 2 exercises given in NCERT . In CBSE NCERT solutions for Class 7 Maths chapter 9 rational numbers, you will get all detailed explanations of all these questions including practice question given at end of the very topic. You can get NCERT Solutions by clicking on the above link. Here you will get solutions to two exercises of this chapter.

NCERT Solutions for Maths Chapter 9 Rational Numbers Class 7 - Important Formulae

Rational number = p/q, Where p and q are integers and q ≠ 0.

Numerator and Denominator: In the p/q , p is the numerator and q is the denominator.

Comparison of Rational Numbers:

p/q < a/b If pb < aq

p/q > a/b If pb > aq

Operations on Rational Number:

  • Addition of rational numbers : (p/q) + (a/b) = ((p×b) + (a×q))/(q×b)

  • Subtraction of rational numbers: (p/q) - (a/b) = ((p×b) - (a×q))/(q×b)

  • Multiplication of rational numbers: p/q) × (a/b) = (p×a)/(q×b)

  • Division of rational numbers: (p/q) ÷ (a/b) = (p×b)/(q×a)

Reciprocal of p/q = q/p

(Rational number)(Reciprocal) = 1

NCERT Solutions for Class 7 Maths Chapter 9 Rational Numbers - Important Points

A rational can be expressed in the form of p/q , Where p and q are integers and q ≠ 0.

Numerator and Denominator: In the p/q , p is the numerator and q is the denominator.

We obtain another equivalent rational number by multiplying the numerator and denominator with the same nonzero integer.

+ sign and positive integer: a position to the right of 0.

- sign and negative integer: a position to the left of 0.

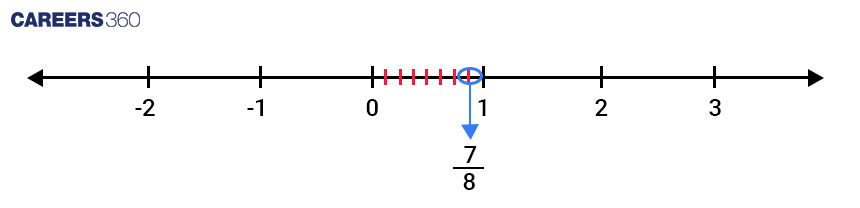
1692068296035

Rational Numbers in Standard Form:

Its denominator is a positive integer.

The numerator and denominator have no common factor other than 1.

Examples: 3/5 , -5/8 , 2/7, etc.

Comparison of Rational Numbers:

p/q < a/b If pb < aq

p/q > a/b If pb > aq

Operations on Rational Number:

  • Addition of rational numbers : (p/q) + (a/b) = ((p×b) + (a×q))/(q×b)

  • Subtraction of rational numbers: (p/q) - (a/b) = ((p×b) - (a×q))/(q×b)

  • Multiplication of rational numbers: p/q) × (a/b) = (p×a)/(q×b)

  • Division of rational numbers: (p/q) ÷ (a/b) = (p×b)/(q×a)

Reciprocal of a rational number:

Reciprocal of p/q = q/p

Product of rational numbers with its reciprocal is always 1.

Free download NCERT Solutions for Class 7 Maths Chapter 9 Rational Numbers PDF for CBSE Exam.

NCERT Solutions for Maths Chapter 9 Rational Numbers Class 7

Download PDF

NCERT Solutions for Class 7 Maths Chapter 9 Rational Numbers (Intext Questions and Exercise)

NCERT Solutions for Maths Chapter 9 Rational Numbers Class 7 Topic 9.3

Question:1 Is the number \frac{2}{-3} rational? Think about it.

Answer: Yes , \frac{2}{-3} is a rational number because it is written in the form: \frac{p}{q} , where p = 2\ and\ q = -3\neq 0 .

Question:2 List ten rational numbers.

Answer: Any ten rational numbers are:

1, \frac{2}{3}, -\frac{1}{3}, -5, 0, 356, -\frac{39}{25}, -36, 1999, \frac{-1}{-2}


Question: Fill in the boxes:

(i) \frac{5}{4}=\frac{\square }{16}=\frac{25}{\square }=\frac{-15}{\square } (ii) \frac{-3}{7}=\frac{\square }{14}=\frac{9}{\square }=\frac{-6}{\square }

Answer:

(i) \frac{5}{4}=\frac{\square }{16}=\frac{25}{\square }=\frac{-15}{\square }

\frac{5}{4} can be written as:

\Rightarrow \frac{5}{4} = \frac{5\times4}{4\times4} = \frac{20}{16}

\Rightarrow \frac{5}{4} = \frac{5\times5}{4\times5} = \frac{25}{20}

\Rightarrow \frac{5}{4} = \frac{5\times-3}{4\times-3} = \frac{-15}{-12}

Hence, we have

\frac{5}{4}=\frac{20 }{16}=\frac{25}{20 }=\frac{-15}{-12 }

(ii) \frac{-3}{7}=\frac{\square }{14}=\frac{9}{\square }=\frac{-6}{\square }

\frac{-3}{7} can be written as:

\Rightarrow \frac{-3}{7} = \frac{-3\times2}{7\times2} = \frac{-6}{14}

\Rightarrow \frac{-3}{7} = \frac{-3\times-3}{7\times-3} = \frac{9}{-21}

\Rightarrow \frac{-3}{7} = \frac{-3\times2}{7\times2} = \frac{-6}{14}

Hence, we have

\frac{-3}{7}=\frac{-6 }{14}=\frac{9}{-21}=\frac{-6}{14 }

NCERT rational numbers class 7 solutions Topic 9.4

Question:1 Is 5 a positive rational number?

Answer: Yes, 5 can be written as a positive rational number \frac{5}{1} , where 5 and 1 are both positive integers and denominator not equal to zero.

Question:2 List five more positive rational numbers.

Answer: Five more positive rational numbers are:

4,\ \frac{1}{3},\ 56,\ \frac{99}{98}, 5

Question:1 Is – 8 a negative rational number?

Answer: Yes, -8 is a negative rational number because it can be written as \frac{-8}{1} , where the numerator is negative integer and denominator is a positive integer.

Question:2 List five more negative rational numbers.

Answer: Five more negative rational numbers are:

-1,\ -99,\ -\frac{2}{3},\ -\frac{5}{7}, \ -27

Question: Which of these are negative rational numbers?

(i) \frac{-2}{3} (ii) \frac{5}{7} (iii) \frac{3}{-5} (iv) 0 (v) \frac{6}{11} (vi) \frac{-2}{-9}

Answer: (i) \frac{-2}{3} here, the numerator is -2 which is negative and the denominator is 3 which is positive.

Hence, the fraction is negative.

(ii) \frac{5}{7} here, the numerator is 5 which is positive and the denominator is 7 which is also positive.

Hence, the fraction is positive.

(iii) \frac{3}{-5} here, the numerator is 3 which is positive and the denominator is -5 which is negative.

Hence, the fraction is negative.

(iv) 0 zero is neither a positive nor a negative number.

(v) \frac{6}{11} here, the numerator is 6 which is positive and the denominator is 11 which is also positive.

Hence, the fraction is positive.

(vi) \frac{-2}{-9} here, the numerator is -2 which is negative and the denominator is -9 which is also a negative integer.

Hence, the fraction is overall a positive fraction.

NCERT Solutions for Class 7 Maths Chapter 9 Topic 9.6

Question: Find the standard form of

(i) \frac{-18}{45} (ii) \frac{-12}{18}

Answer: (i) Given fraction \frac{-18}{45} .

We can make it in the standard form :

\frac{-18}{45} = \frac{-6\times3}{15\times3} = \frac{-2\times3\times3}{5\times3\times3} = \frac{-2}{5}

(i) Given fraction \frac{-12}{18} .

We can make it in the standard form :

\frac{-12}{18} = \frac{-6\times2}{9\times2} = \frac{-2\times3\times2}{3\times3\times2} = \frac{-2}{3}

NCERT Solutions for Maths Chapter 9 Rational Numbers Class 7 Topic 9.8

Question: Find five rational numbers between

\frac{-5}{7} and \frac{-3}{8}

Answer: LCM of 7 and 8 is 56.

Hence we can write given fractions as:

\frac{-5}{7} = \frac{-5\times8}{7\times8} = \frac{-40}{56} and \frac{-3}{8} = \frac{-3\times7}{8\times7} = \frac{-21}{56}

Therefore, we can find five rational numbers between \frac{-5}{7} and \frac{-3}{8} .

\frac{-39}{56},\ \frac{-38}{56},\ \frac{-37}{56},\ \frac{-36}{56},\ and\ \frac{-35}{56}

NCERT Solutions for Class 7 Chapter 9 Rational Numbers Maths Exercise 9.1

Question: 1(i) List five rational numbers between:

–1 and 0

Answer: To find five rational numbers between -1\ and\ 0 we will convert each rational numbers as a denominator 5+1 =6 , we have

-1 = \frac{-1\times6}{6} = \frac{-6}{6}\ and\ \frac{0\times6}{6} = \frac{0}{6}

So, we have five rational numbers between \frac{-6}{6}\ and\ \frac{0}{6}

\frac{-6}{6}<\frac{-5}{6}<\frac{-4}{6}<\frac{-3}{6}<\frac{-2}{6}<\frac{-1}{6}<\frac{0}{6}

Hence, the five rational numbers between -1 and 0 are:

\frac{-5}{6},\frac{-4}{6},\frac{-3}{6},\frac{-2}{6}\ and\ \frac{-1}{6}.

Question: 1(ii) List five rational numbers between:

–2 and –1

Answer: To find five rational numbers between -2\ and\ -1 we will convert each rational numbers as a denominator 5+1 =6 , we have

-2 = \frac{-2\times6}{6} = \frac{-12}{6}\ and\ \frac{-1\times6}{6} = \frac{-6}{6}

So, we have five rational numbers between \frac{-12}{6}\ and\ \frac{-6}{6}

\frac{-12}{6}<\frac{-11}{6}<\frac{-10}{6}<\frac{-9}{6}<\frac{-8}{6}<\frac{-7}{6}<\frac{-6}{6}

Hence, the required rational numbers are

\frac{-11}{6},\frac{-5}{3},\frac{-3}{2},\frac{-4}{3}\ and\ \frac{-7}{6}.

Question: 1(iii) List five rational numbers between:

\frac{-4}{5}and \frac{-2}{3}

Answer: To find five rational numbers between \frac{-4}{5}and \frac{-2}{3} we will convert each rational numbers with the denominator as 5\times3 =15 , we have \left ( \because LCM\ of\ 5\ and\ 3 = 15 \right )

\frac{-4}{5} = \frac{-4\times3}{5\times3} = \frac{-12}{15}\ and\ \frac{-2}{3} = \frac{-2\times5}{3\times5} = \frac{-10}{15}

Since there is only one integer i.e., -11 between -12 and -10, we have to find equivalent rational numbers.

\frac{-12}{15} = \frac{-12\times3}{15\times3} = \frac{-36}{45}\ and\ \frac{-10}{15} = \frac{-10\times3}{15\times3} = \frac{-30}{45}

Now, we have five rational numbers possible:

\therefore \frac{-36}{45}<\frac{-35}{45}<\frac{-34}{45}<\frac{-33}{45}<\frac{-32}{45}<\frac{-31}{45}<\frac{-30}{45}

Hence, the required rational numbers are

\frac{-7}{9},\frac{-34}{45},\frac{-11}{15},\frac{-32}{45}\ and\ \frac{-31}{45}.

Question: 1(iv) List five rational numbers between:

-\frac{1}{2} and \frac{2}{3}

Answer: To find five rational numbers between -\frac{1}{2} and \frac{2}{3} we will convert each rational numbers in their equivalent numbers, we have

Making denominator as LCM(2,3)=6

that is

\frac{-3}{6}\ and\ \frac{4}{6}

Now, we have five rational numbers possible:

\therefore \frac{-3}{6}<\frac{-2}{6}<\frac{-1}{6}<\frac{0}{6}<\frac{2}{6}<\frac{3}{6}<\frac{4}{6}

Hence, the required rational numbers are

\frac{-1}{3},\frac{-1}{6},0,\frac{1}{3}\ and\ \frac{1}{2}.

Question: 2(i) Write four more rational numbers in each of the following patterns:

\frac{-3}{5}, \frac{-6}{10}, \frac{-9}{15}, \frac{-12}{20}

Answer: We have the pattern:

\frac{-3}{5} = \frac{-3\times1}{5\times1} \ \frac{-6}{10} = \frac{-3\times2}{5\times2} \frac{-9}{15} = \frac{-3\times3}{5\times3} \frac{-12}{20} = \frac{-3\times4}{5\times4}

Now, following the same pattern, we have

\frac{-3\times5}{5\times5} = \frac{-15}{25} \frac{-3\times6}{5\times6} = \frac{-18}{30} \frac{-3\times7}{5\times7} = \frac{-21}{35} \frac{-3\times8}{5\times8} = \frac{-24}{40}

Hence, the required rational numbers are:

\frac{-15}{25},\ \frac{-18}{30},\ \frac{-21}{35},and\ \frac{-24}{40}.

Question: 2(ii) Write four more rational numbers in each of the following patterns:

\frac{-1}{4},\frac{-2}{8}, \frac{-3}{12}....

Answer: We have the pattern:

\frac{-1}{4} = \frac{-1\times1}{4\times1} \frac{-2}{8} = \frac{-1\times2}{4\times2} \frac{-3}{12} = \frac{-1\times3}{4\times3}

Now, following the same pattern, we have

\frac{-1\times4}{4\times4} = \frac{-4}{16} \frac{-1\times5}{4\times5} = \frac{-5}{20} \frac{-1\times6}{4\times6} = \frac{-6}{24} \frac{-1\times7}{4\times7} = \frac{-7}{28}

Hence, the required rational numbers are:

\frac{-4}{16},\ \frac{-5}{20},\ \frac{-6}{24},and\ \frac{-7}{28}.

Question: 2(iii) Write four more rational numbers in each of the following patterns:

\frac{-1}{6}, \frac{-2}{12}, \frac{-3}{18}, \frac{-4}{24}....

Answer: We have the pattern:

\frac{-1}{6} = \frac{-1\times1}{6\times1} \frac{-2}{12} = \frac{-1\times2}{6\times2} \frac{-3}{18} = \frac{-1\times3}{6\times3} \frac{-4}{24} = \frac{-1\times4}{6\times4}

Now, following the same pattern, we have

\frac{-1\times5}{6\times5} = \frac{-5}{30} \frac{-1\times6}{6\times6} = \frac{-6}{36} \frac{-1\times7}{6\times7} = \frac{-7}{42} \frac{-1\times8}{6\times8} = \frac{-8}{48}

Hence, the required rational numbers are:

\frac{-5}{30},\ \frac{-6}{36},\ \frac{-7}{42},and\ \frac{-8}{48}.

Question: 2(iv) Write four more rational numbers in each of the following patterns:

\frac{-2}{3}, \frac{2}{-3},\frac{4}{-6}, \frac{6}{-9}....

Answer: We have the pattern:

\frac{-2}{3} = \frac{-2\times1}{3\times1} \frac{2}{-3} =\frac{2}{-3} = \frac{2\times1}{-3\times1} \frac{4}{-6} = \frac{-2\times2}{3\times2} \frac{-6}{9} = \frac{-2\times3}{3\times3}

Now, following the same pattern, we have

\frac{-2\times4}{3\times4} = \frac{-8}{12}\ or\ \frac{8}{-12} \frac{-2\times5}{3\times5} = \frac{-10}{15}\ or\ \frac{10}{-15}

\frac{-2\times6}{3\times6} = \frac{-12}{18}\ or\ \frac{12}{-18} \frac{-2\times7}{3\times7} = \frac{-14}{21}\ or\ \frac{14}{-21}

Hence, the required rational numbers are:

\frac{8}{-12},\ \frac{10}{-15},\ \frac{12}{-18},and\ \frac{14}{-21}.

Question: 3(i) Give four rational numbers equivalent to:

\frac{-2}{7}

Answer: \frac{-2}{7} can be written as:

\frac{-2}{7} = \frac{-2\times2}{7\times2} = \frac{-4}{14} \frac{-2}{7} = \frac{-2\times3}{7\times3} = \frac{-6}{21}

\frac{-2}{7} = \frac{-2\times4}{7\times4} = \frac{-8}{28} \frac{-2}{7} = \frac{-2\times5}{7\times5} = \frac{-10}{35}

Hence, the required equivalent rational numbers are

\frac{-4}{14},\frac{-6}{21}, \frac{-8}{28},\ and\ \frac{-10}{35} .

Question: 3(ii) Give four rational numbers equivalent to:

\frac{5}{-3}

Answer: \frac{5}{-3} can be written as:

\frac{5}{-3} = \frac{5\times2}{-3\times2} = \frac{10}{-6} \frac{5}{-3} = \frac{5\times3}{-3\times3} = \frac{15}{-9}

\frac{5}{-3} = \frac{5\times4}{-3\times4} = \frac{20}{-12} \frac{5}{-3} = \frac{5\times5}{-3\times5} = \frac{25}{-15}

Hence, the required equivalent rational numbers are

\frac{10}{-6},\frac{15}{-9}, \frac{20}{-12},\ and\ \frac{25}{-20} .

Question: 3(iii) Give four rational numbers equivalent to:

\frac{4}{9}

Answer: \frac{4}{9} can be written as:

\frac{4}{9} = \frac{4\times2}{9\times2} = \frac{8}{18} \frac{4}{9} = \frac{4\times3}{9\times3} = \frac{12}{27}

\frac{4}{9} = \frac{4\times4}{9\times4} = \frac{16}{36} \frac{4}{9} = \frac{4\times5}{9\times5} = \frac{20}{45}

Hence, the required equivalent rational numbers are

\frac{8}{18},\frac{12}{27}, \frac{16}{36},\ and\ \frac{20}{45} .

Question: 4(i) Draw the number line and represent the following rational numbers on it:

\frac{3}{4}

Answer: Representation of \frac{3}{4} on the number line,

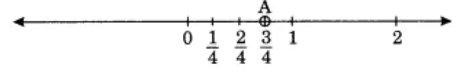
1643867890014

Question: 4(ii) Draw the number line and represent the following rational numbers on it:

\frac{-5}{8}

Answer: Representation of \frac{-5}{8} on the number line,

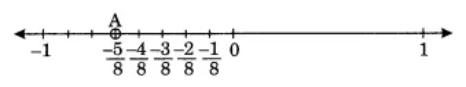
1643867932616

Question: 4(iii) Draw the number line and represent the following rational numbers on it:

\frac{-7}{4}

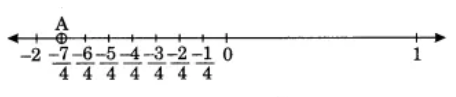
Answer: Representation of \frac{-7}{4} on the number line, 1643867960214

Question: 4(iv) Draw the number line and represent the following rational numbers on it:

\frac{7}{8}

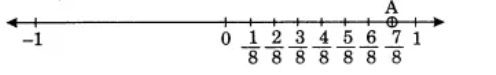
Answer: Representation of \frac{7}{8} on the number line, 1643867985343

Question: 5 The points P, Q, R, S, T, U, A and B on the number line are such that, TR = RS = SU and AP = PQ = QB. Name the rational numbers represented by P, Q, R, and S.

1643868029468

Answer: Given TR = RS = SU and AP = PQ = QB then, we have

There are two rational numbers between A and B i.e., P and Q which are at equal distances hence,

The rational numbers represented by P and Q are:

P =2+ \frac{1}{3} = \frac{7}{3}\ and\ Q = 2+\frac{2}{3} = \frac{8}{3}

Also, there are two rational numbers between U and T i.e., S and R which are at equal distances hence,

The rational numbers represented by S and R are:

S =-2+ \frac{1}{3} = \frac{-5}{3}\ and\ R = -2+\frac{2}{3} = \frac{-4}{3}

Question: 6 Which of the following pairs represent the same rational number?

(i) \frac{-7}{21}\ and\ \frac{3}{9} (ii) \frac{-16}{20} \ and\ \frac{20}{-25}

(iii) \frac{-2}{-3} \ and\ \frac{2}{3} (iv) \frac{-3}{5}\ and\ \frac{-12}{20}

(v) \frac{8}{-5}\ and\ \frac{-24}{15} (vi) \frac{1}{3}\ and\ \frac{-1}{9}

(vii) \frac{-5}{-9}\ and\ \frac{5}{-9}

Answer: To compare we multiply both numbers with denominators:

(i) We have \frac{-7}{21}\ and\ \frac{3}{9}

\Rightarrow \frac{-7\times9}{21\times9} = \frac{-63}{189}

\Rightarrow \frac{3\times21}{9\times21} = \frac{63}{189}

\Rightarrow \frac{-63}{189} \neq \frac{63}{189}

Here, they are equal but are in opposite signs hence, \frac{-7}{21}\ and\ \frac{3}{9} do not represent the same rational numbers.

(ii) We have \frac{-16}{20} \ and\ \frac{20}{-25}

\Rightarrow \frac{-16\times-25}{20\times-25} = \frac{400}{-500}

\Rightarrow \frac{20\times20}{-25\times20} = \frac{400}{-500}

\Rightarrow \frac{400}{-500} = \frac{400}{-500}

So, they represent the same rational number.

(iii) We have \frac{-2}{-3} \ and\ \frac{2}{3}

Here, Both represents the same number as these minus signs on both numerator and denominator of \frac{-2}{-3} = \frac{2}{3} will cancel out and gives the positive value.

(iv) We have \frac{-3}{5}\ and\ \frac{-12}{20}

\Rightarrow \frac{-3\times20}{5\times20} = \frac{-60}{100}

\Rightarrow \frac{-12\times5}{20\times5} = \frac{-60}{100}

\Rightarrow \frac{-60}{100} = \frac{-60}{100}

So, they represent the same rational number.

(v) We have \frac{8}{-5}\ and\ \frac{-24}{15}

\Rightarrow \frac{8\times15}{-5\times15} = \frac{120}{-75}

\Rightarrow \frac{-24\times-5}{15\times-5} = \frac{120}{-75}

\Rightarrow \frac{120}{-75} = \frac{120}{-75}

So, they represent the same rational number.

(vi) We have \frac{1}{3}\ and\ \frac{-1}{9}

\Rightarrow \frac{1\times9}{3\times9} = \frac{9}{27}

\Rightarrow \frac{-1\times3}{9\times3} = \frac{-3}{27}

\Rightarrow \frac{9}{27} \neq \frac{-3}{27}

So, They do not represent the same rational number.

(vii) We have \frac{-5}{-9}\ and\ \frac{5}{-9}

Here, the denominators of both are the same but -5 \neq 5 .

So, \frac{-5}{-9}\ and\ \frac{5}{-9} do not represent the same rational numbers.

Question: 7 Rewrite the following rational numbers in the simplest form:

(i) \frac{-8}{6} (ii) \frac{22}{25} (iii) \frac{-44}{72} (iv) \frac{-8}{10}

Answer: (i) \frac{-8}{6} can be written as:

\Rightarrow \frac{-8}{6} = \frac{-8/2}{6/2}= \frac{-4}{3} \left [ HCF\ of\ 8\ and\ 6\ is\ 2 \right ]

(ii) \frac{25}{45} can be written in the simplest form:

\Rightarrow \frac{25}{45} = \frac{25/5}{45/5}= \frac{5}{9} \left [ HCF\ of\ 25\ and\ 45\ is\ 5 \right ]

(iii) \frac{-44}{72} can be written as in simplest form:

\Rightarrow \frac{-44}{72} = \frac{-44/4}{72/4}= \frac{-11}{18} \left [ HCF\ of\ 44\ and\ 72\ is\ 4 \right ]

Question: 8 Fill in the boxes with the correct symbol out of >, <, and =.

(i) \frac{-5}{7} \square \frac{2}{3} (ii) \frac{-4}{5} \square \frac{-5}{7} (iii) \frac{-7}{8} \square \frac{14}{-16}

(iv) \frac{-8}{5} \square \frac{-7}{4} (v) \frac{1}{-3} \square \frac{-1}{4} (vi) \frac{5}{-11} \square \frac{-5}{11}

(vii) 0 \square \frac{-7}{6}

Answer: (i) \frac{-5}{7} \square \frac{2}{3}

\Rightarrow \frac{-5\times3}{7\times 3}\square \frac{2\times7}{3\times7}

\Rightarrow \frac{-15}{21} < \frac{14}{21}

Hence, \frac{-5}{7} < \frac{2}{3}

(ii) \frac{-4}{5} \square \frac{-5}{7}

\Rightarrow \frac{-4\times7}{5\times 7}\square \frac{-5\times5}{7\times5}

\Rightarrow \frac{-28}{35} < \frac{-25}{35}

Hence, \frac{-4}{5} < \frac{-5}{7}

(iii) \frac{-7}{8} \square \frac{14}{-16}

\Rightarrow \frac{-7\times-16}{8\times -16}\square \frac{14\times8}{-16\times8}

\Rightarrow \frac{112}{-128} = \frac{112}{-128}

Hence, \frac{-7}{8} = \frac{14}{-16}

(iv) \frac{-8}{5} \square \frac{-7}{4}

\Rightarrow \frac{-8\times4}{5\times 4}\square \frac{-7\times5}{4\times5}

\Rightarrow \frac{-32}{20} > \frac{-35}{20}

Hence, \frac{-8}{5} > \frac{-7}{4}

(v) \frac{1}{-3} \square \frac{-1}{4}

\Rightarrow \frac{1\times4}{-3\times 4}\square \frac{-1\times-3}{4\times-3}

\Rightarrow \frac{4}{-12} < \frac{3}{-12}

Hence, \frac{1}{-3} < \frac{1}{-4}

(vi) \frac{5}{-11} \square \frac{-5}{11}

\Rightarrow \frac{5\times11}{-11\times 11}\square \frac{-5\times-11}{11\times-11}

\Rightarrow \frac{55}{-121} = \frac{55}{-121}

Hence, \frac{5}{-11} = \frac{-5}{11}

(vii) 0 \square \frac{-7}{6}

Zero is always greater than every negative number.

Therefore, 0 > \frac{-7}{6}

Question: 9 Which is greater in each of the following:

(i) \frac{2}{3}, \frac{5}{2} (ii) \frac{-5}{6}, \frac{-4}{3}

(iii) \frac{-3}{4}, \frac{2}{-3} (iv) \frac{-1}{4}, \frac{1}{4}

(v) -3\frac{2}{7}, -3\frac{4}{5}

Answer: (i) \frac{2}{3}, \frac{5}{2}

\Rightarrow \frac{2\times2}{3\times2}, \frac{5\times3}{2\times3}

\Rightarrow \frac{4}{6}, \frac{15}{6}

Since, \frac{15}{6}> \frac{4}{6}

So, \frac{5}{2}> \frac{2}{3}.

(ii) \frac{-5}{6}, \frac{-4}{3}

\Rightarrow \frac{-5\times3}{6\times3}, \frac{-4\times6}{3\times6}

\Rightarrow \frac{-15}{18}, \frac{-24}{18}

Since, \frac{-15}{18}> \frac{-24}{18}

So, \frac{-5}{6}> \frac{-4}{3}.

(iii) \frac{-3}{4}, \frac{2}{-3}

\Rightarrow \frac{-3\times-3}{4\times-3}, \frac{2\times4}{-3\times4}

\Rightarrow \frac{9}{-12}, \frac{8}{-12}

Since, \frac{8}{-12}> \frac{9}{-12}

So, \frac{2}{-3}> \frac{-3}{4}

(iv) \frac{-1}{4}, \frac{1}{4}

\Rightarrow \frac{1}{4}> \frac{-1}{4}

As each positive number is greater than its negative.

(v) -3\frac{2}{7}, -3\frac{4}{5}

\Rightarrow \frac{-23}{7}, \frac{-19}{5} =\frac{-23\times5}{7\times5}, \frac{-19\times7}{5\times7}

\Rightarrow \frac{-115}{35} > \frac{-133}{35}

So, -3\frac{2}{7}> -3\frac{4}{5}

Question: 10(i) Write the following rational numbers in ascending order:

\frac{-3}{5}, \frac{-2}{5}, \frac{-1}{5}

Answer: (i) Here the denominator value is the same.

Therefore, -3<-2<-1

Hence, the required ascending order is

\frac{-3}{5}<\frac{-2}{5}<\frac{-1}{5}

Question: 10(ii) Write the following rational numbers in ascending order:

\frac{-1}{3},\frac{2}{9}, \frac{-4}{3}

Answer: Given \frac{-1}{3},\frac{2}{9}, \frac{-4}{3}

LCM of 3,9\ and\ 3 = 9 .

Therefore, we have

\frac{1\times3}{3\times3}, \frac{-2\times1}{9\times1}, \frac{-4\times3}{3\times3}

\Rightarrow \frac{3}{9}, \frac{-2}{9}, \frac{-12}{9}

Since \frac{-12}{9}<\frac{-2}{9}<\frac{3}{9}

Hence, the required ascending order is

\frac{-4}{3}<\frac{-2}{9}<\frac{1}{3}

Question: 10(iii) Write the following rational numbers in ascending order:

\frac{-3}{7},\frac{-3}{2}, \frac{-3}{4}

Answer: Given \frac{-1}{3},\frac{2}{9}, \frac{-4}{3}

LCM of 7,2\ and\ 4 =28 .

Therefore, we have

\frac{-3\times4}{7\times4}, \frac{-3\times14}{2\times14}, \frac{-3\times7}{4\times7}

\Rightarrow \frac{-12}{28}, \frac{-42}{28}, \frac{-21}{28}

Since \frac{-42}{28}<\frac{-21}{28}<\frac{-12}{28}

Hence, the required ascending order is

\frac{-3}{2}<\frac{-3}{4}<\frac{-3}{7}

NCERT Solutions for Chapter 9 Maths Class 7 Rational Numbers Topic 9.9.1

Question: Find:

\frac{-13}{7}+\frac{6}{7},\frac{19}{5}+\left ( \frac{-7}{5} \right )

Answer: For the given sum: \frac{-13}{7}+\frac{6}{7}

Here the denominator value is same that is 7 hence we can sum the numerator as:

\frac{-13}{7}+\frac{6}{7}= \frac{-13+6}{7} = \frac{-7}{7} = -1

For the given sum: \frac{19}{5}+\left ( \frac{-7}{5} \right )

Here also the denominator value is the same and is equal to 5 hence we can write it as:

\frac{19}{5}+\left ( \frac{-7}{5} \right ) = \frac{19-7}{5} = \frac{12}{5}

Question:(i) Find:

\frac{-3}{7}+\frac{2}{3}

Answer: Given sum: \frac{-3}{7}+\frac{2}{3}

Taking LCM of 7 and 3 we get; 21

Hence we can write the sum as:

\Rightarrow \frac{-3\times3}{7\times3}+\frac{2\times7}{3\times7}

\Rightarrow \frac{-9}{21}+\frac{14}{21} = \frac{5}{21}

Question:(ii) Find:

\frac{-5}{6}+\frac{-3}{11}

Answer: Given sum: \frac{-5}{6}+\frac{-3}{11}

Taking LCM of 6 and 11 we get; 66

Hence we can write the sum as:

\Rightarrow \frac{-5\times11}{6\times11}+\frac{-3\times6}{11\times6}

\Rightarrow \frac{-55}{66}+\frac{-18}{66} = \frac{-73}{66}

NCERT Solutions for Chapter 9 Maths Class 7 Rational Numbers Topic 9.9.2

Question:1 What will be the additive inverse of

-3/9, -9/11, 5/7

Answer: The additive inverse of

\frac{-3}{9} \ is \ \frac{3}{9}

The additive inverse of

\frac{-9}{11} \ is \ \frac{9}{11}

The additive inverse of

\frac{5}{7} \ is \ \frac{-5}{7}

Question:2 Find (i)\frac{7}{9}-\frac{2}{5} \ \ \ \ \ (ii) 2 \frac{1}{5}-\frac{(-1)}{3}

Answer:

(i)\frac{7}{9}-\frac{2}{5} =\frac{7 \times 5-9 \times2}{45}=\frac{17}{45} \\ (ii) 2 \frac{1}{5}-\frac{(-1)}{3}=\frac{11}{5}+\frac{1}{3}=\frac{11 \times 3+ 1 \times 5}{15}=\frac{38}{15}

NCERT Solutions for Class 7th Math Chapter 9 Rational Numbers Topic 9.9.3

Question: What will be

(i) \frac{-3}{5}\times 7 (ii) \frac{-6}{5}\times (-2)

Answer: (i) \frac{-3}{5}\times 7

We can write the product as:

\frac{-3}{5}\times 7 = \frac{-3}{5}\times\frac{7}{1} = \frac{-21}{5}

(i) \frac{-6}{5}\times (-2)

We can write the product as:

\frac{-6}{5}\times (-2) = \frac{-6}{5}\times\frac{-2}{1} = \frac{12}{5}

Question:(i) Find:

\frac{-3}{4}\times \frac{1}{7}

Answer: Given product:

\frac{-3}{4}\times \frac{1}{7} = \frac{-3\times1}{4\times7} = \frac{-3}{28}

Question:(ii) Find:

\frac{2}{3}\times \frac{-5}{9}

Answer: Given product: \frac{2}{3}\times \frac{-5}{9}

\Rightarrow \frac{2}{3}\times \frac{-5}{9} = \frac{2\times-5}{3\times9} = \frac{-10}{27}

NCERT Solutions for Class 7th Math Chapter 9 Rational Numbers Topic 9.9.4

Question: What will be the reciprocal of

\frac{-6}{11} and \frac{-8}{5}?

Answer: The reciprocal of \frac{-6}{11} will be:

1\div \frac{-6}{11} = \frac{1}{\frac{-6}{11}} =\frac{11}{-6}

The reciprocal of \frac{-8}{5} will be:

1\div \frac{-8}{5} = \frac{1}{\frac{-8}{5}} =\frac{5}{-8}

NCERT Solutions for Class 7th Math Chapter 9 Rational Numbers Exercise: 9.2

Question: 1(i) Find the sum:

\frac{5}{4}+\left (\frac{-11}{4} \right )

Answer: Given sum: \frac{5}{4}+\left (\frac{-11}{4} \right )

Here the denominator is the same which is 4.

\frac{5}{4}+\left (\frac{-11}{4} \right ) = \frac{5-11}{4} = \frac{-6}{4} = \frac{-3\times2}{2\times2} = \frac{-3}{2}

Question: 1(ii) Find the sum:

\frac{5}{3}+\frac{3}{5}

Answer: Given sum: \frac{5}{3}+\frac{3}{5}

Here the LCM of 3 and 5 is 15.

Hence, we can write the sum as:

\Rightarrow \frac{5}{3}+\frac{3}{5} = \frac{5\times5}{3\times5}+\frac{3\times3}{5\times3}

\Rightarrow \frac{25}{15}+\frac{9}{15} = \frac{34}{15}

Question: 1(iii) Find the sum:

\frac{-9}{10}+\frac{22}{15}

Answer: Given sum: \frac{-9}{10}+\frac{22}{15}

Taking the LCM of 10 and 15, we have 30

\Rightarrow \frac{-9\times3}{10\times3}+\frac{22\times2}{15\times2}

\Rightarrow \frac{-27}{30}+\frac{44}{30} = \frac{-27+44}{30} = \frac{17}{30}


Question: 1(iv) Find the sum:

\frac{-3}{-11}+\frac{5}{9}

Answer: Given sum: \frac{-3}{-11}+\frac{5}{9}

Taking LCM of 11 and 9 we have,

\Rightarrow \frac{-3\times9}{-11\times9}+\frac{5\times11}{9\times11}

\Rightarrow \frac{-27}{-99}+\frac{55}{99}=\frac{27+55}{99} = \frac{82}{99}

Question: 1(v) Find the sum :

\frac{-8}{19}+\frac{(-2)}{57}

Answer: Given sum: \frac{-8}{19}+\frac{(-2)}{57}

Taking LCM of 19 and 57, we have 57

We can write the sum as:

\frac{-8\times3}{57}+\frac{(-2)}{57} = \frac{-24-2}{57} = \frac{-26}{57}

Question: 1(vi) Find the sum:

\frac{-2}{3}+0

Answer: Given sum: \frac{-2}{3}+0

Adding any number to zero we get, the number itself

Hence, \frac{-2}{3}+0 = \frac{-2}{3}

Question: 1(vii) Find the sum:

-2\frac{1}{3}+4\frac{3}{5}

Answer: Given the sum: -2\frac{1}{3}+4\frac{3}{5}

Taking the LCM of 3 and 5 we have: 15

\Rightarrow \frac{-7\times5}{3\times5}+\frac{23\times3}{5\times3} = \frac{-35}{15}+\frac{69}{15} = \frac{34}{15}

Question: 2(i) Find

\frac{7}{24} -\frac{17}{36}

Answer: Given sum: \frac{7}{24} -\frac{17}{36}

We have LCM of 24 and 36 will be, 72

Hence,

\Rightarrow \frac{7\times3}{24\times3} -\frac{17\times2}{36\times2} = \frac{21}{72} - \frac{34}{72} = \frac{-13}{72}

Question: 2(ii) Find

\frac{5}{63}-\left (\frac{-6}{21} \right )

Answer: Given \frac{5}{63}-\left (\frac{-6}{21} \right ) :

LCM of 63 and 21 is 63,

Then we have;

\Rightarrow \frac{5}{63}-\left (\frac{-6\times3}{21\times3} \right ) = \frac{5+18}{63} =\frac{23}{63}

Question: 2(iii) Find

\frac{-6}{13}-\left (\frac{-7}{15} \right )

Answer: Given \frac{-6}{13}-\left (\frac{-7}{15} \right ) :

We have, LCM of 13 and 15 is 195.

Then,

\Rightarrow \frac{-6\times15}{13\times15}-\left (\frac{-7\times13}{15\times13} \right ) = \frac{-90}{195} - \left ( \frac{-91}{195} \right ) = \frac{1}{195}

Question: 2(iv) Find

\frac{-3}{8}-\frac{7}{11}

Answer: Given \frac{-3}{8}-\frac{7}{11} :

LCM of 8 and 11 is 88, then

\Rightarrow \frac{-3}{8}-\frac{7}{11} = \frac{-3\times11}{8\times11} - \frac{7\times8}{11\times8}

\Rightarrow \frac{-33}{88} - \frac{56}{88} = \frac{-33-56}{88} = \frac{-89}{88}

Question: 2(v) Find

-2\frac{1}{9}-6

Answer: Given: -2\frac{1}{9}-6

\Rightarrow -2\frac{1}{9}-6 = \frac{-19}{9} - 6 = \frac{-19}{9} - \frac{6}{1}

LCM of 9 and 1 will be, 9

Hence,

\Rightarrow \frac{-19}{9} - \frac{6}{1} = \frac{-19}{9} - \frac{6\times9}{1\times9}

\Rightarrow \frac{-19}{9} - \frac{54}{9} = \frac{-73}{9}.

Question: 3(i) Find the product:

\frac{9}{2}\times\left ( \frac{-7}{4} \right )

Answer: Given product: \frac{9}{2}\times\left ( \frac{-7}{4} \right )

\Rightarrow \frac{9}{2}\times\left ( \frac{-7}{4} \right ) = \frac{9\times(-7)}{2\times4}

\Rightarrow \frac{-63}{8}

Question: 3(ii) Find the product:

\frac{3}{10}\times (-9)

Answer: Given \frac{3}{10}\times (-9)

\Rightarrow \frac{3}{10}\times\frac{-9}{1} = \frac{3\times(-9)}{10\times1}

So the value

\Rightarrow \frac{-27}{10}

Question: 3(iii) Find the product:

\frac{-6}{5}\times \frac{9}{11}

Answer: Given product: \frac{-6}{5}\times \frac{9}{11}

\Rightarrow \frac{-6}{5}\times \frac{9}{11} = \frac{-6\times9}{5\times11}

The value of given product is

\Rightarrow \frac{-54}{55}

Question: 3(iv) Find the product:

\frac{3}{7}\times \left (\frac{-2}{5} \right )

Answer: Given product \frac{3}{7}\times \left (\frac{-2}{5} \right )

\Rightarrow \frac{3}{7}\times(\frac{-2}{5}) = \frac{3\times(-2)}{7\times5} = \frac{-6}{35}

Question: 3(v) Find the product:

\frac{3}{11}\times \frac{2}{5}

Answer: Given product: \frac{3}{11}\times \frac{2}{5}

\Rightarrow \frac{3}{11}\times \frac{2}{5} = \frac{3\times2}{11\times5} = \frac{6}{55}

Question: 3(vi) Find the product:

\frac{3}{-5}\times \frac{-5}{3}

Answer: Given product: \frac{3}{-5}\times \frac{-5}{3}

\Rightarrow \frac{3}{-5}\times \frac{-5}{3} = \frac{3\times-5}{-5\times3} = \frac{-15}{-15} =1

Question: 4(i) Find the value of:

(-4)\div \frac{2}{3}

Answer: Given: (-4)\div \frac{2}{3}

Dividing -4 by \frac{2}{3} , we get

\Rightarrow \frac{-4}{\frac{2}{3}} =\frac{-4\times3}{2} = \frac{-12}{2} = \frac{-6}{1}

Question: 4(ii) Find the value of:

\frac{-3}{5}\div 2

Answer: Given \frac{-3}{5}\div 2

Dividing \frac{-3}{5} with 2 we get,

\Rightarrow \frac{\frac{-3}{5}}{2} = \frac{-3}{10}

Question: 4(iii) Find the value of:

\frac{-4}{5}\div (-3)

Answer: Given: \frac{-4}{5}\div (-3)

So, dividing \frac{-4}{5} with -3, we get

\Rightarrow \frac{\frac{-4}{5}}{-3} = \frac{-4}{5\times-3} = \frac{-4}{-15} = \frac{4}{15}

Question: 4(iv) Find the value of:

\frac{-1}{8}\div \frac{3}{4}

Answer: Given: \frac{-1}{8}\div \frac{3}{4}

Simplifying it:

\Rightarrow \frac{-1}{8}\times\frac{4}{3} = \frac{-1\times4}{8\times3}

\Rightarrow \frac{-4}{24} = \frac{-1}{6}

Question: 4(v) Find the value of:

\frac{-2}{13}\div \frac{1}{7}

Answer: Given: \frac{-2}{13}\div \frac{1}{7}

Simplifying it: we get

\Rightarrow \frac{-2}{13}\times\frac{7}{1} = \frac{-14}{13}

Question: 4(vi) Find the value of:

\frac{-7}{12}\div \left (\frac{-2}{3} \right )

Answer: Given: \frac{-7}{12}\div \left (\frac{-2}{3} \right )

Simplifying it: we get

\Rightarrow \frac{-7}{12}\times\frac{3}{-2} = \frac{-7\times3}{12\times-2} = \frac{-21}{-24} = \frac{7\times3}{8\times3} = \frac{7}{8}

Question: 4(vii) Find the value of:

\frac{3}{13}\div \left (\frac{-4}{65} \right )

Answer: Given: \frac{3}{13}\div \left (\frac{-4}{65} \right )

Simplifying it: we get

\\\Rightarrow \frac{3}{13}\div \left (\frac{-4}{65} \right ) = \frac{3}{13}\times\frac{65}{-4} = \frac{3\times65}{13\times-4} \\since\ 13\times5=65 \\\ \frac{3}{13}\times\frac{65}{-4} =\frac{15}{-4}

Rational Numbers Class 7 Maths Chapter 9-Topics

  • Need For Rational Numbers
  • What Are The Rational Numbers?
  • Positive And Negative Rational Numbers
  • Rational Numbers On A Number Line
  • Rational Numbers In A Standard Form
  • Comparison Of Rational Numbers
  • Rational Numbers Between Two Rational Numbers
  • Operations On Rational Numbers

NCERT Solutions for Class 7 Maths Chapter Wise

Chapter No.

Chapter Name

Chapter 1

Integers

Chapter 2

Fractions and Decimals

Chapter 3

Data Handling

Chapter 4

Simple Equations

Chapter 5

Lines and Angles

Chapter 6

The Triangle and its Properties

Chapter 7

Congruence of Triangles

Chapter 8Comparing quantities

Chapter 9

Rational Numbers

Chapter 10

Practical Geometry

Chapter 11

Perimeter and Area

Chapter 12

Algebraic Expressions

Chapter 13

Exponents and Powers

Chapter 14

Symmetry

Chapter 15Visualising Solid Shapes

NCERT Solutions for Class 7 Subject Wise

NCERT Solutions for Class 7 Maths

NCERT Solutions for Class 7 Science

Benefits of NCERT Solutions for Class 7 Maths Chapter 9 Rational Numbers

  • You will study the different types of numbers which you should know in NCERT Class 7 Mathematics book .

  • It will help you in your homework as all the practice questions including questions given below every topic are covered in this article.
  • You will also learn addition, multiplication, subtraction, and division of rational numbers.
  • You will also get solutions to the practice questions given below every topic which will give you conceptual clarity.
  • You should practice all the NCERT questions including examples. If you facing difficulties in doing so, you can take help from the NCERT solutions for Class 7 Maths chapter 9 Rational Numbers.

Happy learning!!!

Also Check NCERT Books and NCERT Syllabus here:

Also Read

Articles

Upcoming School Exams

National Institute of Open Schooling 12th Examination

Admit Card Date:04 October,2024 - 29 November,2024

National Institute of Open Schooling 10th examination

Admit Card Date:04 October,2024 - 29 November,2024

Mizoram Board of School Education 12th Examination

Application Date:07 October,2024 - 22 November,2024

Mizoram Board 10th Examination

Application Date:07 October,2024 - 22 November,2024

Punjab Board of Secondary Education 10th Examination

Application Correction Date:08 October,2024 - 27 November,2024

View All School Exams
Get answers from students and experts

Popular Questions

A block of mass 0.50 kg is moving with a speed of 2.00 ms-1 on a smooth surface. It strikes another mass of 1.00 kg and then they move together as a single body. The energy loss during the collision is

Option 1)

0.34\; J

 Option 2)

0.16\; J
Option 3)

1.00\; J

 Option 4)

0.67\; J
Read More

A person trying to lose weight by burning fat lifts a mass of 10 kg upto a height of 1 m 1000 times.  Assume that the potential energy lost each time he lowers the mass is dissipated.  How much fat will he use up considering the work done only when the weight is lifted up ?  Fat supplies 3.8×107 J of energy per kg which is converted to mechanical energy with a 20% efficiency rate.  Take g = 9.8 ms−2 :

Option 1)

2.45×10−3 kg

 Option 2)

 6.45×10−3 kg
Option 3)

 9.89×10−3 kg

 Option 4)

12.89×10−3 kg

 
Read More

An athlete in the olympic games covers a distance of 100 m in 10 s. His kinetic energy can be estimated to be in the range

Option 1)

2,000 \; J - 5,000\; J

 Option 2)

200 \, \, J - 500 \, \, J
Option 3)

2\times 10^{5}J-3\times 10^{5}J

 Option 4)

20,000 \, \, J - 50,000 \, \, J
Read More

A particle is projected at 600   to the horizontal with a kinetic energy K. The kinetic energy at the highest point

Option 1)

K/2\,

 Option 2)

\; K\;
Option 3)

zero\;

 Option 4)

K/4
Read More

In the reaction,

2Al_{(s)}+6HCL_{(aq)}\rightarrow 2Al^{3+}\, _{(aq)}+6Cl^{-}\, _{(aq)}+3H_{2(g)}

Option 1)

11.2\, L\, H_{2(g)}  at STP  is produced for every mole HCL_{(aq)}  consumed

 Option 2)

6L\, HCl_{(aq)}  is consumed for ever 3L\, H_{2(g)}      produced
Option 3)

33.6 L\, H_{2(g)} is produced regardless of temperature and pressure for every mole Al that reacts

 Option 4)

67.2\, L\, H_{2(g)} at STP is produced for every mole Al that reacts .
Read More

How many moles of magnesium phosphate, Mg_{3}(PO_{4})_{2} will contain 0.25 mole of oxygen atoms?

Option 1)

0.02

 Option 2)

3.125 × 10-2
Option 3)

1.25 × 10-2

 Option 4)

2.5 × 10-2
Read More

If we consider that 1/6, in place of 1/12, mass of carbon atom is taken to be the relative atomic mass unit, the mass of one mole of a substance will

Option 1)

decrease twice

 Option 2)

increase two fold
Option 3)

remain unchanged

 Option 4)

be a function of the molecular mass of the substance.
Read More

With increase of temperature, which of these changes?

Option 1)

Molality

 Option 2)

Weight fraction of solute
Option 3)

Fraction of solute present in water

 Option 4)

Mole fraction.
Read More

Number of atoms in 558.5 gram Fe (at. wt.of Fe = 55.85 g mol-1) is

Option 1)

twice that in 60 g carbon

 Option 2)

6.023 × 1022
Option 3)

half that in 8 g He

 Option 4)

558.5 × 6.023 × 1023
Read More

A pulley of radius 2 m is rotated about its axis by a force F = (20t - 5t2) newton (where t is measured in seconds) applied tangentially. If the moment of inertia of the pulley about its axis of rotation is 10 kg m2 , the number of rotations made by the pulley before its direction of motion if reversed, is

Option 1)

less than 3

 Option 2)

more than 3 but less than 6
Option 3)

more than 6 but less than 9

 Option 4)

more than 9
Read More

Colleges After 12th

Popular Course After 12th

Applications for Admissions are open.

VMC VIQ Scholarship Test
Apply

Register for Vidyamandir Intellect Quest. Get Scholarship and Cash Rewards.

JEE Main Important Physics formulas
Apply

As per latest 2024 syllabus. Physics formulas, equations, & laws of class 11 & 12th chapters

JEE Main Important Chemistry formulas
Apply

As per latest 2024 syllabus. Chemistry formulas, equations, & laws of class 11 & 12th chapters

TOEFL ® Registrations 2024
Apply

Accepted by more than 11,000 universities in over 150 countries worldwide

Pearson | PTE
Apply

Register now for PTE & Unlock 20% OFF : Use promo code: 'C360SPL20'. Valid till 30th NOV'24! Trusted by 3,500+ universities globally

JEE Main high scoring chapters and topics
Apply

As per latest 2024 syllabus. Study 40% syllabus and score upto 100% marks in JEE

View All Application Forms
News and Notifications
Govt informs SC about NCERT guidelines for development of e-content for children with disabilities
Government school principal in Andhra chops off girl students' hair for coming late
Delhi Air Pollution: DU, JNU, Jamia shift to online classes; Delhi, Gurugram schools suspend physical sessions
‘Are the lungs of teachers made of steel?’ MCD teachers say admin forcing them to attend school
Bihar teacher accused of causing serious eye injury to 12-year-old student for skipping homework
Delhi schools closed: Physical classes to discontinue for students, says CM Atishi
GRAP IV curbs on Delhi-NCR from Monday; states may close schools, allow work from home
Back to top