NCERT Solutions for Class 6 Maths Chapter 6 - Perimeter and Area

Perimeter and area are one of the fundamental concepts in mathematics that help students understand the way to calculate the length of the boundary and the area enclosed within the boundary. Perimeter refers to the length of the boundary, while area refers to the surface area enclosed within the boundary. These perimeter and area play a major role in many real-life situations, like calculating the amount of paint required to paint a wall and the length of the fence required, etc. This articles consist of the solutions for all the questions in this chapter. NCERT Solutions Class 6 Maths Chapter 6 Perimeters and Areas will help you to understand all the concepts related to it.

These solutions provide comprehensive step-by-step solutions for all the questions in this chapter. These reliable NCERT Solutions for Class 6 Maths can be used by the students for exam preparation as they are created by the subject matter experts of Careers360. Students can refer to the NCERT Solutions for Class 6 to access the subject-wise solutions of Class 6.

NCERT Solutions for Class 6 Maths Chapter 6 Exercise

Q1. Find the missing terms:

a. Perimeter of a rectangle =14 cm; breadth =2 cm; length = ?

b. Perimeter of a square =20 cm; side of a length = ?

c. Perimeter of a rectangle =12 m; length =3 m; breadth = ?

Solution:

a. Given: Perimeter of a rectangle =14 cm, breadth =2 cm

Perimeter of Rectangle = 2×(L+B), where L is the length and B is the breadth of the rectangle.

⇒ 14=2×(L+2)

⇒ 7=L+2

⇒ L=5 cm

b. Given: Perimeter of a Square =20 cm

Perimeter of square = 4×(side)

20=4×(sides)

⇒ 204=side

⇒ side=5 cm

c. Given: Perimeter of a rectangle =12 m; length =3 m

The perimeter of a rectangle is P=2(l+b), where l is the length and b is the breadth of the rectangle.

⇒ 12=2(3+b)

⇒ 6=3+b

⇒ b=6−3=3 cm

Q2. A rectangle having sidelengths 5 cm and 3 cm is made using a piece of wire. If the wire is straightened and then bent to form a square, what will be the length of a side of the square?

Solution:
Perimeter of the rectangle =2×(l+b), where l is the length and b is the breadth of the rectangle.

Here perimeter of the rectangle =2(5+3)

=2×8

=16 cm

Now the wire is straightened and then bent to form a square.

Perimeter of Square =4×a, where a is the side of the square.

∴ Perimeter of square =16 cm

⇒ 4a=16 cm

⇒ a=4 cm, the required length of the side of the square.

Q3. Find the length of the third side of a triangle having a perimeter of 55 cm and having two sides of length 20 cm and 14 cm, respectively.

Solution:
Perimeter of a Triangle = Sum of all three sides of the triangle

First side of the triangle =20 cm

Second side of the triangle =14 cm

Let the third side of the triangle be ' x '

Perimeter of the triangle =55 cm

∴20+14+x = 55cm

⇒ 34+x = 55cm

⇒ x = 55−34

⇒ x = 21

∴ The length of the third side of the triangle is 21 cm.

Q4. What would be the cost of fencing a rectangular park whose length is 150 m and breadth is 120 m, if the fence costs ₹ 40 per metre?

Solution:
Perimeter of the rectangle =2(l+b) [Where ‘l’ is the length of the Park and ‘b’ is the breadth of the Park]

The length of the fence is the perimeter of the rectangular park.

Given that the length of the rectangular park =150 m and the breadth = 120 m

= 2×(150+120)

= 2×(270)

= 540m

Now the cost of fencing per meter = Rs 40

The cost of fencing the rectangular park is = Rs 40 × 540 = Rs. 21600

Q5. A piece of string is 36 cm long. What will be the length of each side, if it is used to form:

a. A square

b. A triangle with all sides of equal length, and

c. A hexagon (a six-sided closed figure) with sides of equal length?

Solution:
Given: A piece of string is 36 cm long

a. Given that a piece of string is 36 cm long

∴ length of each side of the square =a,

Perimeter of a Square =4×a

Perimeter =36
⇒ 4a=36
⇒ a=364
⇒ a=9 cm

b. Length of each side of the triangle =a (Given)

Perimeter of a triangle = Sum of all sides of a Triangle

Perimeter =36
⇒ 3a=36
⇒ a=363
⇒ a=12 cm

c. Length of each side of the hexagon =x

Perimeter of a Hexagon = Sum of all sides of a hexagon

Perimeter = 36
⇒ 3a=36
⇒ a=363
⇒ a=12 cm

Q6. A farmer has a rectangular field with a length of 230 m and a breadth of 160 m. He wants to fence it with 3 rounds of rope as shown. What is the total length of rope needed?

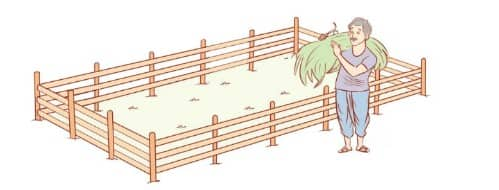
Solution: Perimeter of the rectangular field =2(l+b)

Given l = 230 m, b = 160 m
∴ P = 2×(230+160)
= 2×390
= 780 m

Distance covered in one round = 780 m

∴ Required length of rope = 3×780 = 2340 m

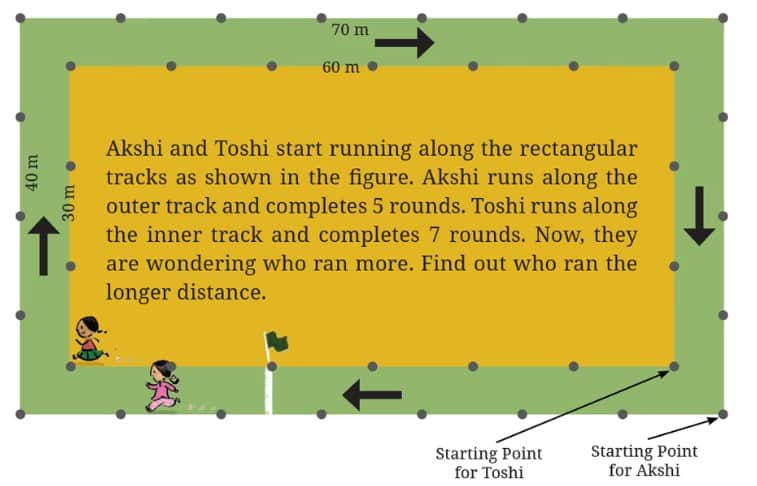
Each track is a rectangle. Akshi's track has a length of 70 m and a breadth of 40 m. Running one complete round on this track would cover 220 m, i.e., 2×(70+40)m = 220 m. This is the distance covered by Akshi in one round.

Q1. Find out the total distance Akshi has covered in 5 rounds.

Solution:
Distance covered by Akshi in 5 rounds =5× perimeter of outer rectangle

=5×220

=1100 m

Q2. Find out the total distance Toshi has covered in 7 rounds. Who ran a longer distance?

Solution:
Distance covered by Toshi in 7 rounds =7×180=1260 m ∴1260 m>1100 m

Hence, Toshi ran the longer distance.

Q3. Think and mark the positions as directed-

a. Mark 'A' at the point where Akshi will be after she has run 250 m.

b. Mark 'B' at the point where Akshi will be after she has run 500 m.

c. Now, Akshi ran 1000 m. How many full rounds has she finished running around her track? Mark her position as 'C'.

d. Mark ' X ' at the point where Toshi will be after she has run 250 m.

e. Mark ' Y ' at the point where Toshi will be after she has run 500 m.

f. Now, Toshi ran 1000 m. How many full rounds has she finished running around her track? Mark her position as ' Z '.

Solution:

a. Here, one round of a rectangular field is =220 meters.

The distance Akshi has run is =250 meters.

Extra distance beyond one round =250−220=30 meters.

Since Akshi has already completed one full round, she will be 30 meters into her second round.

Thus, after running an additional 30 meters, she will be on the length side of the track, 30 meters from the starting point. Therefore, mark 'A' at the point 30 meters along the length of the track from the starting point.

b. Distance per round =220 meters

Total distance Akshi runs = 500 meters.

First, we will find out how many complete rounds she runs:

Number of complete rounds =500220=2.27 (approx)

This means Akshi completes 2 full rounds and then runs an additional distance =500−(2×220)=60 m Therefore, Akshi will be 60 meters along the length of the track from the starting point, we can mark point ' B ' at this position on the track.

c. Now, Akshi ran 1000 meters.

Number of full rounds =1000220=4.545 rounds.

Akshi has completed 4 full rounds and is partway through her 5th round.

To find her position on the track, we calculate the remaining distance after 4 full rounds:

Remaining distance =1000 m−(4×220 m)

=1000−880

=120 m

Since she has run an additional 120 meters after completing 4 full rounds, her position will be 120 meters from the starting point. If we mark her starting point as 'P', her position after running 1000 meters can be marked as ' C ', which is 120 meters from ' A ' along the track.

d. Perimeter of the track =180 meters

Distance Toshi runs =250 meters

Since 250 meters is more than one complete round ( 180 meters), Toshi will have completed one full round and will have 70 meters left to run ( 250−180=70 meters). So, Toshi will be 70 meters along the length of the track from the starting point. You can mark ' X ' at this point on the track.

e. Given that Toshi has run an additional 140 meters after completing 2 rounds, her position will be 140 meters from the starting point. If we mark her starting point as ' A ', her position after running 500 meters can be marked as ' Y '.

f. Number of full rounds =1000180=5.56

Toshi has finished 5 full rounds.

Remaining distance =1000 meters −(5×180 meters )

=1000 meters −900 meters

=100 meters

Starting from the initial point, Toshi would be 100 meters into her 6th round. Since the track is 60 meters long and 30 meters wide, she would be somewhere along the length of the track.

Let's mark this position as ' Z '.

Q1. The area of a rectangular garden 25 m long is 300 sq m. What is the width of the garden?

Solution:
Given, area of rectangular garden =300 sq.m and length =25 m

Area of rectangular field = l×b
⇒ 300 = 25×b
⇒ b = $\frac{300}{25}$ m
⇒ b = 12 m

Q2. What is the cost of tiling a rectangular plot of land 500 m long and 200 m wide at the rate of Rs.8 per hundred sq m?

Solution: Length =500 m and breadth =200 m

Hence, the area of the rectangular plot = length × breadth
= 500×200
= 1,00,000 m²

Now the cost of tilling a rectangular plot is Rs. $\frac{8}{100}$ per sq. m

Hence, the cost of tilling 1,00,000 sq. m of rectangular plot = $\frac{8}{100}$ × 100000 = Rs. 8,000

Q3. A rectangular coconut grove is 100 m long and 50 m wide. If each coconut tree requires 25 sq m, what is the maximum number of trees that can be planted in this grove?

Solution: Area of rectangular coconut grove = 100×50 = 5000 sq. m

Given each coconut tree requires 25 sq. m, then the maximum no. of trees that can be planted in this grove is = $\frac{5000}{25}$ = 200

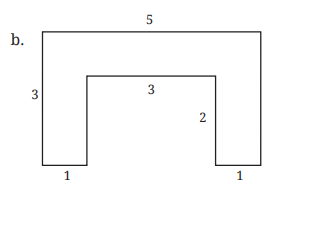
Q4. By splitting the following figures into rectangles, find their areas (all measures are given in metres).

a.

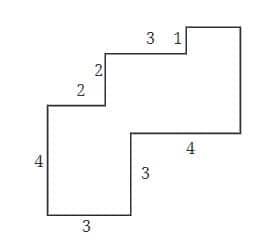
Solution:
a.

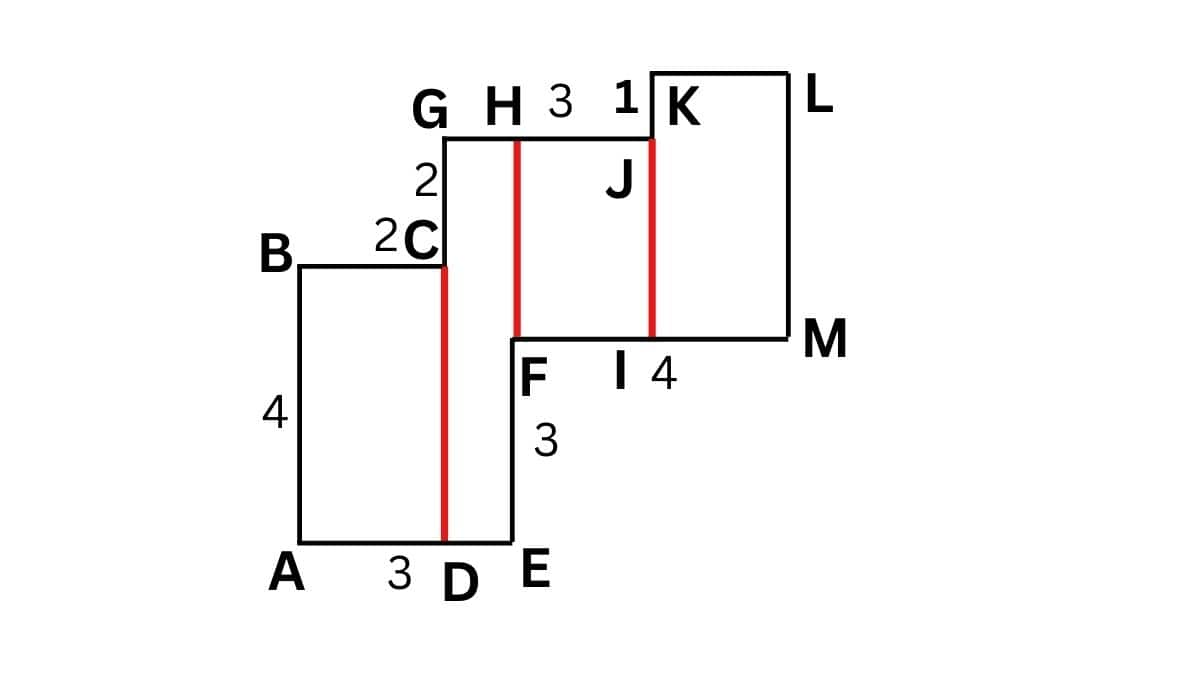
There will be four rectangles as shown in the figure.
Total Area = Area of rectangle ABCD + Area of rectangle DGHE + Area of rectangle FHKI + Area of rectangle KLMI
= AB×BC + DG×DE + HF×JH + LM×KL
= 4×2 +(4+2)×(3−2) + (4+2−3)×(3−1) + (3+1)×(1+4−3)
= 8+6+6+8
= 28 m²

b.

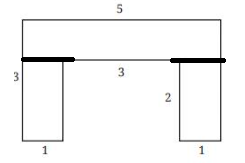
There are three rectangles as shown in the figure
So, the required area = Area of the sum of three triangles
= 5×1 + 2×1 + 2×1
= 5+2+2
= 9 m²

Cut out the tangram pieces given at the end of your textbook.

Q1. Explore and figure out how many pieces have the same area.

Solution:
Shapes C and E are the two pieces with the same area. Similarly, A and B also have the same area.

Q2. How many times bigger is Shape D as compared to Shape C? What is the relationship between Shapes C, D, and E?

Solution:
As you can see in the figure, shape D is twice the size of Shape C.

The area of Shape D is equal to the sum of the areas of Shape C and Shape E.

⇒ Shape C + Shape E = Shape D.

Q3. Which shape has more area: Shape D or F? Give reasons for your answer.

Solution:
Both Shape D and Shape F have equal area.

It's because Shape C and Shape E together fit perfectly into both Shape D and Shape F.

Q4. Which shape has more area: Shape F or G? Give reasons for your answer.

Solution:
Both Shape F and Shape G have equal area.

It's because Shape C and Shape E together fit perfectly into both Shape F and Shape G.

Q5. What is the area of Shape A as compared to Shape G? Is it twice as big? Four times as big?

(Hint: In the tangram pieces, by placing the shapes over each other, we can find out that Shapes A and B have the same area, Shapes C and E have the same area. You would have also figured out that Shape D can be exactly covered using Shapes C and E, which means Shape D has twice the area of Shape C or Shape E, etc.)

Solution:
The area of Shape A is twice as big as Shape G.

Q6. Can you now figure out the area of the big square formed with all seven pieces in terms of the area of Shape C?

Solution:
The area of the big square is 16 times the area of Shape C.

Q7. Arrange these 7 pieces to form a rectangle. What will be the area of this rectangle in terms of the area of Shape C now? Give reasons for your answer.

Solution:
The area of the rectangle formed by combining all 7 pieces will be 16 times the area of Shape C. This is because the total area of all the pieces will remain the same, regardless of how they are aligned.

Q8. Are the perimeters of the square and the rectangle formed from these 7 pieces different or the same? Explain your answer.

Solution:
The perimeter of the square and the rectangle formed from these 7 pieces will be different. This is because the distance around the outside of the shape is different.

1. Find the areas of the figures below by dividing them into rectangles and triangles.

Solution:

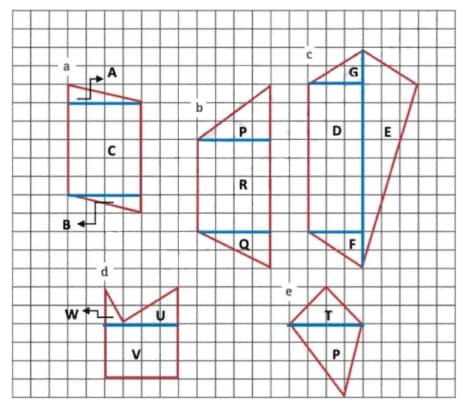
(a) Area of the figure = Area of triangle A+ Area of triangle B+ Area of rectangle C
= $\frac{1}{2}$ × Base × Height + $\frac{1}{2}$ × Base × Height + Length × Breadth
= (2+20+2) sq. units
= 24 sq. units.

(b) Area of the figure = Area of triangle P+ Area of triangle Q+ Area of rectangle R

= $\frac{1}{2}$ × Base × Height + $\frac{1}{2}$ × Base × Height + Length × Breadth
= 6 sq. units +4 sq. units +20 sq. units
= 30 sq. units.

(c) Area = Area of triangle G + Area of triangle E + Area of triangle F + Area of rect. D
= $\frac{1}{2}$ × Base × Height + $\frac{1}{2}$ × Base × Height + Length × Breadth
= 3 sq. units +18 sq. units +3 sq. units +24 sq. units
= 48 sq. units.

(d) Area of the figure = Area of triangle W+ Area of triangle U+ Area of rectangle V
= $\frac{1}{2}$ × Base × Height + $\frac{1}{2}$ × Base × Height + Length × Breadth
= 1 sq. units +3 sq. units +12 sq. units
= 16 sq. units.

(e) Area of the figure = Area of triangle T+ Area of triangle P
= $\frac{1}{2}$ × Base × Height + $\frac{1}{2}$ × Base × Height
= 4 sq. units +7 sq. units
= 11 sq. units.

Q1. Give the dimensions of a rectangle whose area is the sum of the areas of these two rectangles having measurements: 5 m×10 m and 2 m×7 m.

Solution:
The sum of the areas of these two rectangles = (5×10+2×7) sqm = 64sqm

Now, the rectangle has an area of 64m2

So, the dimensions of the rectangle =16 m×4 m

Q2. The area of a rectangular garden that is 50 m long is 1000 sq m. Find the width of the garden.

Solution:
Length of a rectangular garden =50 m
Area of the rectangular garden =1000 sq m

Now,
∴ Area of the rectangular garden = length × width
∴1000 = 50× width
∴ Width = $\frac{1000}{50}$ = 20
So, the width of the rectangular garden =20 m

Q3. The floor of a room is 5 m long and 4 m wide. A square carpet whose sides are 3 m in length is laid on the floor. Find the area that is not carpeted.

Solution:
Length of a room =5 m
Width of the room =4 m

So, area of the room = Length of a room×Width of the room = 5×4 sqm = 20 sqm

Now, the length of the sides of a square carpet is =3 m

Area of the carpet =(length of the sides of a square carpet)² = 3×3sqm =9 sqm

The area that is not carpeted is = (20−9) sqm = 11 sqm

Q4. Four flower beds, having sides 2 m long and 1 m wide, are dug at the four corners of a garden that is 15 m long and 12 m wide. How much area is now available for laying down a lawn?

Solution:
Length of garden = 15 m

Width of garden =12 m

So, the area of the garden =Length of Garden×Width of Garden=15×12sqm=180sqm

Now, the length of the flower bed =2 m

Width of flower bed =1 m

Area of the flower bed =Length of flower bed×Width of flower bed=2×1sqm=2sqm

Since the area of four flower beds =2×4sqm=8sqm

Now the area is available for laying down a lawn =(180−8) sq m = 172 sqm

Q5. Shape A has an area of 18 square units, and Shape B has an area of 20 square units. Shape A has a longer perimeter than Shape B. Draw two such shapes satisfying the given conditions.

Solution:

Shape A

Area = 9×2 = 18 square units
Perimeter = 9 + 9 + 2 + 2 = 22 units

Shape B

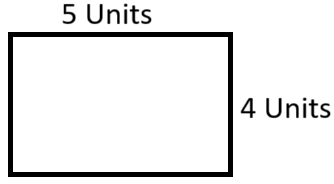
Area = 5×4=20 square units
Perimeter = 5 + 5 + 4 + 4 = 20 units

Clearly, shape A has more perimeter than that of B.

Q6. On a page in your book, draw a rectangular border that is 1 cm from the top and bottom and 1.5 cm from the left and right sides. What is the perimeter of the border?

Solution:
Let the width of the page =W cm
Width of the border =W−1.5 cm (left margin) −1.5 cm (right margin) =W−3 cm.

Let the height of the page =H cm
Height of the border =H−1 cm (top margin) −1 cm (bottom margin) =H−2 cm.

Perimeter of the border =2×(Width of the border + Height of the border)
=2×((W−3)+(H−2))=2×(W+H−5)
=2(W+H)−10

The perimeter of the border is 2(W+H)−10 cm.

Q7. Draw a rectangle of size 12 units ×8 units. Draw another rectangle inside it, without touching the outer rectangle that occupies exactly half the area.

Solution: Area of rectangle =12×8 sq units =96 sq units

The area of the new rectangle is half of this rectangle, so the area of the new rectangle = $\frac{1}{2}$ × 96 sq m = 48 sq m

The possible dimensions of the new rectangle = 8 units ×6 units

Q8. A square piece of paper is folded in half. The square is then cut into two rectangles along the fold. Regardless of the size of the square, one of the following statements is always true. Which statement is true here?

a. The area of each rectangle is larger than the area of the square.

b. The perimeter of the square is greater than the perimeter of both the rectangles added together.

c. The perimeters of both the rectangles added together are always 1$\frac{1}{2}$ times the perimeter of the square.

d. The area of the square is always three times as large as the areas of both rectangles added together.

Solution:

(a) False because the area of each rectangle is exactly half of the area of the square.

(b) False because the perimeter of the two resulting rectangles combined is greater than the perimeter of the square, as now we have two extra edges.

(c) True.
Let the side of the square be x units.
After cutting it into two equal parts, the dimensions of each rectangle will be $x$ and $\frac{x}{2}$ units.
Perimeter of square = $4x$
Perimeter of each rectangle = $2\times (x+\frac{x}{2})=3x$
Sum of the perimeter of two rectangles = $6x=\frac{3}{2}\times 4x$
Clearly, the Sum of the perimeters of two rectangles = 1$\frac{1}{2}$ × the perimeter of the square

(d) False because the area of the square is equal to the sum of the areas of both the rectangles combined.

Perimeter and Area Class 6 Maths Chapter 6: Topics

Given below are the key topics for the NCERT class 6 maths chapter 6, Perimeter and Area:

• Perimeter
• Area

NCERT Solutions for Class 6 Maths Chapter 6 Perimeter and Area - Notes

All the important concepts, formulas, and definitions of the NCERT class 6 maths chapter 6, Perimeter and Area, are given below as notes:

Perimeter and Area:

Perimeter is defined as the boundary that is covered by a 2-dimensional shape, while Area is the space enclosed within the boundary of the specific shape.

In the previous class, we learned about the perimeter and area of some simple shapes like rectangles and squares. In class sixth, we will take our journey further and enhance our conceptual knowledge regarding the perimeter and area of triangles and other polygons that we commonly come across in geometry.

Perimeter and Area of Different Shapes

Rectangle

A rectangle is defined as a two-dimensional shape that has two pairs of equal and opposite sides.

Perimeter of a rectangle: The perimeter of a rectangle is given by P = 2(length + breadth)

Area of a rectangle: The area of a rectangle is given by the formula, length × breadth (i.e,) l×b.

Square

A square is a two-dimensional figure that has all its sides equal in measurement. All the angles of a square are equal to 90 degrees.

Perimeter of a Square: The perimeter of a square is given by P = 4 × side (i.e,) 4×s

Area of a square: The area of a square is given by the formula, side × side (i.e,) s×s.

Triangle

A triangle is a three-sided polygon.

Perimeter of a triangle: The perimeter of a triangle is given by P = the sum of all three sides. (i.e.) P = a+b+c

Regular Polygon

Like squares, closed figures that have all sides and all angles equal are called regular polygons. A few examples of regular polygons are the equilateral triangle (where all three sides and all three angles are equal), and the regular pentagon (where all five sides and all five angles are equal).

Perimeter of a regular polygon: The perimeter of a regular polygon is given by P = number of sides × length of sides

For example, the perimeter of an equilateral triangle = 3 × the length of a side.

NCERT Solutions for Class 6 Maths Chapter 6 Perimeter and Area - Points to Remember

Given below are some important points about the NCERT class 6 maths chapter 6, Perimeter and Area:

Perimeter:

Perimeter of a square: P = 4 × side
Perimeter of a rectangle: P = length × breadth
Perimeter of a triangle: P = sum of all three sides
Perimeter of a regular polygon: P = number of sides × length of the side

Area:

Area of a square: A = side × side
Area of a rectangle: A = length × breadth

NCERT Solutions for Class 6 Maths Chapter Wise

Here are all the links for chapter-wise NCERT Solutions of Class 6 Maths:

NCERT Solutions for Class 6 Subject Wise

It is very important for the students to practice the exercise questions during the exam preparation. So, the students can refer to the subject-wise solutions prepared by the subject matter experts at Careers360 using the link below.

• NCERT Solutions for Class 6 Maths
• NCERT Solutions for Class 6 Science

Students can also check the NCERT Books and the NCERT Syllabus here:

• NCERT Syllabus Class 6 Maths
• NCERT Books Class 6
• NCERT Syllabus Class 6