What Are Exponential And Logarithmic Functions, And How Do They Work?

In the humongous world of maths, two special things called exponential and logarithmic functions are like superheroes. They help us understand how things grow really fast or shrink super quick. These maths powers are not just cool; they're also super important in real-life, to understand how things change over time.

In schools, these maths concepts are introduced in Class 8 and gradually detailed in Class 11 and 12. For tests and exams, knowing about these functions is like having a secret weapon. Questions from the function of real life issues such as money growing fast, how populations change, or even how things break down slowly, limit, continuity, differentations, integrations, differential equations, geometry and many more chapters used these concepts. Even in big tests to get into college, understanding these functions can make a big difference in solving tricky problems.

But wait, these maths concepts aren't just for exams! In everyday life, they're like a secret code helping us understand things around us. They help in planning money expenditure, savings and investments, understanding trends in information, and even figuring out things like how old stuff is or how earthquakes shake things.

So, in this article, we're going to explore these maths functions. We'll learn how they work, what makes them special, and how they're everywhere around us. So keep reading the article to know exponential and logarithmic functions in detail.

Fundamentals of Exponential Functions

Exponential functions are essential in mathematics. They represent growth or decay that occurs at a constant percentage rate. An exponential function takes the form: f(x) = ab^x.

Where, a is the initial value (or starting amount), b is the base of the exponential function (also known as the growth/decay factor), and x represents the exponent.

>> Growth: When b > 1, the function represents exponential growth. As x increases, the function value grows rapidly.

>> Decay: When 0 < b < 1, the function represents exponential decay. As x increases, the function value diminishes.

Exponential functions have a unique characteristic: their rate of growth/decay is proportional to the current value. The graph of an exponential function typically exhibits a steep upward or downward curve, depending on whether it's growth or decay.

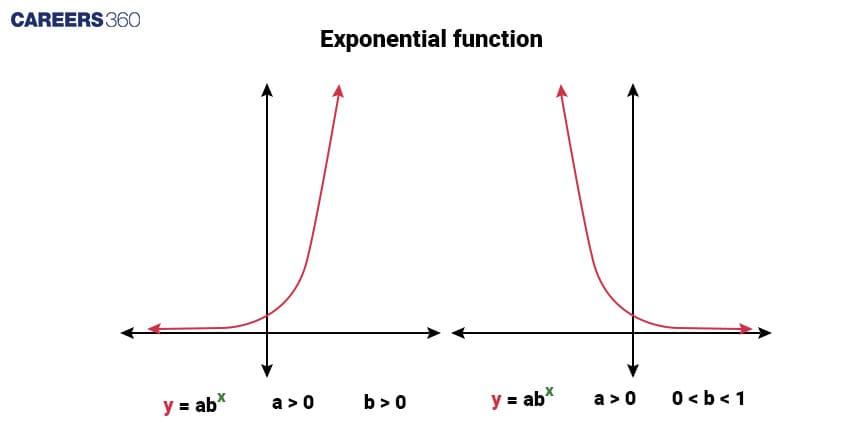
Exponential functions are prevalent in various fields like finance (compound interest), biology (population growth), physics (radioactive decay), and more.

Let's imagine a place where there are 100 people infected with COVID-19 on the first day. Every three days, the number of infected people doubles.

After 3 days: 200 people are infected.

After 6 days: 400 people are infected (doubled again).

After 9 days: 800 people are infected.

After 12 days: 1600 people are infected.

After 15 days: 3200 people are infected.

After 18 days: 6400 people are infected.

After 21 days: 12800 people are infected.

After 24 days: 25600 people are infected.

After 27 days: 51200 people are infected.

After 30 days: 102400 people are infected.

This simple explanation illustrates how the number of cases grows very quickly, starting with a small number and doubling every three days. It emphasises how important it is to take measures to slow down the spread of diseases like COVID-19, especially in the early stages when cases can multiply rapidly. Here the concept of exponential growth comes into play and helps us understand the spread of Covid-19 and project expected cased.

These concepts of exponential growth help us to take measures like lockdown to fight against communicable diseases like Covid-19. Also, understanding exponencial fundamentals can assist in solving problems involving exponential growth/ decay, determining rates, interpreting graphs, and making predictions in real-life scenarios.

Also check - What Is The Mathematics Behind The Google Search Results?

Unravelling Logarithmic Functions

Logarithmic functions are crucial in mathematics. They showcase relationships between exponential growth/decay and the power required by a specific number, termed the base, to achieve a given value. The general form of a logarithmic function is y = log_b(x),

Where: y denotes the logarithm of x with base b. x represents the argument of the logarithm. b is the base of the logarithm.

Logarithmic functions serve as the inverse of exponential functions, reversing their effects. For instance, if y = b^x is an exponential function, then x = log_b(y) acts as its inverse logarithmic function.

Exponential functions and logarithmic functions are interrelated to each other as shown in the graph.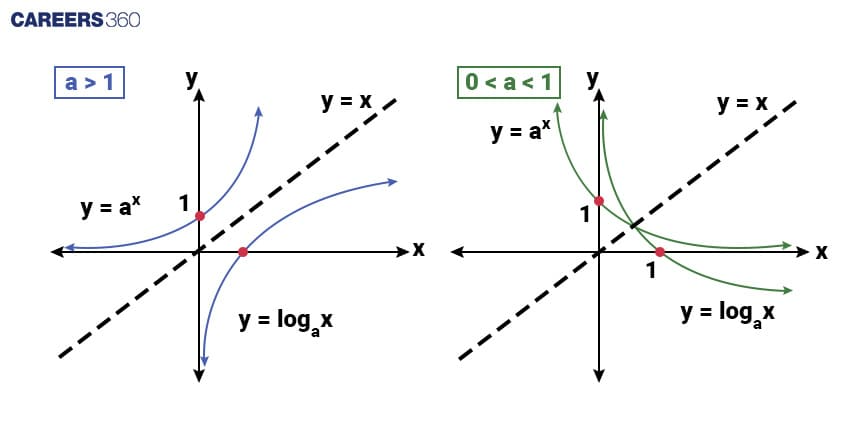

Properties of Logarithmic function

>> Product Rule: When you multiply numbers inside a logarithm (log), it's the same as adding their logs.

log(A * B) = log(A) + log(B).

>> Quotient Rule: When you divide numbers inside a logarithm, it's like subtracting their logs.

log(A / B) = log(A) - log(B).

>> Power Rule: If you have a power inside a log, you can bring that power outside as a multiplier.

Log(A^p) = p log(A).

Logarithmic functions feature vertical asymptotes, especially for bases less than 1. Their growth is slow as x increases, particularly for larger values of x. The graph of a logarithmic function shows a line that either slowly goes up or gently slopes down which is represented by the graph below.

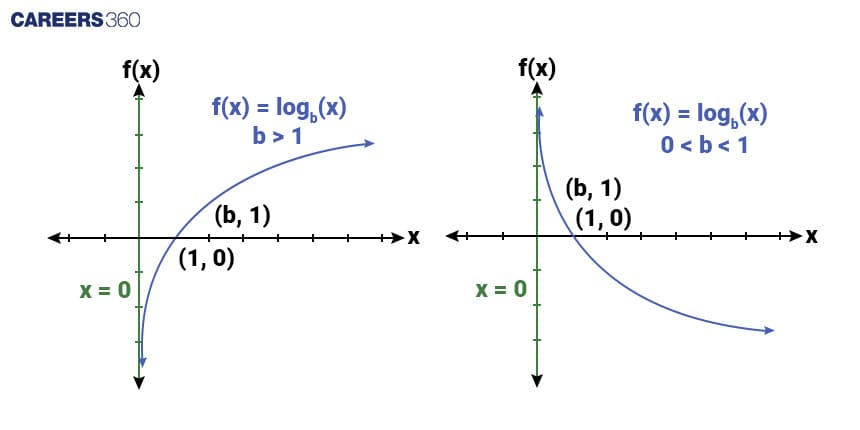
Logarithms find application across various fields, including science, engineering, finance, etc. They help calculate pH in chemistry, measure sound intensity in decibels, represent data on logarithmic scales, etc.

In chemistry, the pH scale tells us if a liquid is acidic or basic. Logarithms are used in pH calculations to measure how strong acids or bases are.

The pH number shows how many hydrogen ions are in a solution. The lower the pH, the more hydrogen ions, and the stronger the acid. A higher pH means fewer hydrogen ions and a stronger base.

Here's the formula: pH = -log[H+]

pH is the pH number we're finding.

[H+] is the concentration of hydrogen ions in the liquid.

Let's say a liquid has a hydrogen ion concentration of 0.0001 moles per litre ([H+] = 0.0001 M). To find the pH:

pH = -log(0.0001)

Using some maths, we get pH = 4.

So, a liquid with a hydrogen ion concentration of 0.0001 M has a pH of 4, which means it's a bit acidic. Logarithms help us understand how acidic or basic a liquid is by condensing big numbers into smaller ones, making it easier for chemists to work with and understand the strengths of acids and bases.

Understanding logarithmic functions aids in solving exponential equations, analysing data on logarithmic scales, and comprehending various real-world phenomena where quantities change at varying rates. They are fundamental in mathematics and play a significant role in scientific and technical applications.

Also check - Understanding The Power Of Synthetic Biology For A Transformed Future

Hope this helps you to understand the mathematics of Exponential and Logarithmic functions concepts such as product rule, quotient rule and many more, and how these concepts can be applied in real life issues.