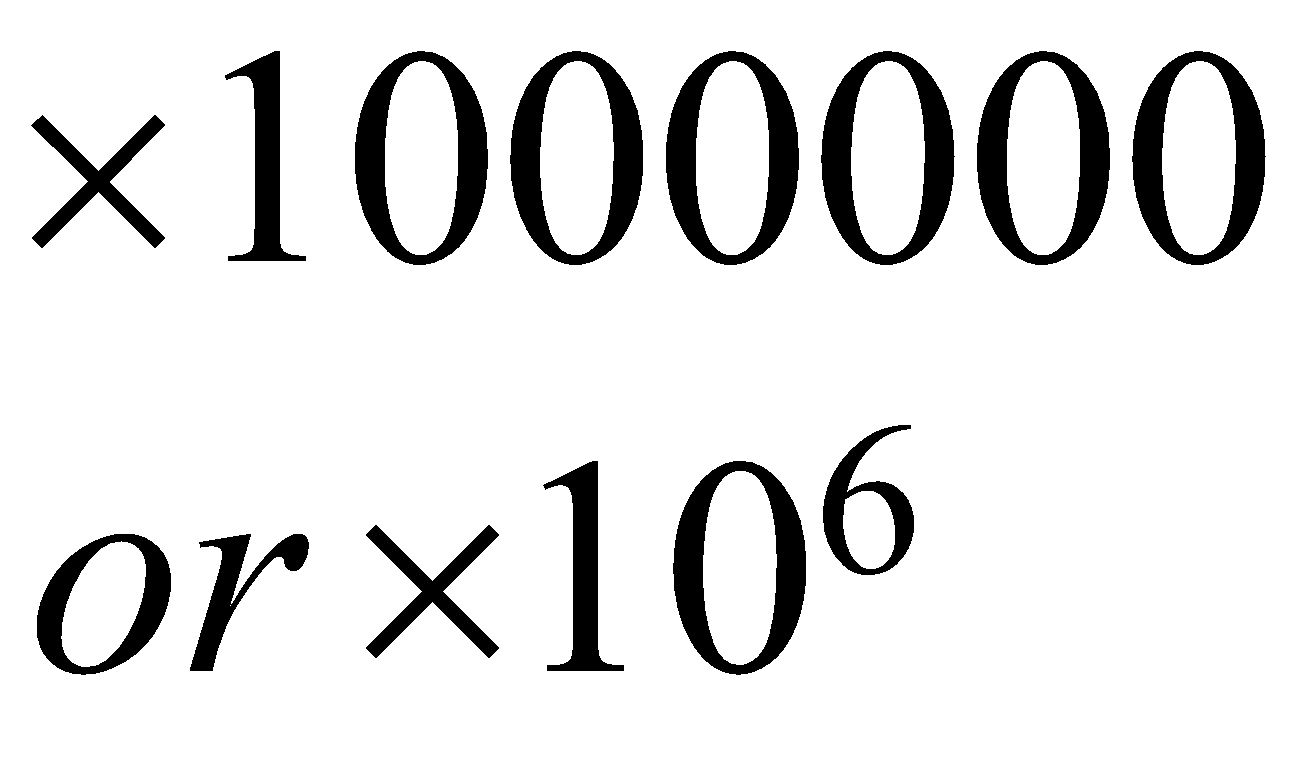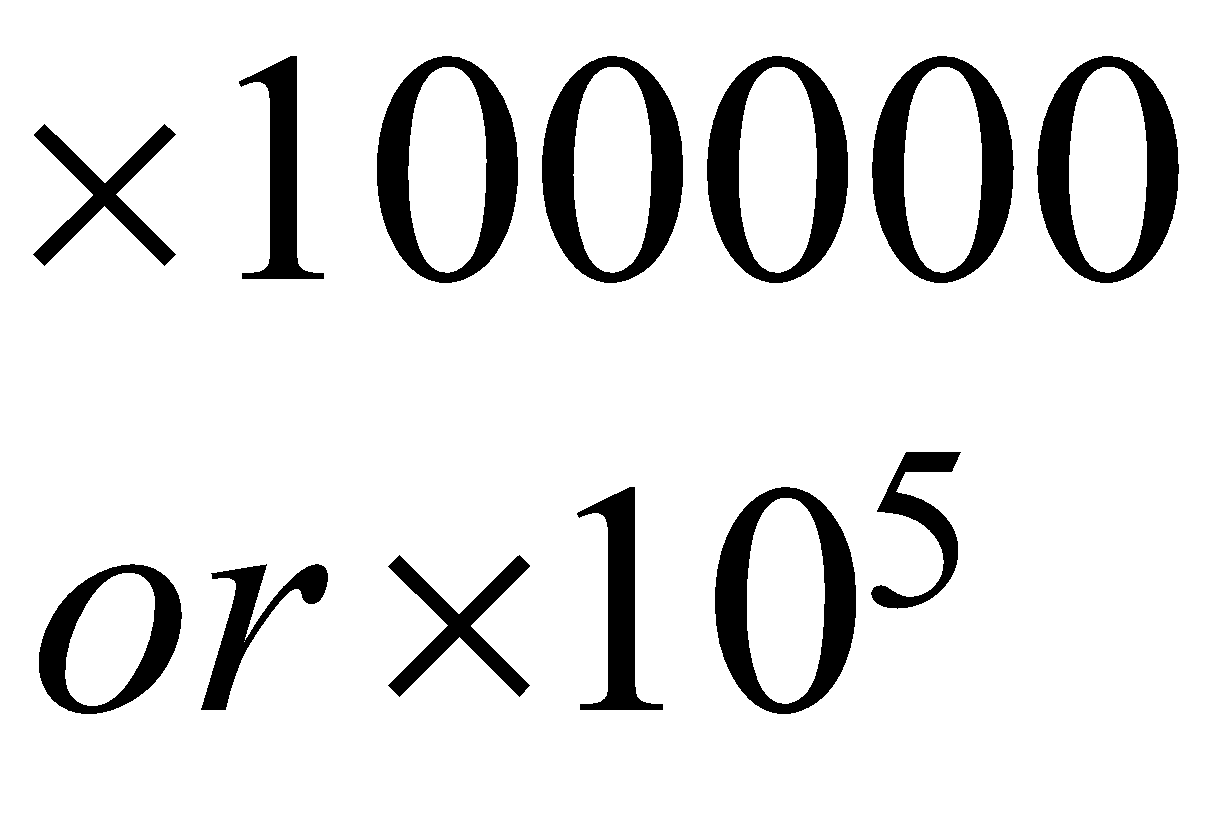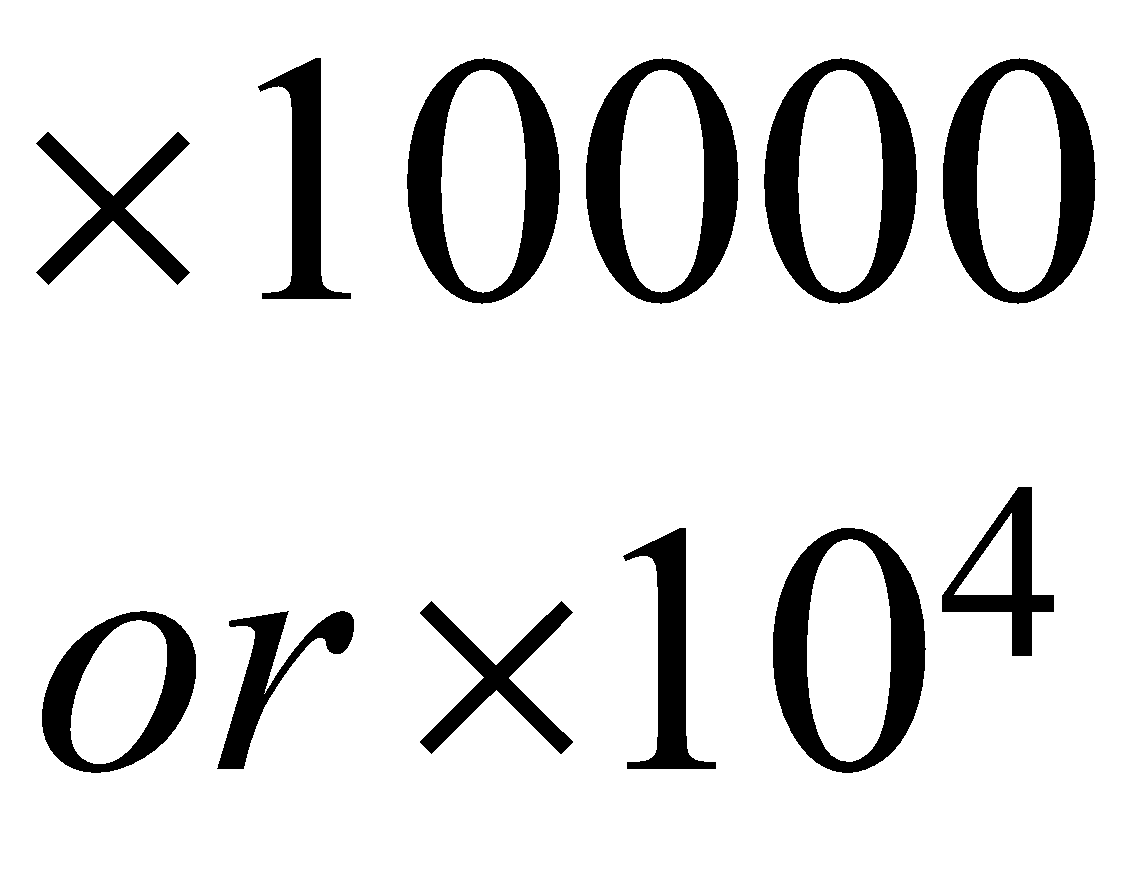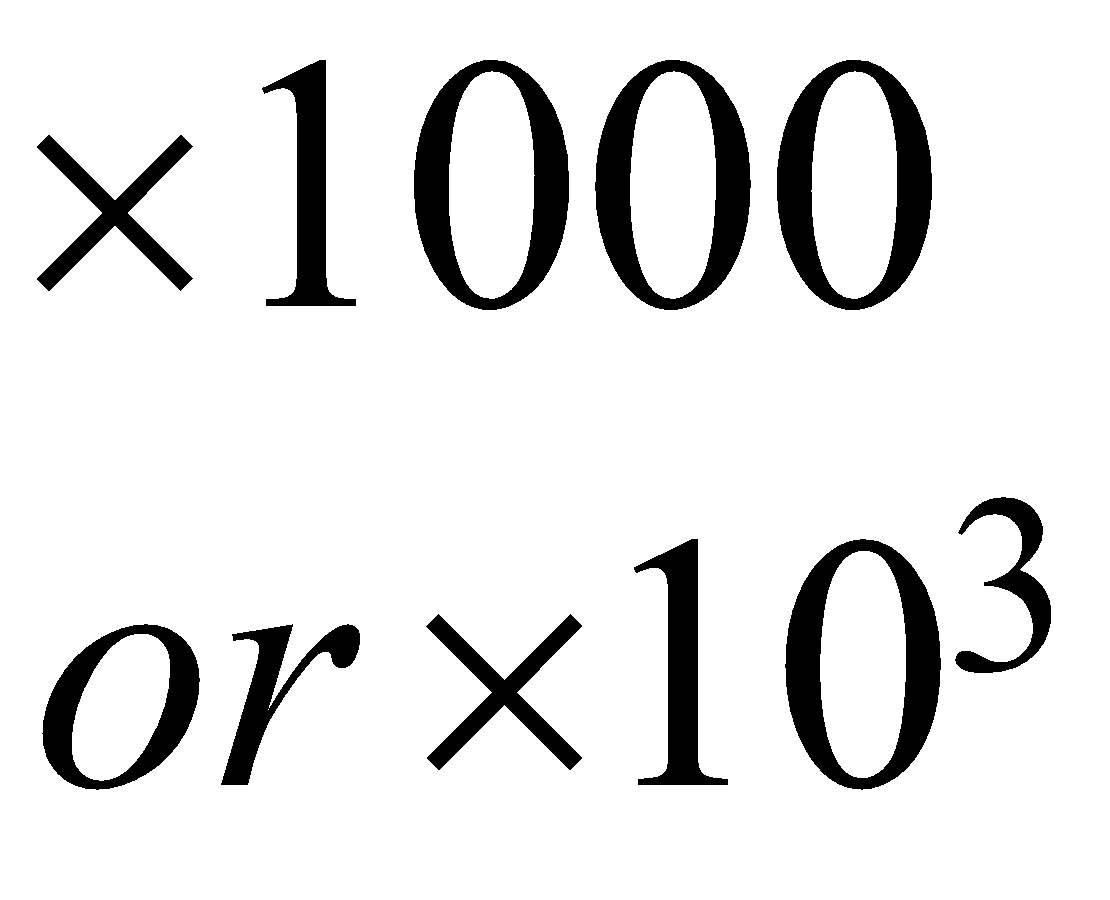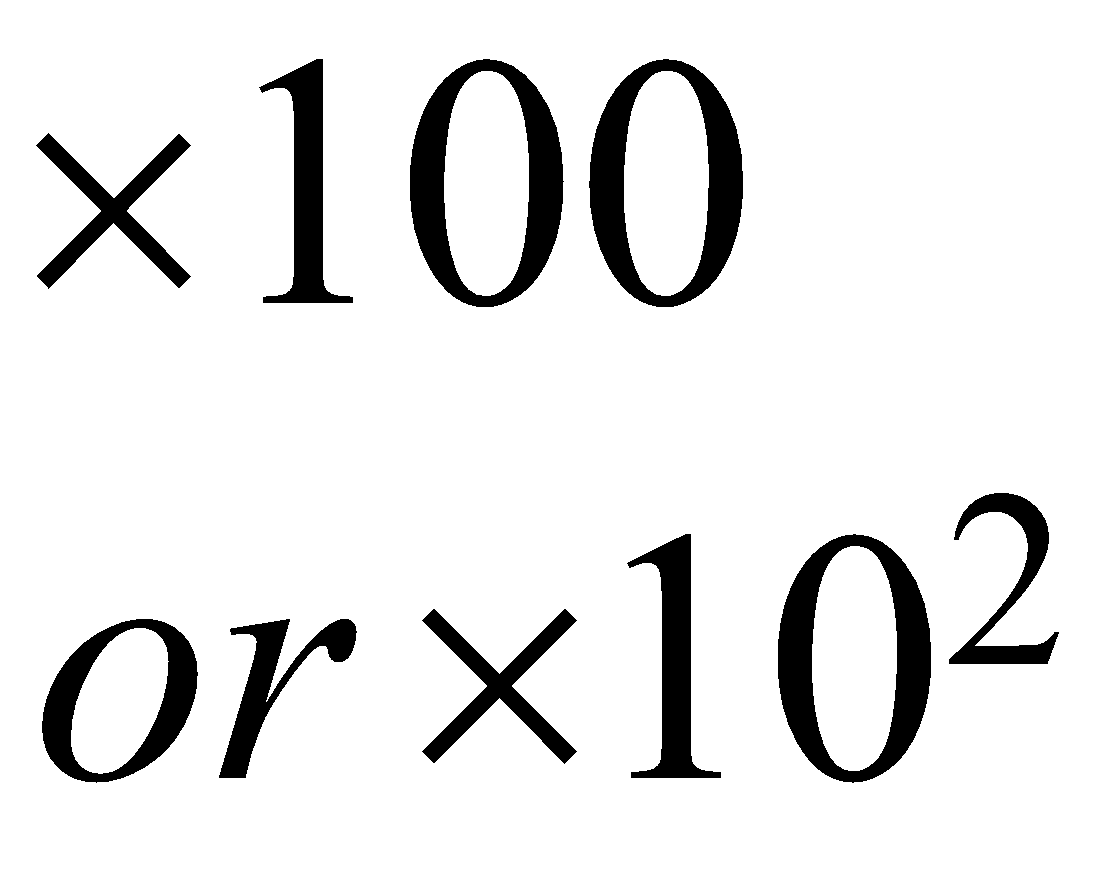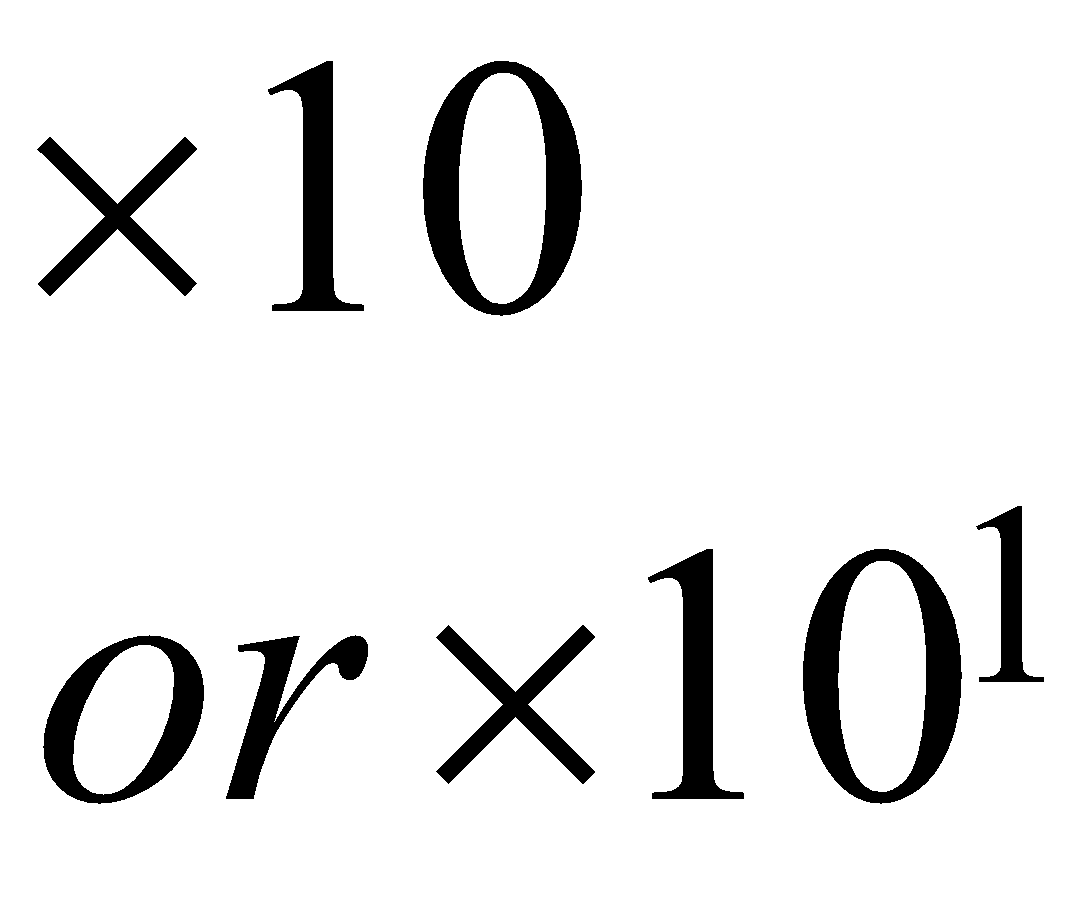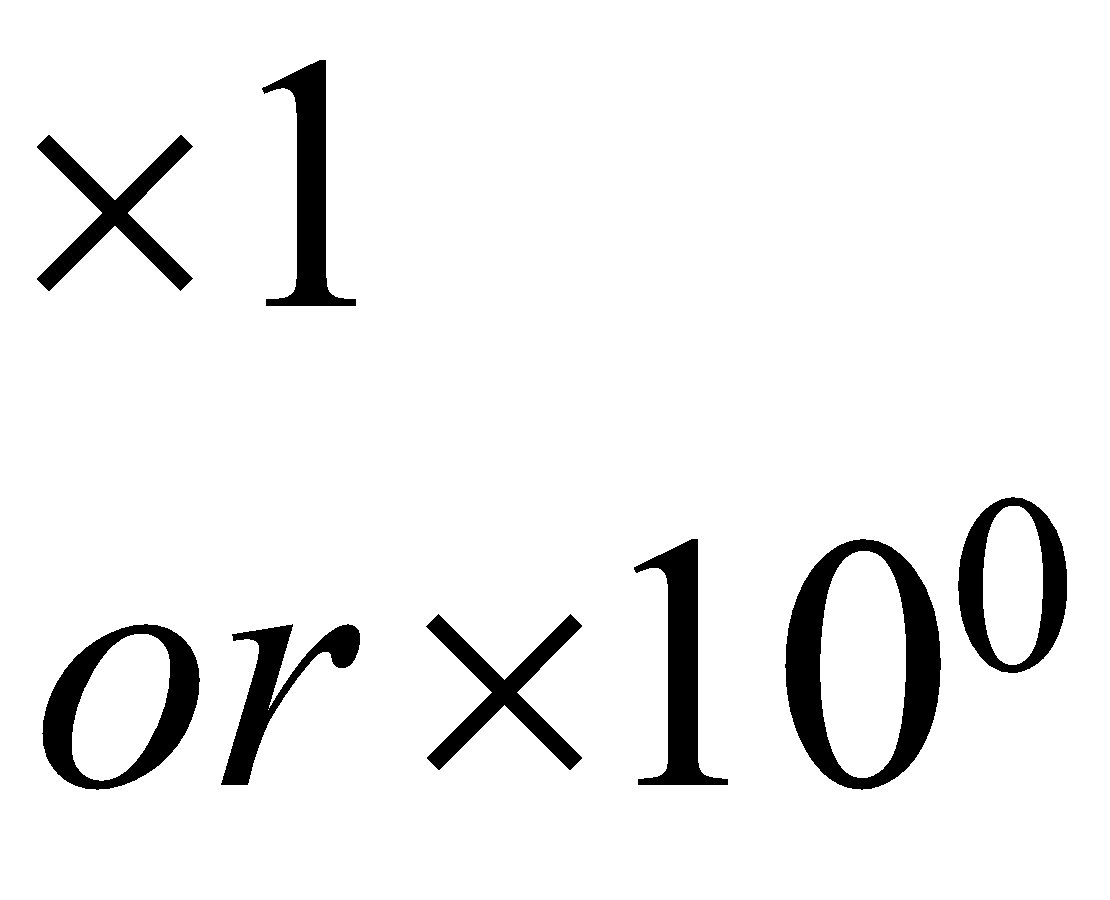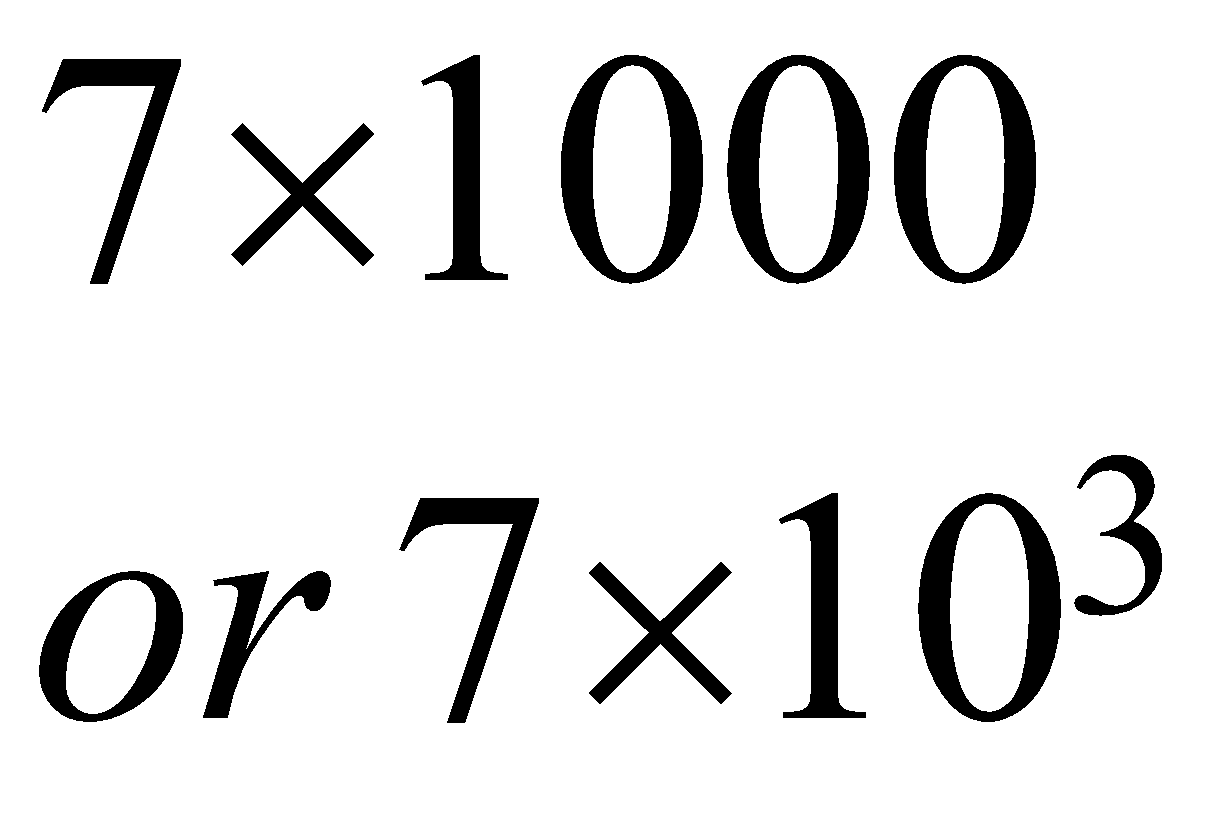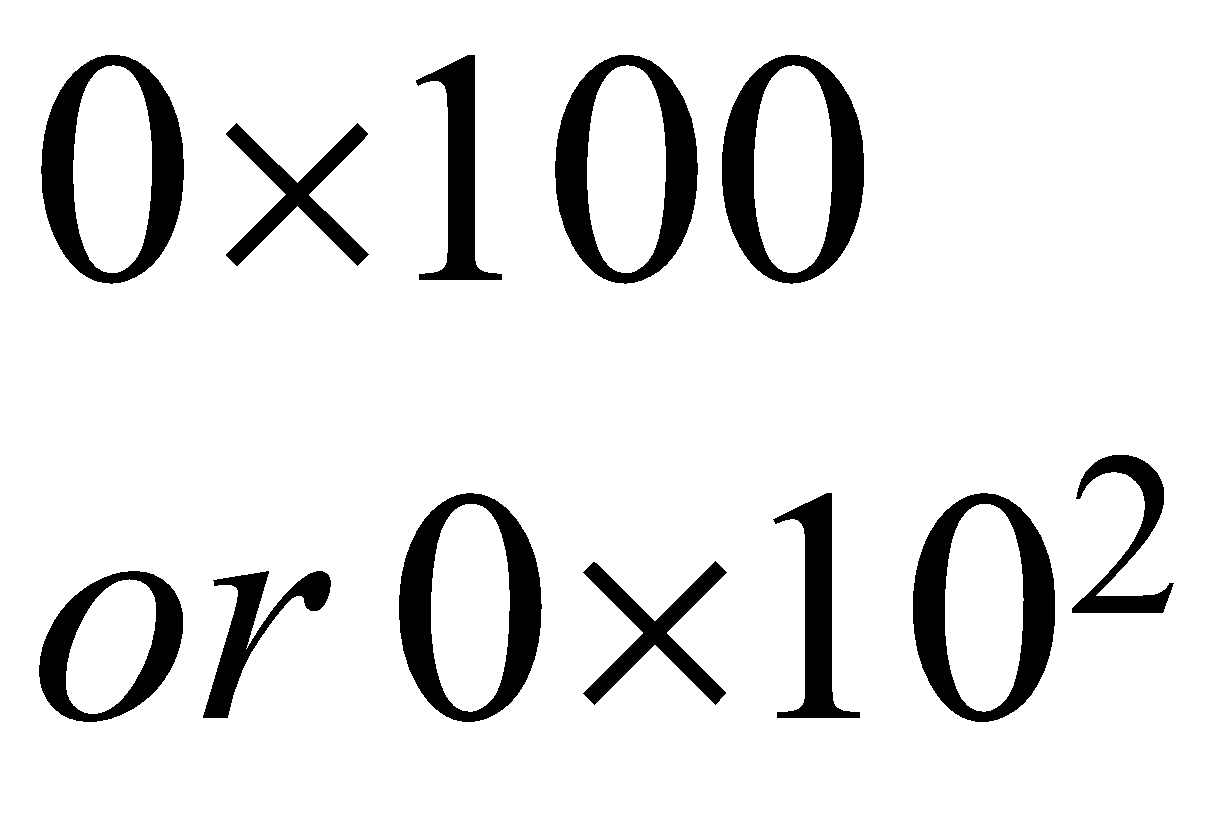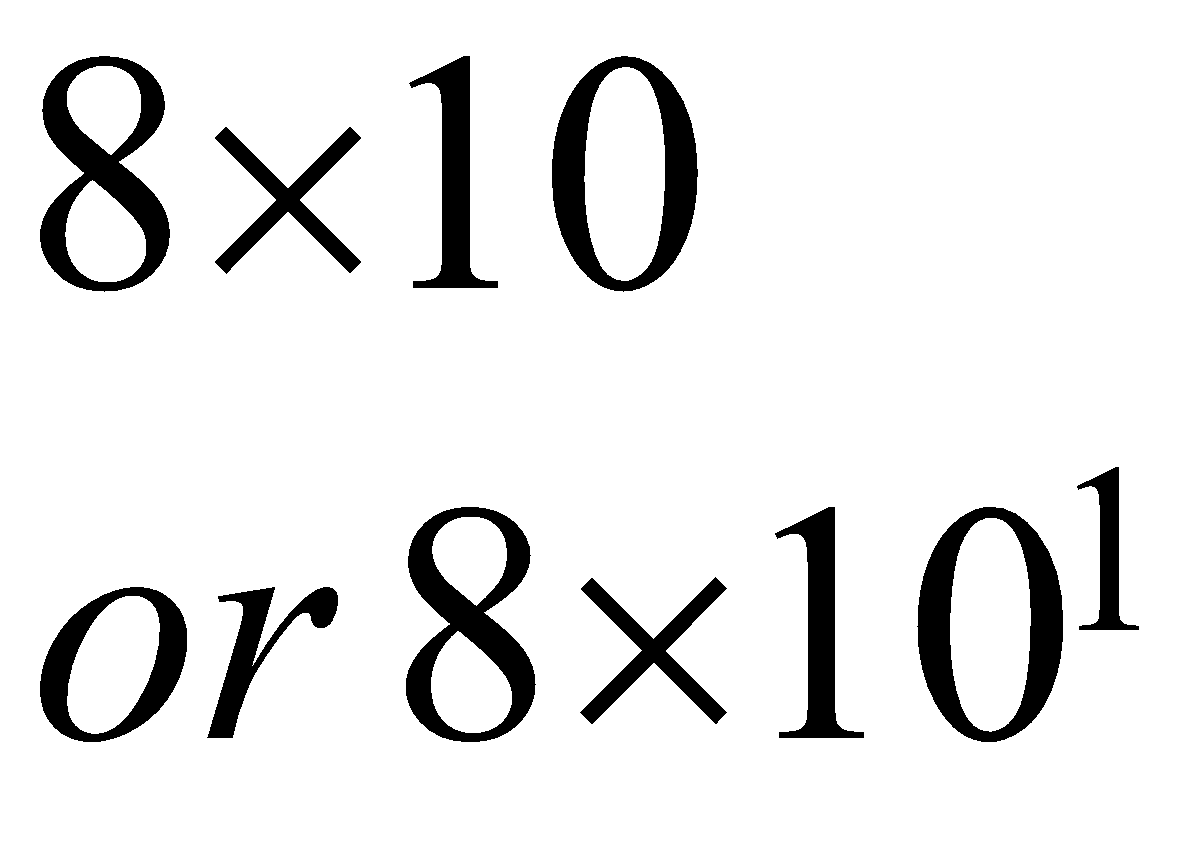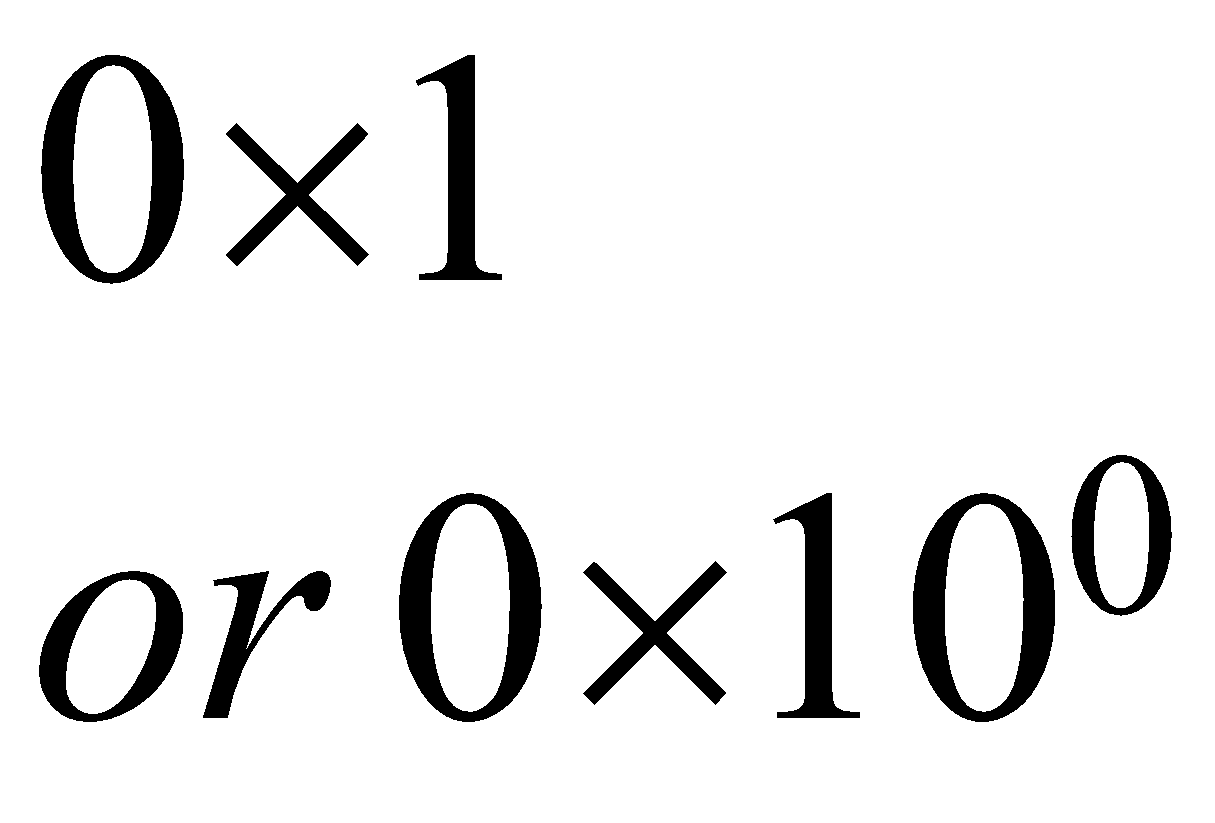7080 in Words
Introduction
To say or write 7080 in words, we have to understand the meaning of 7080 first. At the first glance, 7080 appears to be a number. It is, evidently, true. But by adding some units of measurement (like a milligram, rupees, etc.) to 7080, we can enhance its meaning. The phrases 7080 kg of milk chocolate or 7080 dollars definitely imply some physical quantity Isn’t. 7080 is written as “Seven thousand eighty is written” in word. As such, 7080 is a number consisting of the digits 7, 0, 8, and 0. Every digit has its place value that imparts its unique meaning. Let us dive into it to explore further.
Why is The Decimal Number System Important?
The “decimal number system” allows the numbers to be systematically arranged, engaging the indices of 10 to indicate the place values of the constituent digits of every number.
Once the unique position or place value of each constituting digit of the number in the decimal number system is clear, the number can be, easily translated into words.
The right-hand most digit of a number is taken as the reference to designate the place value of each digit of the number. Here, the place value increases as “powers of 10” as you shift towards the left side from the right-most digit of the number.
Digit’s place | Ten lakh | Lakh | Ten thousand | Thousand | Hundred | Ten’s | Unit or One’s |
Place value | \begin{align} & \times 1000000 \\ & or\ \times {{10}^{6}} \end{align}\
| \begin{align} & \times 100000 \\ & or\ \times {{10}^{5}} \end{align}\
| \begin{align} & \times 10000 \\ & or\ \times {{10}^{4}} \end{align}\
| \begin{align} & \times 1000 \\ & or\ \times {{10}^{3}} \end{align}\
| \begin{align} & \times 100 \\ & or\ \times {{10}^{2}} \end{align}\
| \begin{align} & \times 10 \\ & or\ \times {{10}^{1}} \end{align}\
| \begin{align} & \times 1 \\ & or\ \times {{10}^{0}} \end{align}\
|
How do you say 7080 in Words?
It is clear that 7080 does not have any unit of measurement with it, so 7080 is a number.
You see that the number 7080 consists of 5 digits.
Now, you have the following observations for the place values of the digits in the number 7080.
The digit in the right-hand most position in the number 7080 is 0, which is its “unit’s place”.
The digit towards the immediate left-hand side from the unit’s place in the number 7080 is also 8, which is its “ten’s place”.
Similarly, the “hundred’s place” and the “thousand’s place” in the number 7080 are 0 and 7 respectively.
Digit’s Place | Thousand | Hundred | Ten’s | Unit or One’s |
Digit |
|
|
|
|
place value | \begin{align} & 7\times 1000 \\ & or\ 7\times {{10}^{3}} \end{align}\
| \begin{align} & 0\times 100 \\ & or\ 0\times {{10}^{2}} \end{align}\
| \begin{align} & 8\times 10 \\ & or\ 8\times {{10}^{1}} \end{align}\
| \begin{align} & 0\times 1 \\ & or\ 0\times {{10}^{0}} \end{align}\
|
Mathematically you can expand the following
\begin{align}
& 7\times {{10}^{3}}+0\times {{10}^{2}}+8\times {{10}^{1}}+0\times {{10}^{0}} \\
& =7000+0+80+0 \\
& =7080 \\
\end{align}\
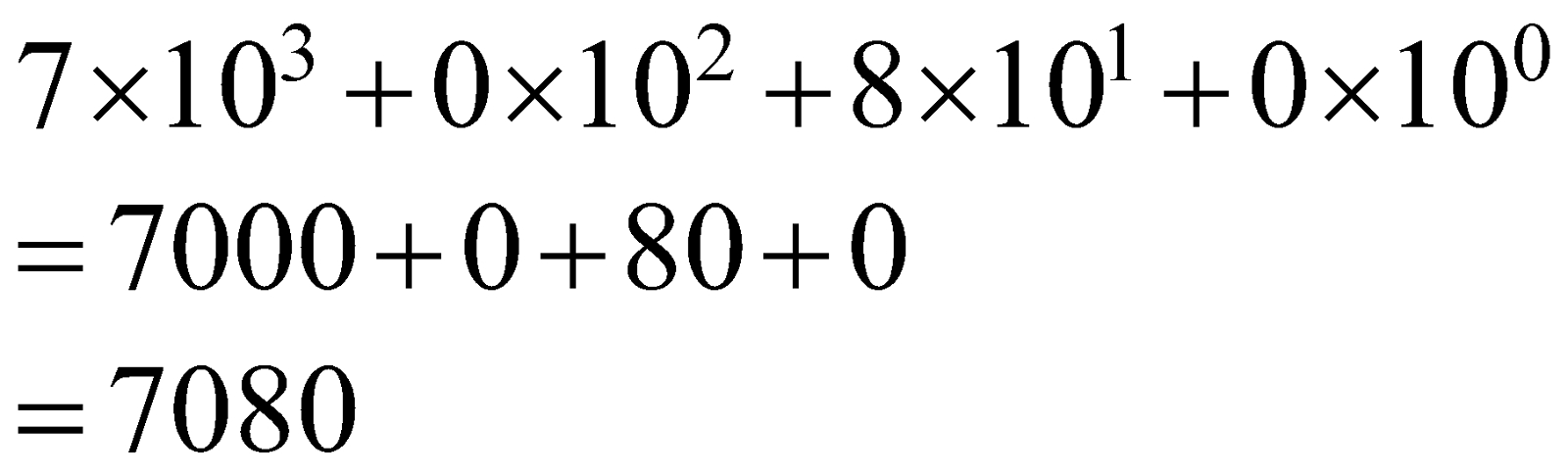
Here, 7080 has only two non-zero digits 7 and 8, in its thousand’s place and ten’s place respectively; while all the digits are zero in its hundred’s place and unit’s place.
Therefore, 7080 is written as “Seven Thousand Eighty in words”.
How do you write 7080 Rupees in Words?
The term 7080 rupees contains the number 7080 followed by the unit rupees.
You can easily express 7080 rupees in words, by writing the number 7080 in words and placing the unit “rupees” after it.
So, you can write “7080 rupees” as “Seven Thousand and Eighty Rupees” in words.
Is 7080 an Even or an Odd Number?
You can define a number as an “even number” only when the number leaves no remainder on being divided by 2.
On the other hand, the number that is not completely divisible by 2 but leaves a remainder is defined as an “odd number”.
Note that you get no remainder when you divide the number 7080 by 2. So, you can infer that it is an even number.
\begin{align}
& 7080\div 2 \\
& =3540 \\
\end{align}\
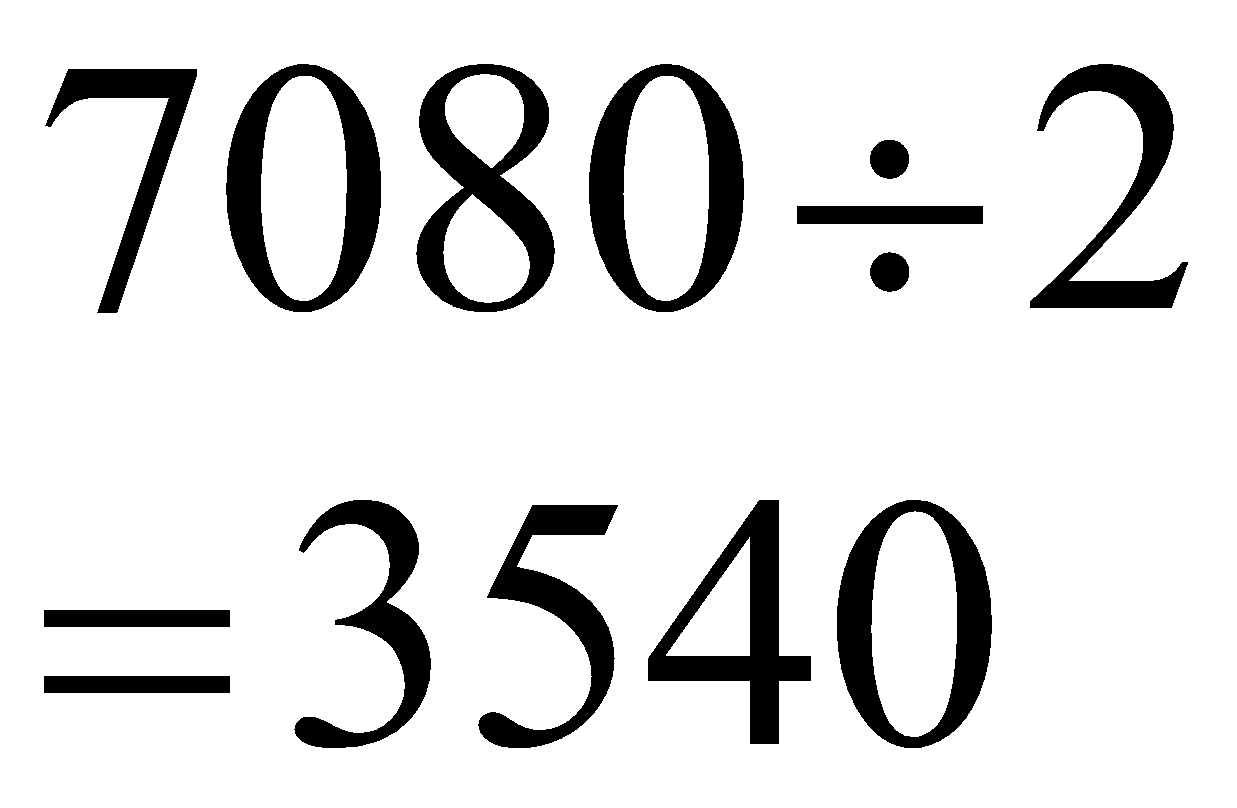
Is 7080 a perfect square number?
When you multiply any number by itself for a single time, the resulting product becomes a “perfect square”. Thus, any perfect square number can be looked upon as the product of two equal integers
\begin{align}
& 7080 \\
& ={{2}^{3}}\times 3\times 5\times 59 \\
\end{align}\
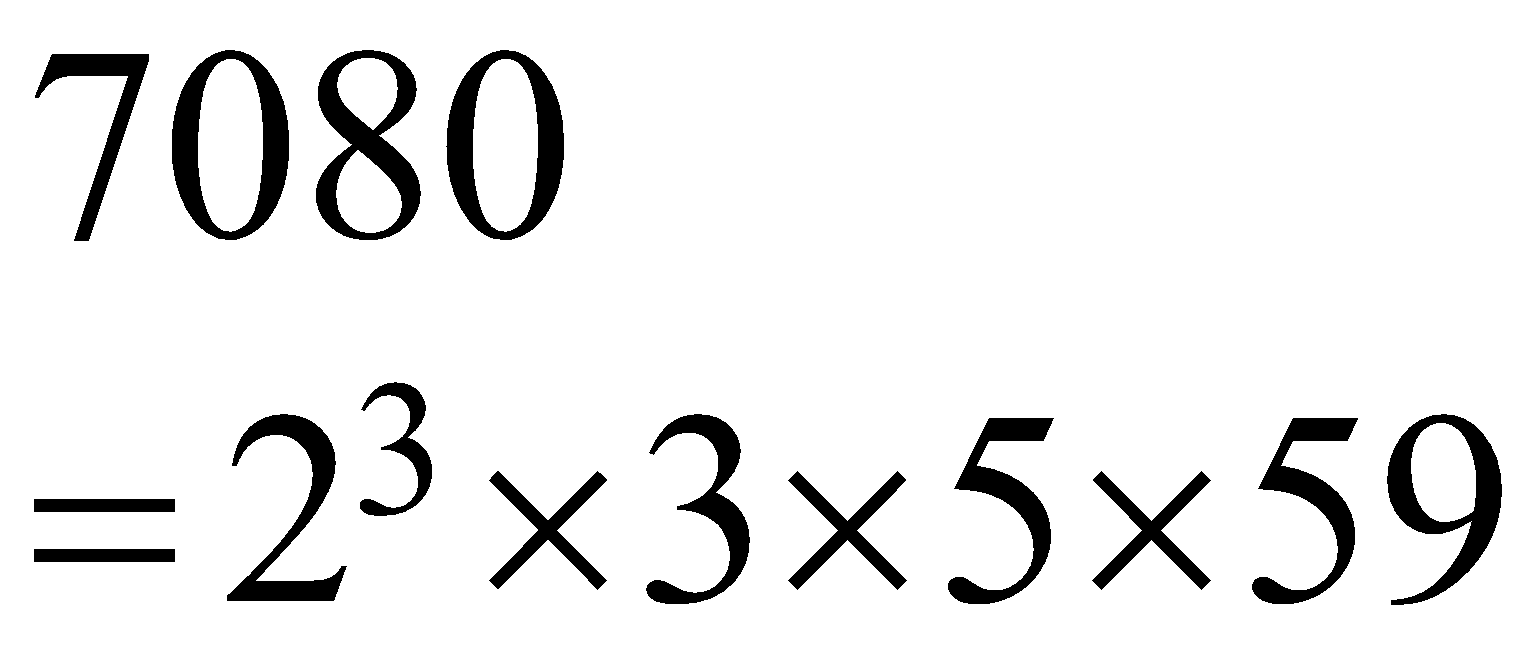
Here, 7080 cannot be expressed as the product of two equal real integers. Hence, you get that 7080 is not a perfect square.
Is 7080 a Perfect Cube Number?
On multiplying any number by itself twice, the resulting product becomes a “perfect cube”. Thus, any perfect cube number can be reproduced as the product of three equal integers
\begin{align}
& 7080 \\
& ={{2}^{3}}\times 3\times 5\times 59 \\
\end{align}\
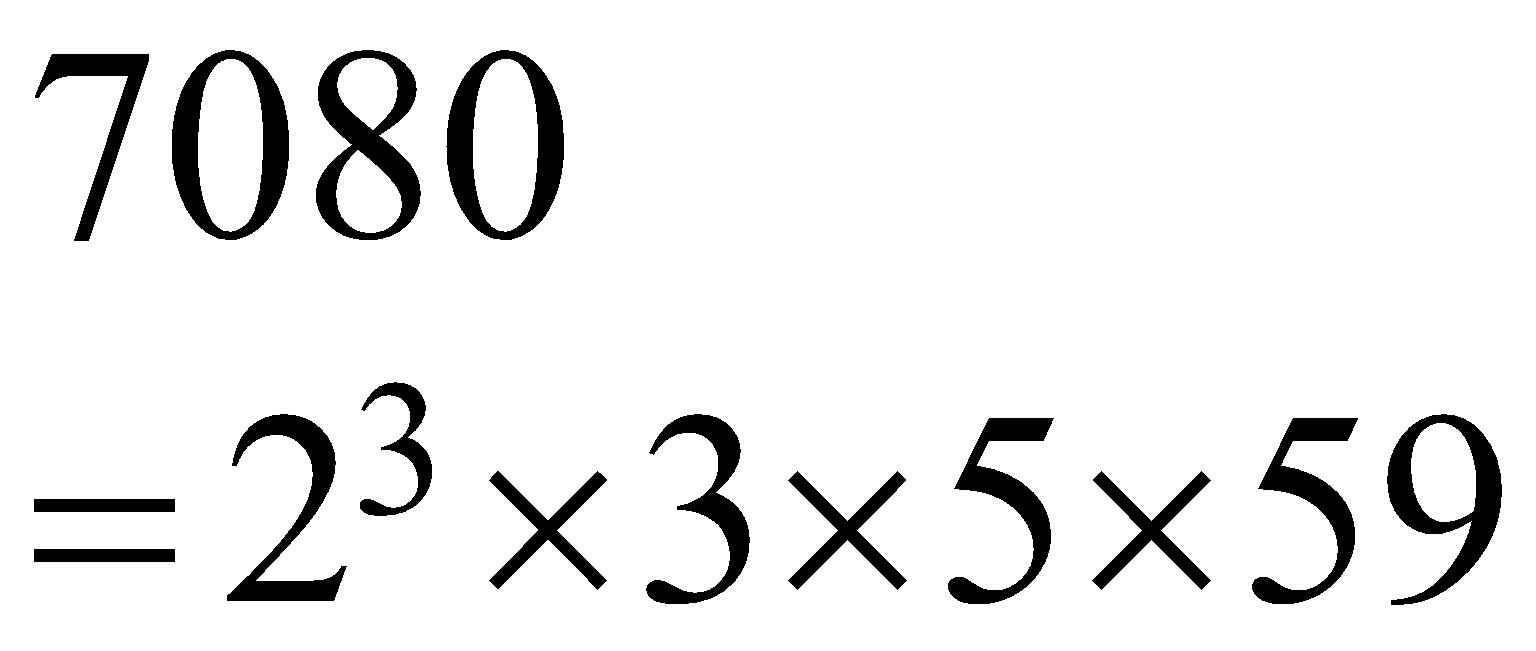
Here, 7080 cannot be represented as the product of three equal integers. Hence, 7080 is not a perfect cube.
Is The Number 7080 Composite or Prime?
When you factorize any number and find that it has only two factors (1 and the number itself), it is known as a “prime number”.
On the other hand, any number that has more than two factors is called a “composite number”.
\begin{align}
& \text{Factors of 70}80 \\
& =1,\ 2,\ 3,\ 4,\ 5,\ 10,15,\ldots ,7080 \\
\end{align}\
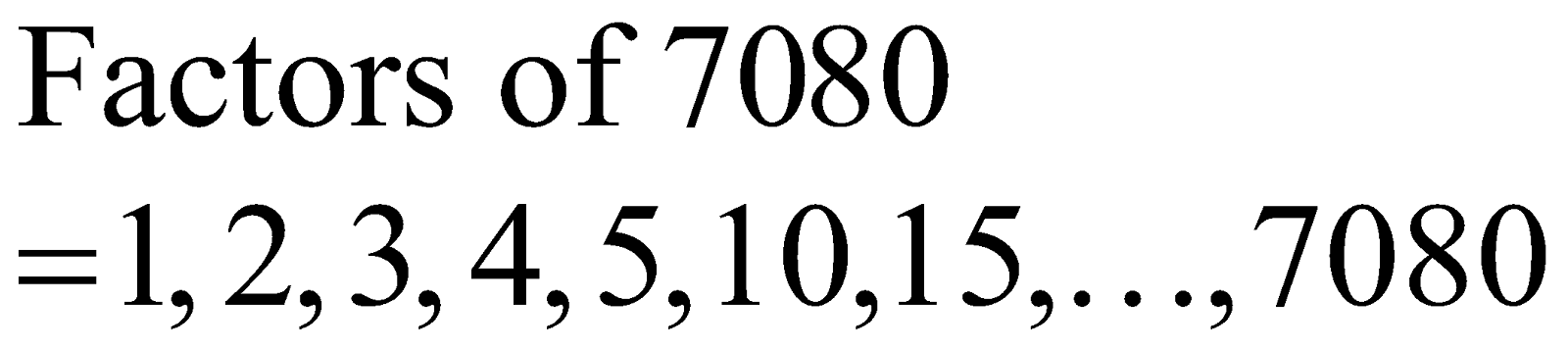
Here the number 7080 has more than two factors. Thus, you gather that 7080 is not a prime number, but rather a composite number.
You can find out the detailed othr number in words article list below:-
Frequently Asked Questions (FAQs)
Yes, the number 7080 falls under the category of an “integer “as it lies in the number line under the set of integers.
\begin{align}
& Z\text{ (integers)} \\
& =\left\{ -\infty ,\ \ldots ,\ -3,\ -2,\ -1,\ 0,\ 1,\ 2,\ 3,\ \ldots +\infty \right\} \\
\end{align}\
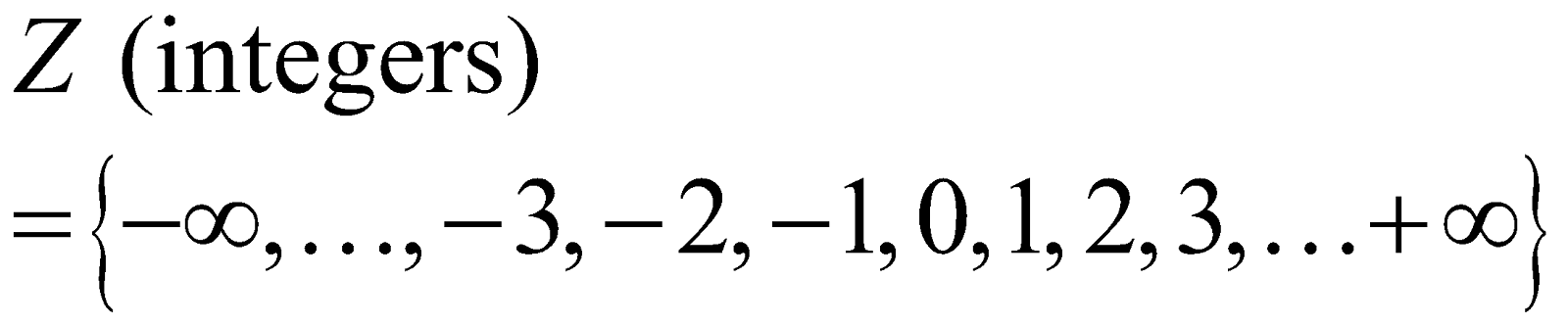
Basically the digits are arranged in any number such that every position has its unique “place value”. When you obtain the products for each of its constituent digits with its corresponding place value and add them up, you get the number. Below are a few examples.
\begin{align}
& \text{eight}={{10}^{0}}\times 8 \\
& =1\times 8 \\
& =8 \\
& \text{eighteen}={{10}^{1}}\times 1+{{10}^{0}}\times 8 \\
& =10\times 1+1\times 8 \\
& =18 \\
& \text{one hundred and eight}={{10}^{2}}\times 1+{{10}^{1}}\times 8+{{10}^{0}}\times 0 \\
& =100\times 1+10\times 8+1\times 0 \\
& =180
\end{align}\

The number 7080 is a natural number as it is grouped in the set of “natural numbers” in the number line.
\begin{align}
& N\text{ (natural numbers)} \\
& =\left\{ \ 1,\ 2,\ 3,\ \ldots +\infty \right\} \\
\end{align}\
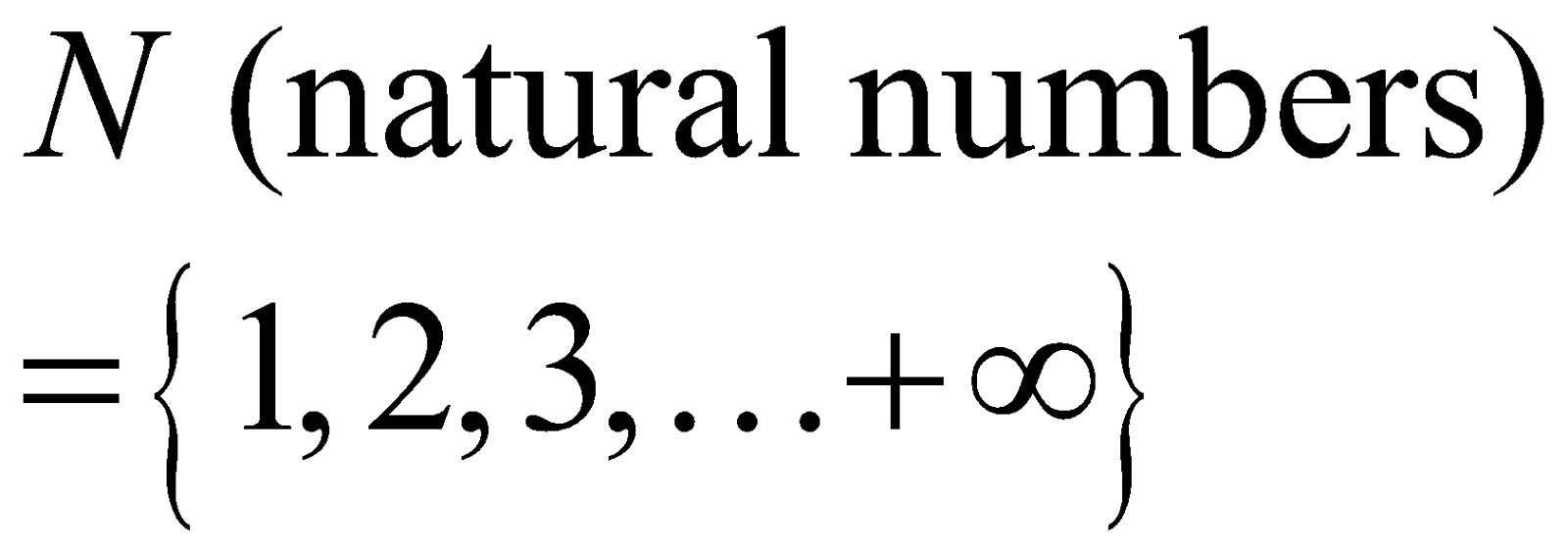
A “complex number” has a real part and the imaginary part. Yes, you can definitely, express 7080 as a complex number with its real part as 7080 and it's imaginary part as 0.
\begin{align}
& 7080 \\
& =7080+i0 \\
\end{align}\
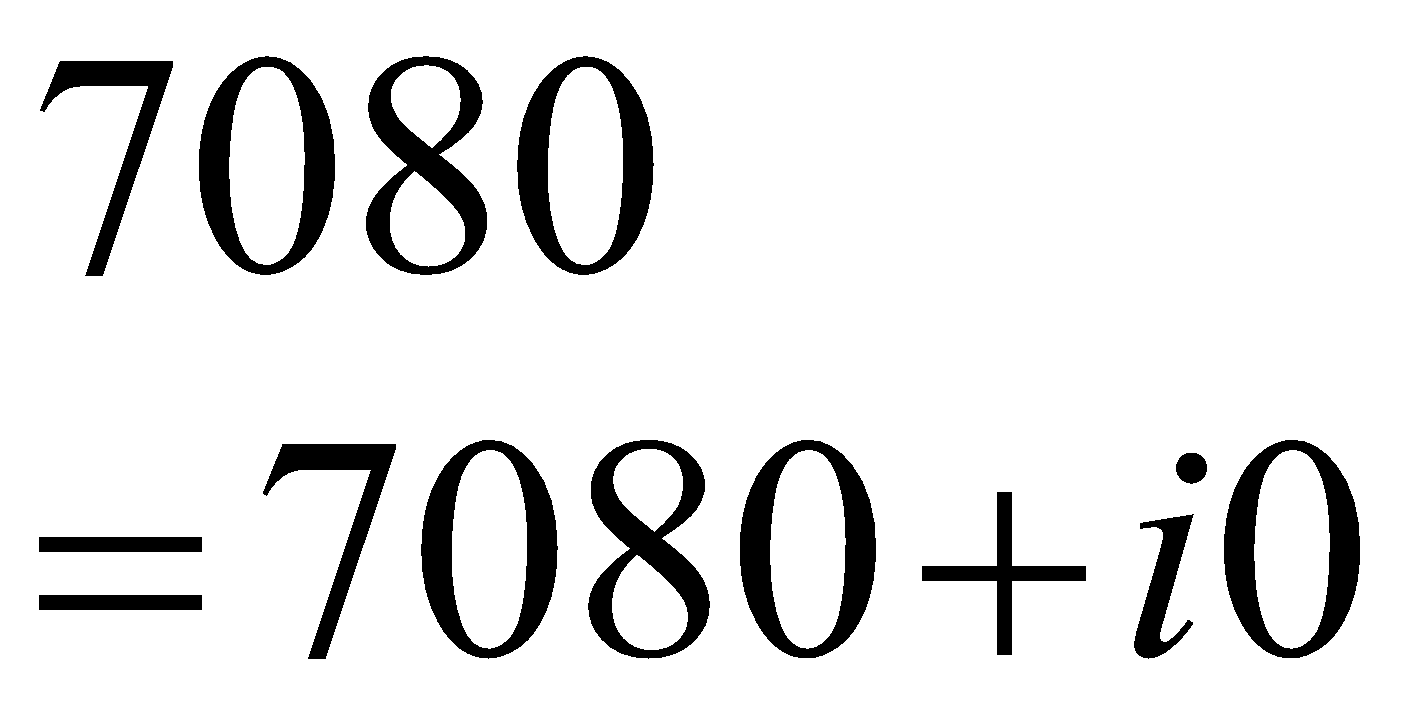
Here you can express 7080 in the form of p/q, where p is any integer and q is, strictly, a non-zero integer. Therefore, the number 7080 is a “rational number”.
\begin{align}
& 7080 \\
& =\frac{7080}{1} \\
& =\frac{p}{q};\text{ where }p=7080,q=1\ne 0 \\
\end{align}\
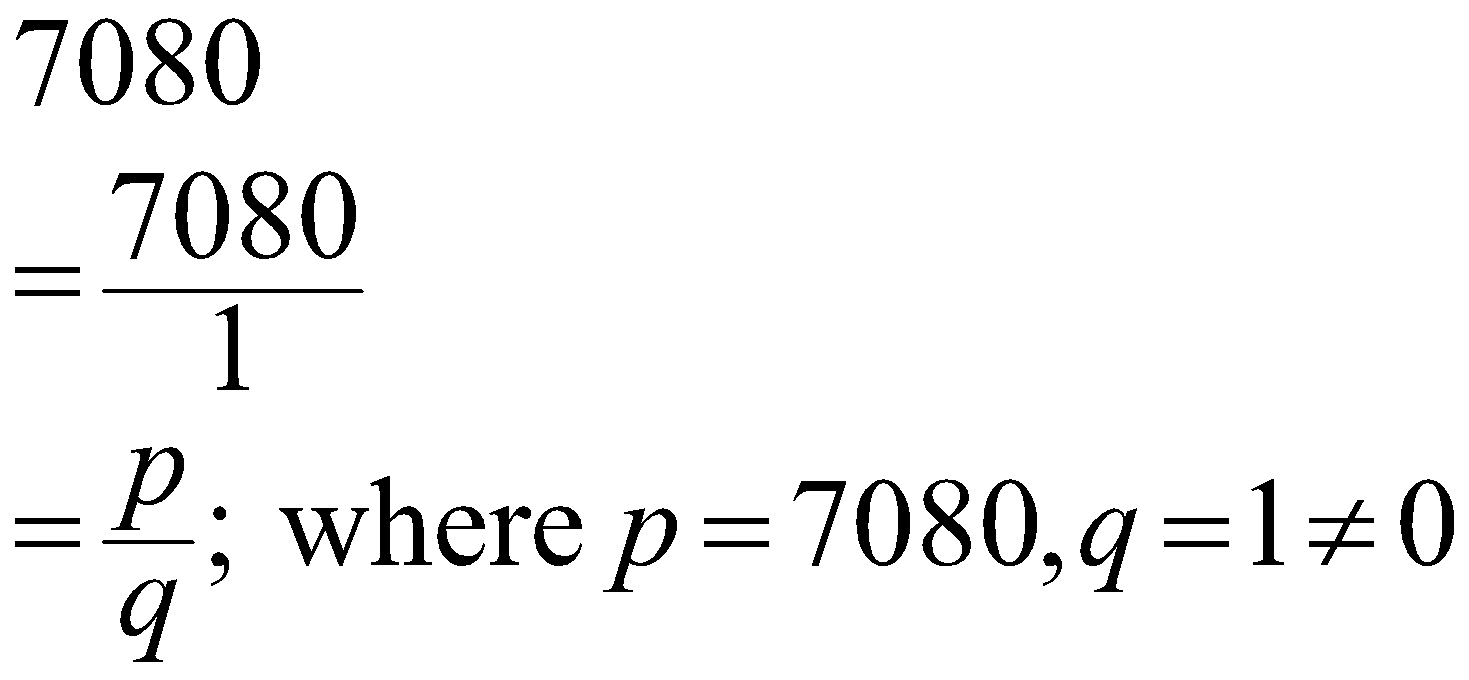
Applications for Admissions are open.
This ebook serves as a valuable study guide for NEET 2025 exam.
NEET Previous 10 Year Questions
Get nowThis e-book offers NEET PYQ and serves as an indispensable NEET study material.
JEE Main Important Physics formulas
Get nowAs per latest syllabus. Physics formulas, equations, & laws of class 11 & 12th chapters
JEE Main Important Chemistry formulas
Get nowAs per latest syllabus. Chemistry formulas, equations, & laws of class 11 & 12th chapters
JEE Main high scoring chapters and topics
Get nowAs per latest 2024 syllabus. Study 40% syllabus and score upto 100% marks in JEE
JEE Main Important Mathematics Formulas
Get nowAs per latest syllabus. Maths formulas, equations, & theorems of class 11 & 12th chapters