How Many Minutes in 1 Degree

Introduction

The relation between degree and minute is 1 degree = 60 minutes. Time and angular conventions, together with the relationships between them, were first developed using the Babylonian sexagesimal number system, which had a base of 60. It is necessary to take the earth's rotation into account, as well as how long it takes for one rotation. To calculate the relationship between degrees and minutes, the idea of standard time conversions and the partition of a circle by degrees-measured angles are both utilized.

Complete Step By Step Process

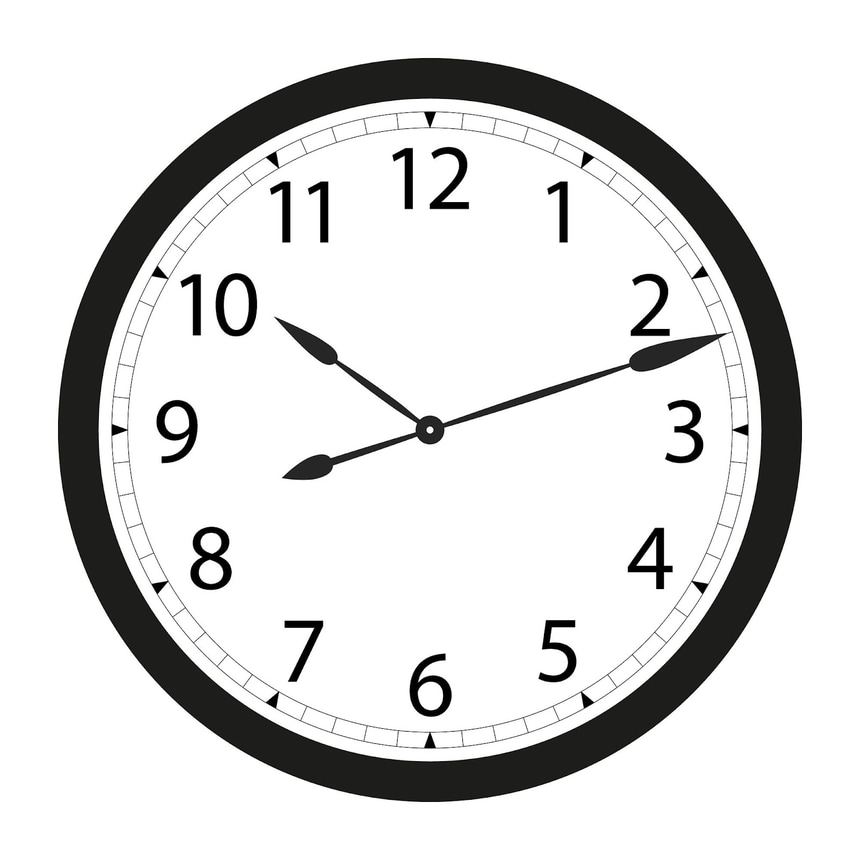
Our regular use of clocks has a lot of significance. The idea that because the clock is fashioned like a circle, the circle is divided into a number of equal parts or sections, with which we compute the hour, minutes, and seconds, is the basis for the hours, minutes, and seconds hands of the clock.

The arc of the circle, or one hour, was divided into 60 equal pieces since the clock is considered to represent a full circle, allowing minutes to be measured. Similar divisions were made to convert minutes into seconds, which are divided into 60 further equal parts. In this way, a link between the quantities of an hour, minute, and second was created. The following time conventions applied:

1hr=60min and 1min=60sec

Similar to how it was demonstrated that there is a relationship between degrees and minutes, the DMS notation, which stands for degree-minute-seconds, was used to illustrate this. Many conversions and calculations used this notation. Minutes and degrees were considered to be related, much as there was a relationship between hours, minutes, and seconds with each other.

The idea of the Earth's revolution must now be taken into account to establish the relationship between degrees and minute amounts. The fact that the Earth rotates around the sun is well known to us, and as a result, the time of day varies. Additionally, we are aware that a whole rotation of the Earth takes 24 hours.

1 day =24 hours

We can conclude that a 360° arc needs to be formed to create a complete circle by using the geometry of the clock, which is stated to have a circular shape. Now, we'll solely think about the clock's hour hand. We are aware that a day begins at midnight on the hour. If we assume that the hour hand begins at 12 a.m., or the number twelve on the clock, we may state that the hour hand of the clock is said to travel or complete sweeping across the entire arc of the circle two times in 24 hours, which is considered to constitute one day.

Once at 12 o'clock noon and once at 12 o'clock at midnight, respectively, to end the current day and begin the next. The hour hand is supposed to trace the clock's 360-degree arc twice, which indicates that it completes two circles in a day, hence the multiplication of:

\begin{equation}

2\times 360

\end{equation}

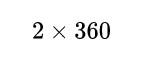
A clock has 12 divisions, so for each division, we obtain the following:

\begin{equation}

1^0= (2\times 360)/12

\end{equation}

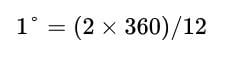
The result of solving this expression is:

∴1° = 60 minutes

Examples

1. How many minutes in 5 degrees?

Solution:

Since, 1° = 60 minutes

Then, 5° = 5 ✕ 60 minutes = 300 minutes

2. How many minutes in 12 degrees?

Solution:

Since, 1° = 60 minutes

Then, 12° = 12 ✕ 60 minutes = 720 minutes

3. How many degrees in 120 minutes?

Solution:

Since, 60 minutes = 1 degree

Then, 120 minutes = \begin{equation} 120 \div 60 = 2 \end{equation}

degrees

Conclusion

It's a common myth that 1 degree equals 4 minutes instead of 60 minutes, but this is untrue. This is due to calculations showing that it takes the Earth about 4 minutes to rotate an angle of 1 degree when taking into account its 360-degree rotation. As a result, the relationship that 1 degree = 60 minutes remains unaffected and is thus demonstrated to be accurate.

To calculate the angle of the earth's rotation and the time taken for rotation, we can also use minutes

To rotate 360°, the earth takes around 24 hours

Or to rotate 360° ,earth takes around

\begin{equation}

(24 \times 60)

\end{equation}

minutes = 1440 minutes

To rotate 1°, earth takes

\begin{equation}

(1440 \div 360)

\end{equation}

= 4 minutes

That means the angle of 1° rotation of the earth is not equal to 4 minutes but the earth takes 4 minutes to rotate 1°.