How Many Faces Does a Cuboid Have

A cuboid has six faces. A cuboid is a three-dimensional solid object with eight vertices, six faces, and twelve edges, each rectangular. All of the angles that are produced in a cuboid are right angles. A cuboid has three dimensions: length, breadth, and height. All angles and opposite faces of a cuboid in a rectangular shape are right angles and equal. These right angles make a right rectangular prism by definition. This polyhedron is referred to as a rectangular parallelepiped or an orthogonal parallelepiped. Numerous items in daily life have cuboid shapes. Boxes made of cardboard (or other containers), bricks, books, Rubik's cubes, wooden beams, six-sided dice, and various structures are all examples of cuboids. The only difference between a cuboid and a cube is that a cube's sides are all the same length.

Number of Faces of a Cuboid

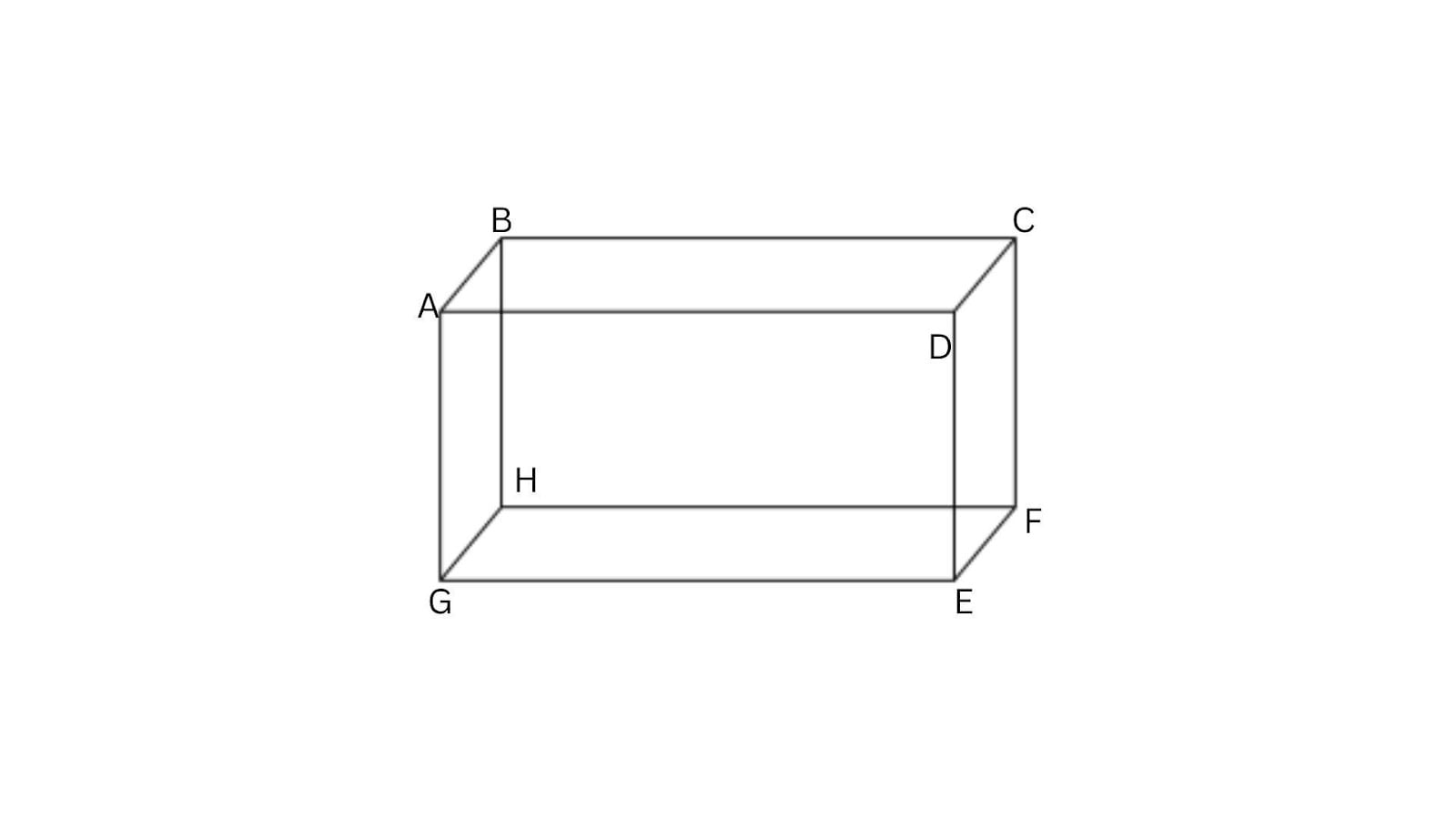
A cuboid is a three-dimensional form with flat, rectangular faces that are parallel to one another but not each of them has the same size. Now, every angle is a right angle, and every face is a rectangle.

Let's first examine the cuboid's appearance before determining how many vertices, faces, and edges it has:

We'll now count the number of faces in a cuboid. Each of the six rectangles that make up a cuboid's face is referred to as a "face." The six faces of the cuboid in the above diagram are ABCD, ABHG, BCFH, HFEG, DCEF and ADEG. A pair of opposing faces are formed by the bottom face EFGH and the top face ABCD.

Between any two adjacent vertices, a line segment constitutes the cuboid's edge. The opposite sides of the rectangle above are equal, and there are 12 edges: AB, AE, AD, HD, HE, HG, GC, GF, FE, FB, EF, and CD. Consequently, AH=DH=FC=DG, EH=FG=AB=CD, and AD=GH=BC=EF.

Now that we are aware, we may name the intersection of a cuboid's three edges—or vertex—a cuboid. The given cuboid contains 8 vertices, which correspond to the cuboid's vertices in the given figure: A, B, C, D, E, F, G, and H, with 8 total vertices, 12 total edges, and 6 total faces.

Conclusion

A cuboid has six faces; all the angles formed are right angles. Three dimensions make up a cuboid: length, breadth, and height. A rectangular cuboid has right angles and equal angles on all of its opposite faces. Everyday objects frequently have cuboid forms. The length of each side of a cube is the only distinction between it and a cuboid. The volume of a cuboid is calculated using the formula V=LxWxH, which stands for length times width times height. A cuboid is a three-dimensional object with parallel, flat, rectangular faces that are not all the same size.

Right angles now characterize all angles, and rectangles make up all faces. A cuboid's face is made up of six rectangles, each of which is referred to as a "face." The cuboid's edge is any line segment connecting two nearby vertices. The cuboid in the above figure has eight vertices: A, B, C, D, E, F, G, and H on the given cuboid, with a total of 6 faces, 12 edges, and 8 vertices.