How Many Edges are in Cube

Introduction

A cube has 12 edges.

A cube is a 3-dimensional shape with equal sides, like a square. Like every 3D(three-dimensional) shape, cubes also have faces, edges, and vertices. These are the basic properties of a 3-dimensional shape.There are plenty of things around us which indicate the shape of the cube. Such as ice cubes, Rubik's cubes, boxes, dice, etc.

Vertices

The point at which two or more edges of a solid shape meets is a vertex. These are the corner points of any shape. The number of vertices is equal in the case of cubes and Cuboids. The number of vertices in cubes and cuboids is 8.

Faces

The plane surface of the shape is its face. Both cube and cuboid have six faces.

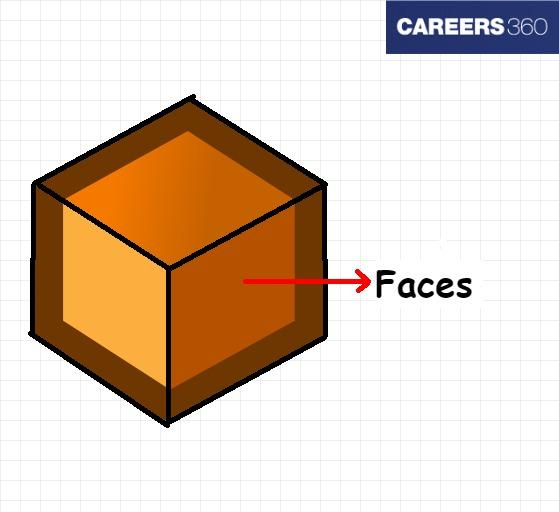
Edges

The line segment, which is the corner of the shape, is its edge. It joins two vertices.

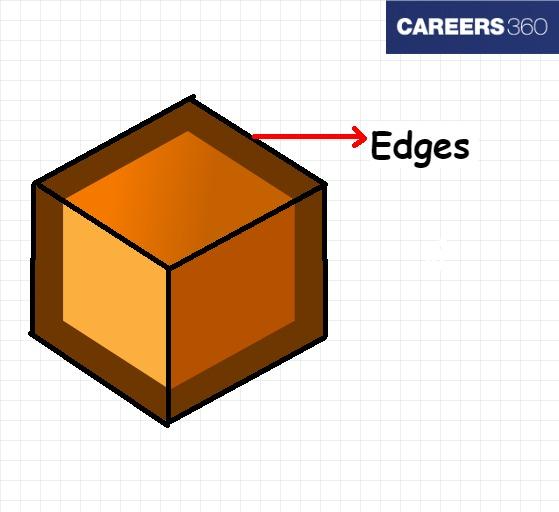
Details About Cube

A cube has six faces, 12 edges, and eight vertices. The solid shape with the same property as the cube is a cuboid, and it also has six faces, 12 edges, and eight vertices. The only difference between the two shapes is the length of their edges. A cube is a 3-dimensional figure of a square, whereas a cuboid is a 3-dimensional figure of a rectangular.

Relation Between Edges, Faces and Vertices

Euler's formula can determine the relation between edges, faces and vertices. This formula can be used for solid shapes which are closed and flat.

\begin{array}{l}

F+V-E = 2 \\

\end{array}

Here,

F = Faces of the cube,

V = Vertices of the cube, and

E = Edges of the cube

Properties of A Cube

• It is a 3-D shape of a square.

• All the faces are equal in a cube.

• The properties of faces, vertices and edges are the same in cubes and cuboids.

• Opposite sides are parallel in a cube.

• The angles in a cube are right angles, that is, 90 degrees.

• The length, breadth and height of a cube are all equal.

\begin{array}{l}

L=B=H \\

\end{array}

Notes

• Euler’s formula helps determine the relationship between vertices, edges and faces.

• The volume of a cube is:

\begin{array}{l}

Volume\: of\: a\: cube=a^{3} \\

\end{array}