How Many Circles Can Be Drawn Through Two Points

An infinite number of circles can be drawn through two given points. If we consider any two points on a plane, there are an unlimited number of circles that can pass through them. If we start drawing the circles by taking the two points to firstly function as the diameter of the first circle, we can then move up along the circumference of the circle such that the points become a chord of the circle instead. In a similar way, we can keep moving down the circumference to make the points become chords of varying lengths and draw an infinite number of circles through the same two points.

Example

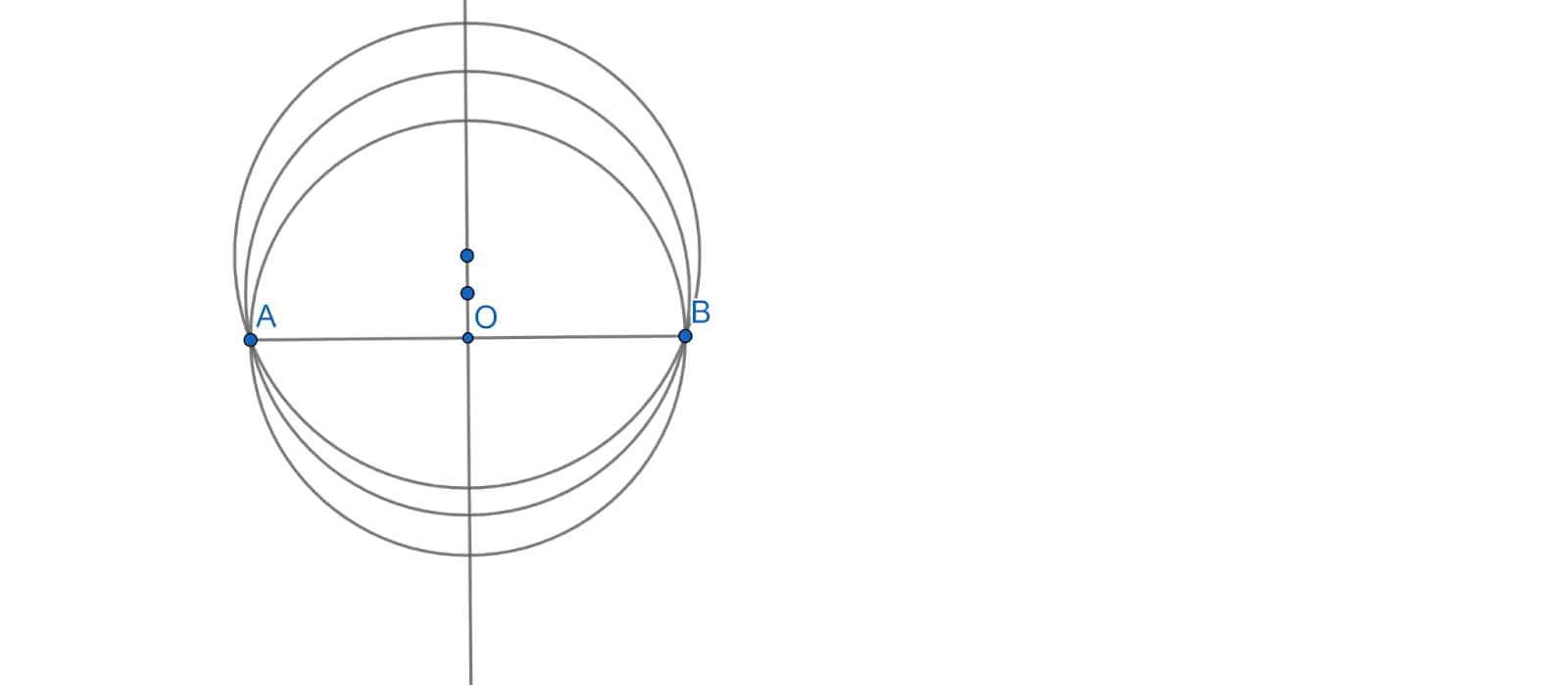
Take two random points A and B. Join these two points to form a line segment. Now draw the perpendicular bisector of this line segment. Consider any point on the perpendicular bisector as the centre O. Join O to A to form OA or O to B to form OB and take this to be the radius of any circle. This circle will pass through both points A and B. As we change the position of O on the perpendicular bisector, the radius of the circle will change and a new circle can be formed at every point. In this way, an infinite number of circles can be drawn that pass through points A and B.

Conclusion

There is an infinite number of circles that can be drawn through two given points.