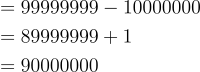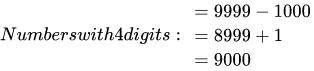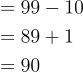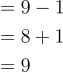How Many 6-Digit Numbers are There in All
Introduction
Numbers are known to go till infinity. The whole number starts from 0 to infinity. From 1 to 9, there is only one digit present in each number, and from 50001 to 99999, there are a total of 5 digits in each number. In this article, we are looking forward to figuring out how many numbers have 6 digits in mathematics.
How to Figure out All 6 Digit Numbers
In general, to find the total number of n-digit numbers, we follow these three steps:
Find the largest n-digit number.
Find the smallest n-digit number.
Subtract the largest n-digit number with the smallest n-digit number.
Add 1 to the solution.
Now, following the major steps, let’s figure out the total number of 6-digit numbers.
Largest 6-digit number: 999999
Smallest 6-digit number: 100000
Therefore, the total number of 6-digit numbers
\begin{aligned}
&=999999-100000\\
&=899999+1\\
&=900000
\end{aligned}
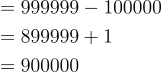
Hence, there are a total of Nine Lakh numbers with 6 digits.
Frequently Asked Questions (FAQs)
Q: Find the total numbers with 8 and 4 digits, and subtract them.
A:
Numbers with 8 digits: \begin{aligned} &=99999999-10000000\\ &=89999999+1\\ &=90000000 \end{aligned}
| Numbers with 4 digits: \begin{aligned} &=9999-1000\\ &=8999+1\\ &=9000 \end{aligned}
|
Nine crore | Nine thousand |
On subtracting them, we get:
\begin{aligned}
&=90000000-9000\\
&=89991000
\end{aligned}
Q: Find the total numbers with 2 digits and 1 digit, and also add them.
A:
Numbers with 2 digits: \begin{aligned} &=99-10\\ &=89+1\\ &=90 \end{aligned}
| Numbers with 1 digits: \begin{aligned} &=9-1\\ &=8+1\\ &=9 \end{aligned}
|
Ninety | Nine |
On adding them, we get:
\begin{aligned}
&=90+9\\
&=99
\end{aligned}
Ninety nine
Q: What is the smallest and largest 9-digit number?
A:
The smallest and largest 9-digit numbers are 100000000 and 999999999, respectively.
Q: How many 7-digit numbers are there in all?
A:
To find 7-digit numbers, we use the steps mentioned earlier in the topic.
Largest 7-digit number: 9999999
Smallest 7-digit number: 1000000
Therefore, the total number of 7-digit numbers are:
\begin{aligned}
&=9999999-1000000\\
&=8999999+1\\
&=9000000
\end{aligned}
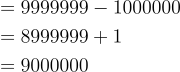
There are a total of Ninety Lakh numbers with 7 digits.
Q: How many 6-digit numbers are left if we subtract 50% of them?
A:
Total 6 digit numbers are 900000, On subtracting 50% of 900000, we get:
\begin{aligned}
&=900000\times(\frac{50}{100})\\
&=450000
\end{aligned}
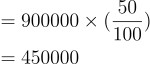
Hence, a total of forty lakh, fifty thousand numbers are left when we subtract 50% of 900000
Study Resources, Applications and Opportunities
Take Aakash iACST and get instant scholarship on coaching programs.
NEET Most scoring concepts
Get nowThis ebook serves as a valuable study guide for NEET 2025 exam.
NEET Previous 10 Year Questions
Get nowThis e-book offers NEET PYQ and serves as an indispensable NEET study material.
JEE Main Important Physics formulas
Get nowAs per latest syllabus. Physics formulas, equations, & laws of class 11 & 12th chapters
JEE Main Important Chemistry formulas
Get nowAs per latest syllabus. Chemistry formulas, equations, & laws of class 11 & 12th chapters
JEE Main high scoring chapters and topics
Get nowAs per latest 2024 syllabus. Study 40% syllabus and score upto 100% marks in JEE