How Many Edges Does a Polyhedron Have

How Many
How Many Edges Does a Polyhedron Have

How Many Edges Does a Polyhedron Have

Team Careers360Updated on 12 Apr 2023, 03:21 PM IST

The edges of a polyhedron can be found by the formula F + V - E = 2 where F is the number of faces, V is the number of vertices and E is the number of edges.

A polyhedron is a three-dimensional geometric shape with flat, polygonal faces, straight edges, and pointed vertices. Some examples of polyhedrons include cubes, pyramids, prisms etc…

One interesting thing to note is that cones and spheres cannot be polyhedrons since their faces are not polygons.

Faces, Edges and Vertices

The three parts of a polyhedron are faces, edges, and vertices.

Face: The flat top of a polyhedron is referred to as its "face." They are basically polygons.

Edge: The edge is the line segment that connects the two faces.

Vertices: A vertex is the point of intersection of two edges.

See the face, vertex, and edges of a shape in the following example.

Euler’s Formula

The relationship between the number of edges, faces and vertices of a polyhedron is defined by a formula called Euler’s formula.

Where,

F = Number of faces

V = Number of vertices

E = Number of edges

So, with this formula, you can easily find the answers to questions like ‘How many edges does a polyhedron have’ or ‘How many vertices does a polyhedron have’.

Solved Examples

How many edges does a polyhedron with 8 faces and 12 vertices have?

F = 8

V = 12

Euler’s formula:

8 + 12 - E = 2

E = 18

Number of edges = 18

A polyhedron has 7 faces and 10 vertices. How many edges does that polyhedron have?

F = 7

V = 10

Euler’s formula:

7 + 10 - E = 2

E = 15

Number of edges = 15

Polyhedron Types

The two main types of polyhedra based on their edges are

Regular polyhedron
Irregular polyhedron

Regular Polyhedron

They are made up of regular polygons. That means, its edges are congruent. The term "platonic solid" is sometimes used to describe a regular polyhedron. In a regular polyhedron, all polyhedral angles are equal. There are five common regular polyhedra in the scene. The following is a list of the five common polyhedrons.

Tetrahedron

A tetrahedron has four faces, six edges, and four vertices (corners). The shape of each face is an equilateral triangle. One of the triangles can be considered the base and the other three form the pyramid. It is also known as a triangular pyramid. The interior angles of a tetrahedron in each plane add up to 180 degrees.

1681292130485

Cube

A cube has 6 faces, 12 edges and 8 vertices. The shape of each face can be seen as a square. It is also called a reg ular hexagon or square parallelepiped.

1681292130051

Regular Octahedron

A regular octahedron has 8 faces, 12 edges, and 6 vertices, each of which is an equilateral triangle. It is a shape that is formed by joining by two pyramids at its bases.

1681292131279

Regular dodecahedrons

It has 20 vertices, 12 faces, and 30 edges. Each face resembles a standard pentagon. A dodecahedron is made up of 12 congruent pentagons with 3 pentagonal faces meeting at each of its 20 vertices.

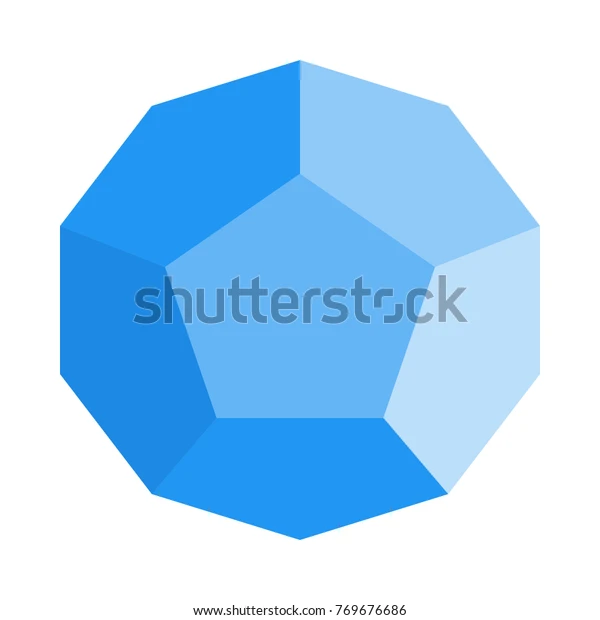
1681292130310

Regular icosahedron

It is one that has 20 faces, 30 edges, and 12 vertices. A regular icosahedron has an equilateral triangle as the form of each face.

1681292130703

Irregular Polyhedron

An irregular polyhedron has faces with uneven polyhedral angles and faces that are not congruent with one another.

Example:

Hexagonal Pyramid

It has a hexagonal base, with 6 sides and 6 triangular lateral faces. A hexagonal pyramid is also known as Heptahedron.

1681292130888

Conclusion

So, we have leant about different types of polyhedron for ex tetrahedron, cube, dodecahedron etc. Euler’s formula gives us the relationship between number of faces, vertices and edges of a polyhedron. Using this relation, we can find the required number of edges based on number of faces and vertices.

Upcoming School Exams

BITS Law Admission Test