ALLEN Coaching
ApplyRegister for ALLEN Scholarship Test & get up to 90% Scholarship
To say or write “4000” in words, you have to understand the significance of “4000” first. At first glance, you will say “4000” is a number. That is certainly true. But if you denote it with any unit of measurement (like a centigram, litre, etc.) after “4000”; it will, clearly, indicate some amount of respective physical quantity (like “4000” kg of sugar or “4000” dollars).

As such, “4000” as a number is made up of digits 4, 0, 0, and 0. Here, every digit has its own unique interpretation depending upon its place value. Let us, deep dive, into it to realize it further.
A system is defined as a decimal number system in which we find the following salient features.
Each constituent digit of the given number system denotes its own place value.
With reference to the right-hand-most digit, the place value of each constituent digit of the number is determined.
As you move away from the right-hand-most digit to the left, the place value grows as powers of 10.
The unit’s or one’s place is the specific place value of a digit in its right-hand most position of the number.
The ten’s place is the unique place value of the digit after the immediate left-hand side to the unit’s place.
The hundredth place denotes the place value of the digit at the immediate leftmost side from the ten’s place. It is followed by thousandth place and ten thousandth place respectively.
Ten crore’s place value | crore place value | Ten lakh’s place value | lakh place value | Ten thousandth place value | Thousandth place value | Hundredth place value | Ten’s place value | Unit or One’s place value |
|
|
|
|
|
|
At the first glimpse of “4000”, it is not associated with any unit of measurement with it, and it follows that “4000” is a number.
The number of digits present in the number “4000” is four.
Following the decimal number system, you have the following place values of the four digits of 4000.
The unit’s place in the number “4000” is ‘0’.
The ten’s place in the number “4000” is ‘0’.
The hundred place in the number “4000” is ‘0’
The thousandth place in the number “4000” is ‘4’.
Thousandth place value | Hundredth place value | Ten’s place value | Unit or One’s place value |
4\times {{10}^{3}}
| 0\times {{10}^{2}} | 0\times {{10}^{1}}
| 0\times {{10}^{0}}
|
Mathematically you can have the following
\[\begin{align}
4\times {{10}^{3}}+0\times {{10}^{2}}+0\times {{10}^{1}}+0\times {{10}^{0}} \
=4\times {{10}^{3}} \
=“4000”
\end{align}\]
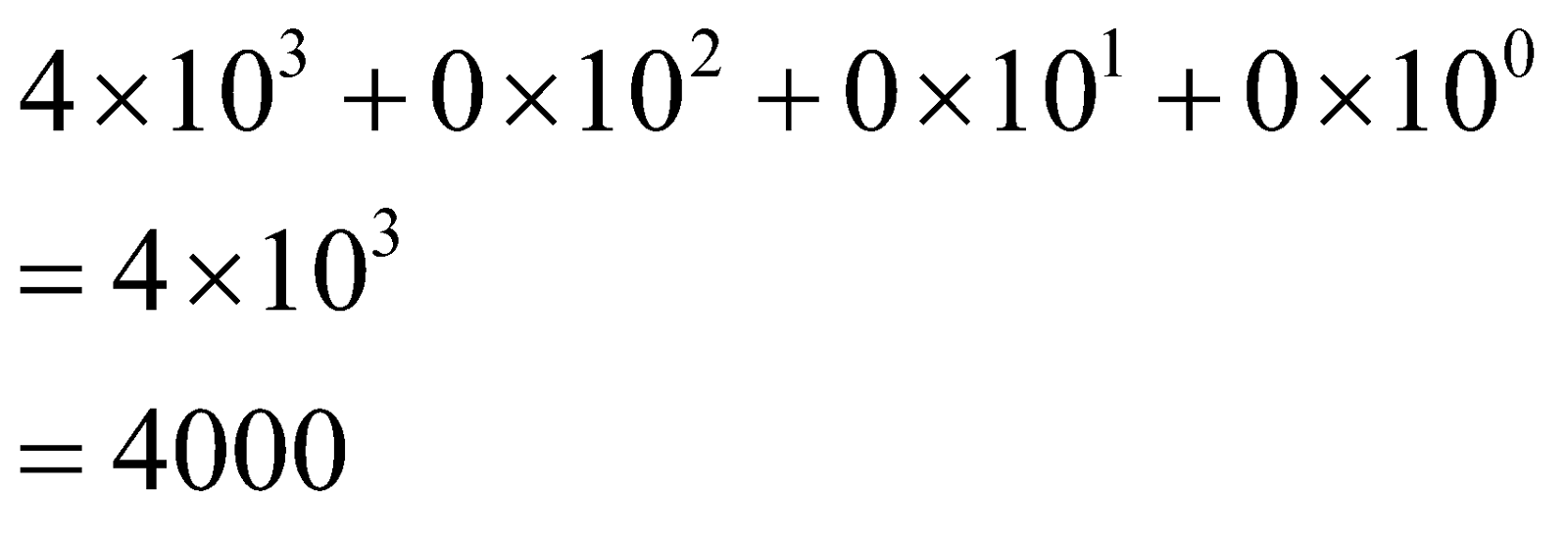
Here, you notice that “4000” has only one non-zero digit 4 placed in its thousandth place. The remaining digits in its hundredth place, ten’s place, and unit’s place are all zeros respectively.
Therefore, you can express “4000” in words as defined in the decimal number system taking into account its thousandth place only.
Thus, you declare that “4000” is written as ‘Four Thousand’ in words.
In the term "4,000 rupees” you can see that the number "4000" is having the adjoining unit "rupees".
You can easily express the number in words. Then position the specific unit “rupees” following the number.
So, you say that “4000 rupees” is written as “Four Thousand rupees” in words.
On dividing any number by 2 you get either of the following cases.
The number gets fully divisible by 2. Then you call it an “even number”.
The number does not get fully divisible by 2. Then you call it an “odd number”.
You get that the number “4000” is absolutely divisible by 2, thus making it an even number, and not an odd number.
Any number, if expressible, as the product of two equal real numbers, you call it a “perfect square number”.
![]()
Here you cannot reduce the product of two equal real numbers to “4000”. Therefore, you can write that the number “4000” is not a perfect square.
You spot any number equivalent to the product of three equal real numbers, you call it a “perfect cube number”.
![]()
Here you cannot equate “4000” to the product of any three equal real numbers. Therefore, you the number “4000” is, definitely, not a perfect cube.
A number that exhibits only two factors such that the factors are 1 and the number itself, you call such a number a “prime number”.
Again, a number that splits into more than two factors, you can tag it as a “composite number”.
![]()
Here you find that more than two factors are present for the number “4000”. Thus, you infer that “4000” is not a prime number but a “composite number”.
Any natural or counting number can be alternatively called a “cardinal number”.
The number “4000” definitely suggests a specific counting number. So, it is considered as a “cardinal number”.
If you use the extension “st” or “rd” or “th” (as it applies) after its numeric value of any number to designate any place or position in a set, series or collection. You call such a number to be an ordinal number. For example: 1st, 2nd, 3rd, 4th, and so on.
Thus, you can get “4000th” as the “ordinal number”.
You know that a perfect cube number is always equivalent to the product of multiplication among any three equal real numbers.
![]()
By inspection, you discover that 2 is the least positive integer to be multiplied with “4000” to obtain the required perfect cube, which is as follows.
\[\begin{array}{*{35}{l}}
4000\times 2={{2}^{5}}\times {{5}^{3}}\times 2 \
={{2}^{6}}\times {{5}^{3}} \
={{\left( {{2}^{2}}\times 5 \right)}^{3}} \
={{\left( 4\times 5 \right)}^{3}} \
={{20}^{3}} \
=8000 \
\end{array}\]
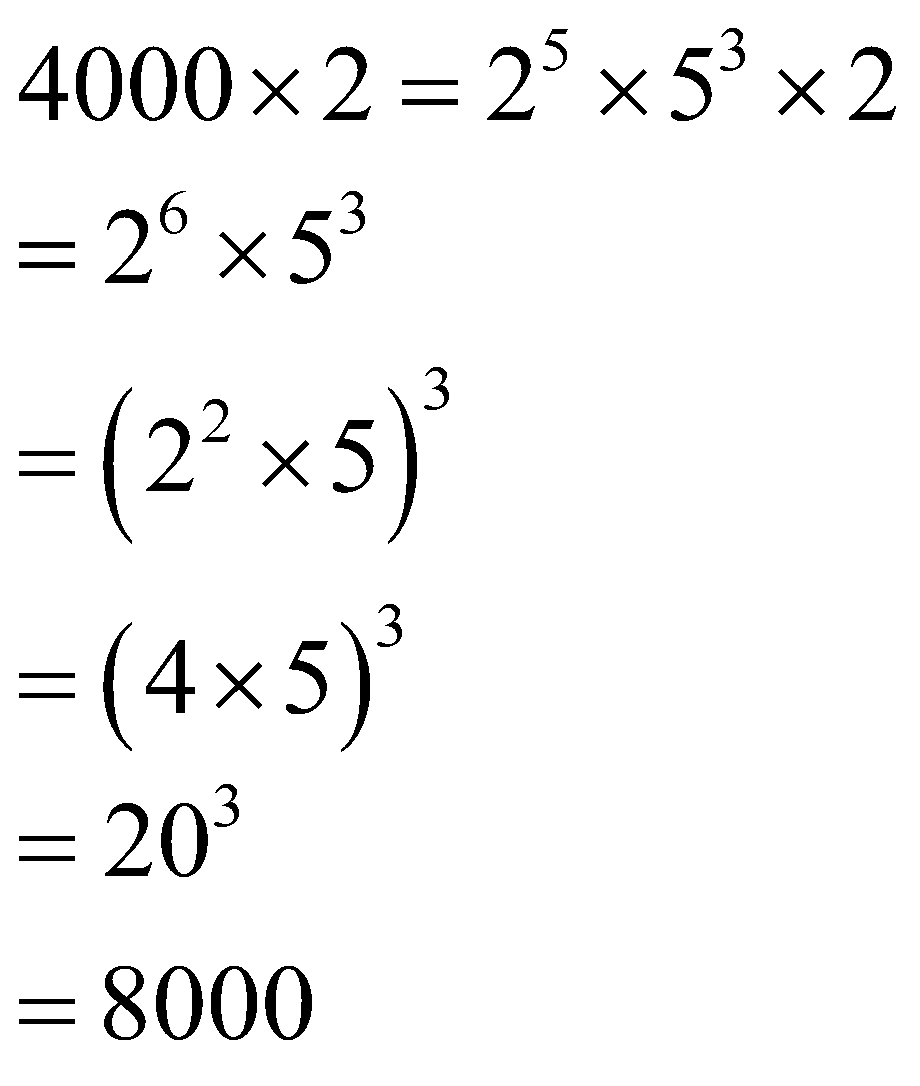
The calculated least number for the needed purpose is 2.
Yes, the number “4000” is a natural number as in the number line it belongs to the defined collection of natural numbers.
\[\begin{align}
N=\left\{ \ 1,\ 2,\ 3,\ \ldots +\infty \right\},\text{ where} \
\infty \text{ denotes infinity and + indicates positive value or direction} \
\end{align}\]
![]()
Yes, the number “4000” is an integer as within the number line it belongs to the defined collective set of the integers given by
![]()
Yes, you can, definitely write “4000” in the form of a complex number; where “4000” implies the real part and, the imaginary part is implied by “0”.
![]()
Yes, the number “4000” is a rational number as you can rewrite “4000” in the specific fraction form of p/q where it is defined that both p and q are integers but q can never be equal to “0”.
\[\begin{align}
80000=\frac{80000}{1} \
=\frac{p}{q},\text{where}\ p=80000,\ q=1\left( \ne 0 \right)
\end{align}\]
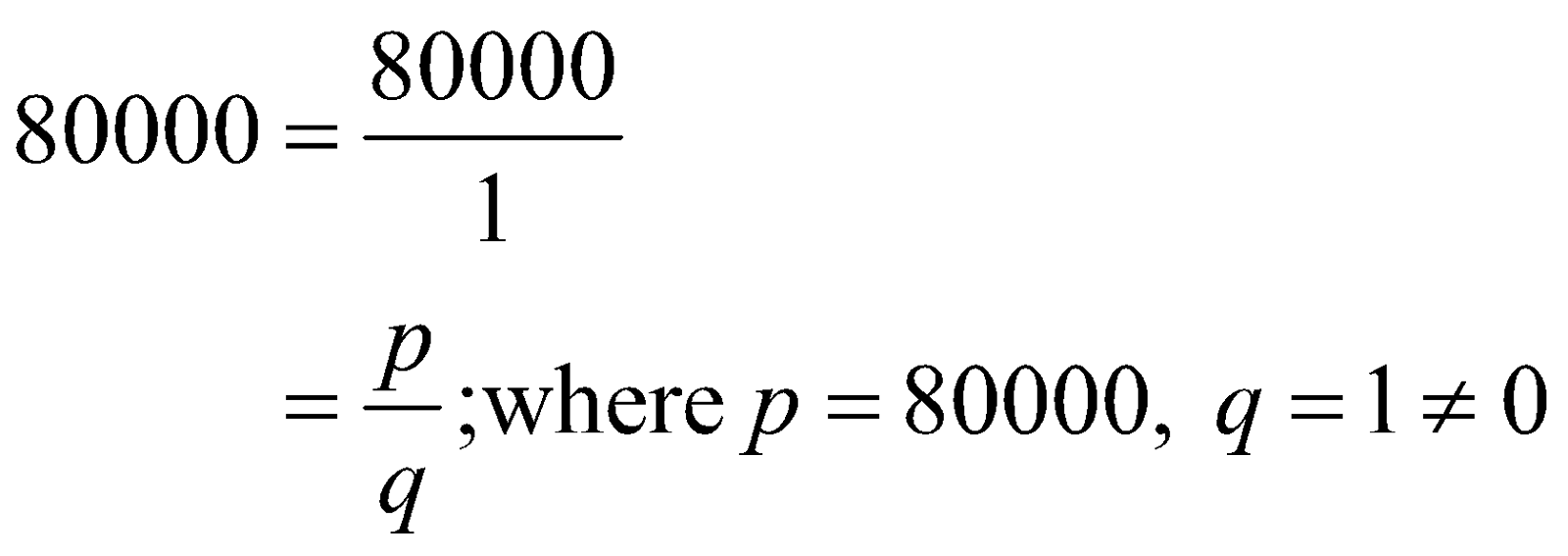

Register for ALLEN Scholarship Test & get up to 90% Scholarship

Get up to 90% Scholarship on Offline NEET/JEE coaching from top Institutes

This ebook serves as a valuable study guide for NEET 2025 exam.

This e-book offers NEET PYQ and serves as an indispensable NEET study material.

As per latest syllabus. Physics formulas, equations, & laws of class 11 & 12th chapters
As per latest syllabus. Chemistry formulas, equations, & laws of class 11 & 12th chapters