32000 In Words
This Story also Contains
- What Are the Natural Numbers?
- Can You Write "32000" In Words?
- Take a note of the following place values of the five digits of "32000."
- Mathematically you can conclude the following
- Can You Write "32000 Rupees” In Words?
- Can You Say If "32000" Is An Even Or An Odd Number?
- Is "32000" A Perfect Square Number?
- Do You Know If "32000" Is A Prime Or A Composite Number?

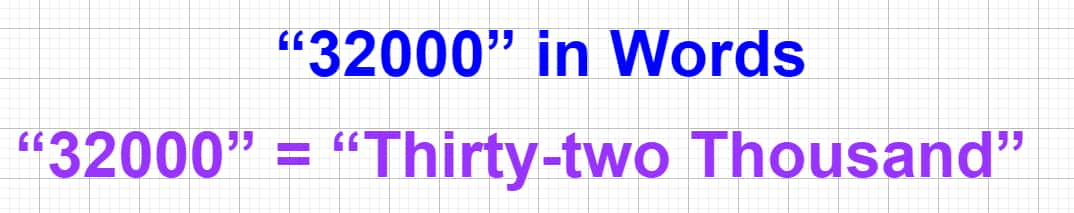
From the first impression, it suggests that “32000” is indicating a number. That is absolutely correct. But if you assign a unit of measurement (like a milligram, a millisecond, etc.) with "32000"; it will undoubtedly point out some physical quantity (like "32000 grams" of sugar or "32000 minutes"). Isn’t it right?
As such, "32000" as a number is made up of the digits 3, 2, 0, 0, and 0. Interestingly, every digit signifies differently as dictated by its place value. when you identify the actual position or place value of any digit of "32000" in the decimal number system, you can write "32000" in words. Let us explore it further.
What Are the Natural Numbers?
You have noticed that it is most common to use the numbers [1, 2, 3,] ![]() ; to count or get the "quantity" of items, like the number of items in your box, or the number of writing pads in your bookshelf.
; to count or get the "quantity" of items, like the number of items in your box, or the number of writing pads in your bookshelf.
The numbers, which you are using for counting, are, in fact, the most common and fundamental entities described in mathematics. They are referred to as"natural numbers" or “counting numbers."
Mathematically, you can point out each of the natural numbers in the number line, and this collective set of natural numbers is represented as follows:
\[\begin{align}
N=\left\{ \ 1,\ 2,\ 3,\ \ldots +\infty \right\},\text{ where} \\
\infty \text{ denotes infinity and + indicates positive value or direction} \\
\end{align}\]
![]()
Thus, "32000" also falls under the category of “natural numbers.”
In general, the decimal number system is used to define any number.
Can You Write "32000" In Words?
Note that "32000" has no unit of measurement associated with it, and so "32000" is a number comprising five digits.
First, try to mark the place value of each digit of "32000" with reference to the decimal number system.
The place value of each constituting digit of any number in the decimal number system is obtained with reference to the rightmost digit. Actually, the place value increases as “powers of 10” as you shift from the right-hand side digit of the referred number in the decimal number system.
Note its following defining features.
The unit’s or one’s place - It indicates the place value of a digit in the right-hand most position of a number.
Similarly, as you gradually shift “digit-wise” from the unit’s place towards the left side, you will achieve the place values of ten’s place, hundreds’ place, thousand’s place, and so on, as described below.
Ten crore’s place value | Crore’s place value | Ten lakh’s place value | lakh place value | Ten thousandth place value | Thousandth place value | Hundredth place value | Ten’s place value | Unit or One’s place value |
\[{{10}^{8}}\]
| \[{{10}^{7}}\]
| \[{{10}^{6}}\]
| \[{{10}^{5}}\]
| \[{{10}^{4}}\]
| \[{{10}^{3}}\]
| \[{{10}^{2}}\]
| \[{{10}^{1}}\]
| \[{{10}^{1}}\]
|
Take a note of the following place values of the five digits of "32000."
Its unit’s place is "0."
Its tenth place is equal to "0."
Its hundredth place equals "0."
Its thousandth place is "2."
Its ten thousandth place is "3".
Ten thousand place value | Thousandth place value | Hundredth place value | Ten’s place value | Unit or One’s place value |
\[3\times {{10}^{4}}\]
| \[2\times {{10}^{3}}\]
| \[0\times {{10}^{2}}\]
| \[0\times {{10}^{1}}\]
| \[0\times {{10}^{0}}\]
|
Mathematically you can conclude the following
\[\begin{align}
3\times {{10}^{4}}+2\times {{10}^{3}}+0\times {{10}^{2}}+0\times {{10}^{1}}+0\times {{10}^{0}} \\
=3\times {{10}^{4}}+2\times {{10}^{3}}+0+0+0 \\
=\left( 30+2 \right)\times {{10}^{3}} \\
=32000 \\
\end{align}\]
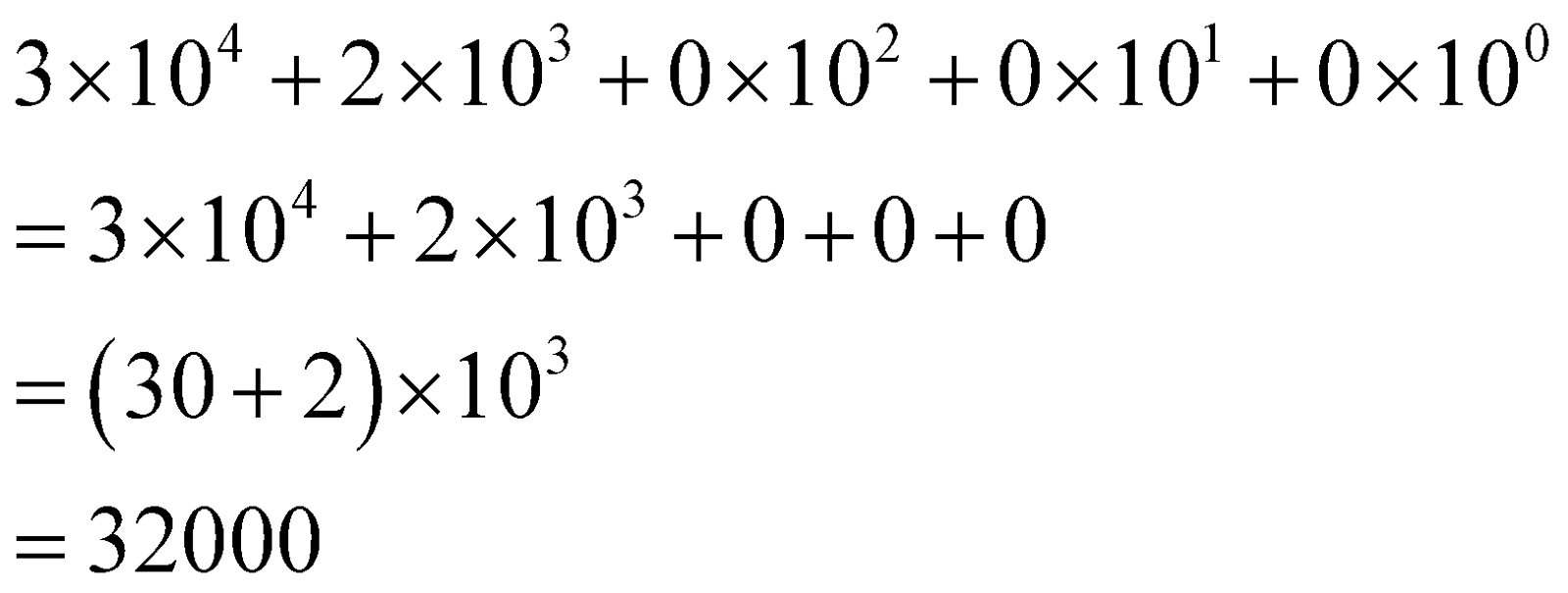
Here, you notice only two non-zero digits, “3” and “2” in the number "32000" in its ten thousand’s place and thousand’s place respectively. See that all the other digits occupying the hundredth place, ten’s place, and unit’s place are zero.
Thus, "32000" is “Thirty-two Thousand” in words.
Can You Write "32000 Rupees” In Words?
In the term "32000 rupees,” it is the unit "rupees" that immediately follows the number "32000."
So, express it by first writing the number in words and next to it place the unit "rupees".
So, you should write "32000 rupees” as “Thirty-two Thousand rupees” in words.
Can You Say If "32000" Is An Even Or An Odd Number?
Note that an “even number” is a number that is completely divisible by 2. Otherwise, the number becomes an “odd number.”
Here, there remains no remainder when you perform the following division..
\[32000\div 2=16000\]
![]()
As the number "32000" is completely divisible by 2, you can easily conclude that “32000” is not an odd number. So it is, definitely, an “even number.”
Is "32000" A Perfect Square Number?
You can declare any number as a “perfect square” in the case you can split that number into two equal integers.
Now, expand “32000” in the following way:
\[32000={{2}^{8}}\times {{5}^{3}}\]
![]()
Here you find that "32000" is not equal to any product of two equal numbers. Hence, "32000" is, evidently, not a perfect square.
Do You Know If "32000" Is A Prime Or A Composite Number?
Any number displaying only two factors, one being itself and the other factor being 1, is a “prime number”.Conversely, the number becomes a “composite number” which has more than two factors.
Here, you notice the following factors:
\[\text{Factors of 320}00=\ 1,\ 2,\ 4,\ 5,\ 8,\ 10,\ \ldots \ 32000\]
![]()
So, you find that the number "32000" has more than two factors.
Thus, "32000" is not a prime number. It is certainly a “composite number.”
Frequently Asked Questions (FAQs)
Yes, you can absolutely express "32000" as a “complex number,” where "32000" becomes the real part, while the imaginary part becomes “0.”
\[32000=32000+i.0\]
![]()
Yes, "32000" is a “rational number,” as you can express "32000" in the form of a fraction p/q, where p and q are assumed to be integers and q is never equal to “0.”
\[\begin{align}
32000=\frac{32000}{1} \\
=\frac{p}{q};\text{ where }p=32000,\ q=1\ne 0
\end{align}\]
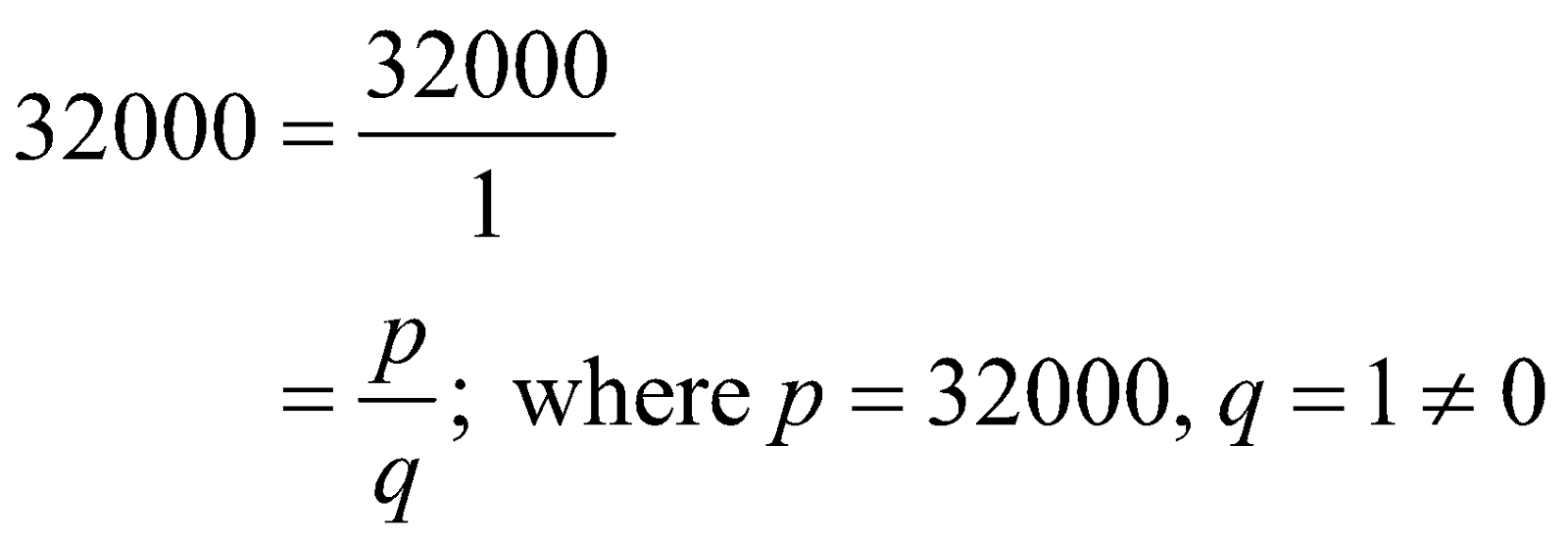
Yes, "32000" is called an “integer,” as evident from its inclusion in the collection of integers in the number line.
\[Z=\left\{ -\infty ,\ \ldots ,\ -3,\ -2,\ -1,\ 0,\ 1,\ 2,\ 3,\ \ldots +\infty \right\}\]
![]()
Yes, "32000" is called a “whole number,” because it occurs in the collective set of the whole numbers of the number line.
\[\begin{align}
W=\left\{ \ 0,\ 1,\ 2,\ 3,\ \ldots +\infty \right\},\text{ where} \\
\infty \text{ denotes infinity and + indicates positive value or direction} \\
\end{align}\]
![]()
The number “32000” is truly a specific number that is a natural or a counting one. Therefore, it is, definitely, a cardinal number.
Applications for Admissions are open.
This ebook serves as a valuable study guide for NEET 2025 exam.
NEET Previous 10 Year Questions
Get nowThis e-book offers NEET PYQ and serves as an indispensable NEET study material.
JEE Main Important Physics formulas
Get nowAs per latest syllabus. Physics formulas, equations, & laws of class 11 & 12th chapters
JEE Main Important Chemistry formulas
Get nowAs per latest syllabus. Chemistry formulas, equations, & laws of class 11 & 12th chapters
JEE Main high scoring chapters and topics
Get nowAs per latest 2024 syllabus. Study 40% syllabus and score upto 100% marks in JEE
JEE Main Important Mathematics Formulas
Get nowAs per latest syllabus. Maths formulas, equations, & theorems of class 11 & 12th chapters