100 in Words
Introduction
100 is written as “One Hundred” in words in the English language.
Do you know what this "100" signifies? How can you say or write "100" in words? From the first impression, “100” appears to be a number. Isn’t it right?
In case of measurement purposes you may add some “units” (like a mg, a cm, etc.) along with "100", what will it mean? It will, then, indicate some physical quantity (like "100 g" of chocolate cake, or "100 ml” of oil).
As such, "100" is a number comprising the digits 1, 0, and 0; and here the position or place value of every digit matters in assigning the overall value to the number. Let us explore it further.
How Is The Decimal Number System Designed?
The design of the decimal number system is described under the following heads.
It is an organized arrangement of numbers employing the “indices of 10” for indicating the place values of the digits of every number.
On knowing the unique place value or the position of every single digit of the number, you can express a number in words.
To determine the place value of any digit in a decimal number, the reference from the rightmost digit is universally accepted. The place value, basically, increases by “a power of 10” as you go towards the “left-hand side” from the first digit in the right-hand side of the number.
Ten Lakhs | Lakhs | Ten Thousands | Thousands | Hundreds | Tens | Units or Ones |
\[{{10}^{6}}\]
| \[{{10}^{5}}\]
| \[{{10}^{4}}\]
| \[{{10}^{3}}\]
| \[{{10}^{2}}\]
| \[{{10}^{1}}\]
| \[{{10}^{1}}\]
|
Can You Write "100" In Words?
As we find no unit of measurement after or before ‘"100". So, evidently, "100" represents a unitless number only.
You get only 3 digits in the number "100".
Observe that the decimal number system reveals the following information about the place values of these 3 digits of "100".
The unit’s place in the number "100" is "0".
The ten’s place in the number "100" is "0".
The hundredth place in the number "100" is "1".
Hundredth place value | Ten’s place value | Unit or One’s place value |
\[1\times {{10}^{2}}\]
| \[0\times {{10}^{1}}\]
| \[0\times {{10}^{0}}\]
|
Mathematically you can write the following
\[\begin{align}
1\times {{10}^{2}}+0\times {{10}^{1}}+0\times {{10}^{0}} \\
=1\times {{10}^{2}} \\
=100 \\
\end{align}\]
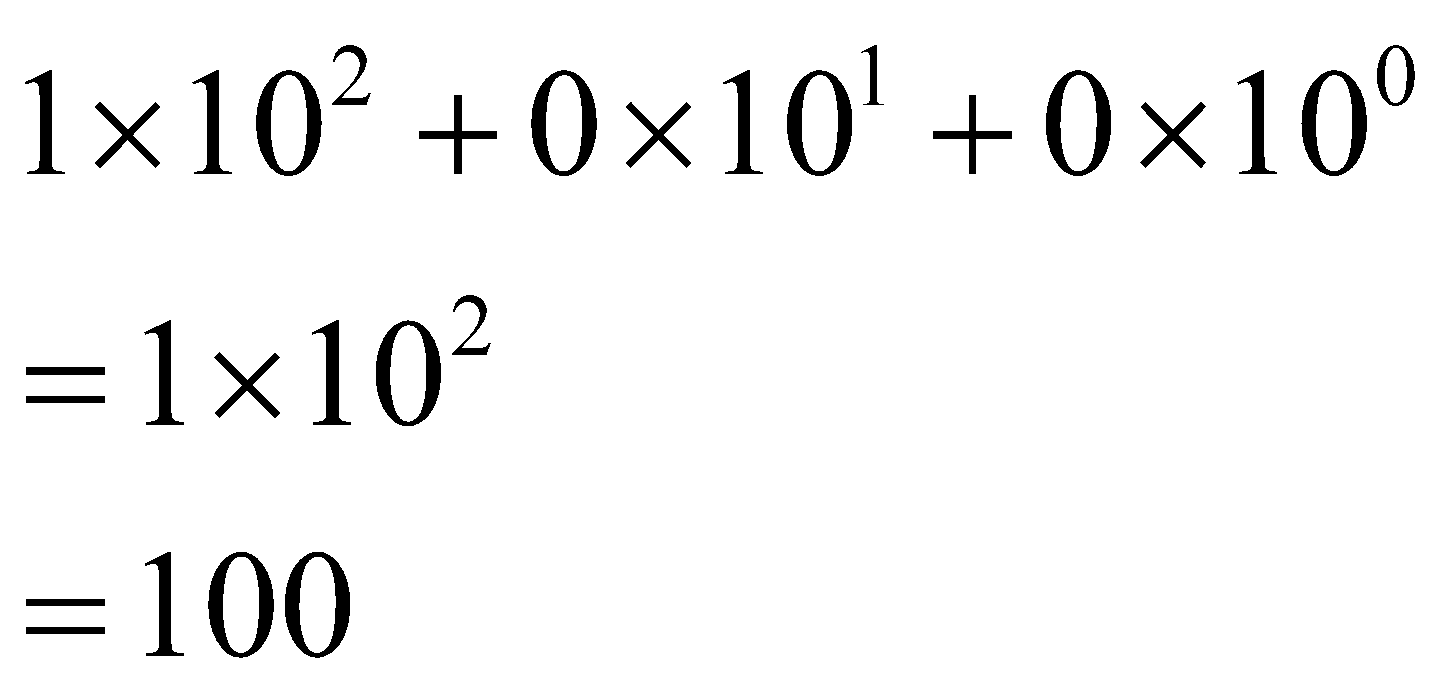
Now, you see that there is only one non-zero digit “1” in the hundredth place of "100"; while its ten’s place and unit’s place have digits 0, and 0.
Thus, you can represent "100" in words as per the rules of the decimal number system in this way.
"100" is “One Hundred” in words.
Can You Write "100 Rupees” In Words?
In the term "100 rupees” you can, easily, identify that the number "100" is being immediately followed by the unit "rupees".
Now first write the number "100” in words. Then put the unit "rupees".
So, you get that "Rs. 100” is “One Hundred rupees” in words.
Can You Say If "100" Is An Even Or An Odd Number?
You are aware that when “2” can divide any number completely, without leaving any remainder, you declare that number as an “even number”.
On the other hand, if you get any number that leaves any remainder on being divided by “2”, it is an “odd number”.
Here, when you divide the number "100" by 2, there remains no remainder as evident from the following division.
\[100\div 2=50\]
![]()
Therefore, it can be concluded that “100” is not an odd number. It is an “even number”.
Is The Number "100" A Perfect Square?
Note that a “perfect square” is such a number, that you can write that number as the product of two equal integers.
Expand the number “100” in the following way.
\[\begin{align}
100={{2}^{2}}\times {{5}^{2}} \\
={{\left( 2\times 5 \right)}^{2}} \\
={{10}^{2}}
\end{align}\]
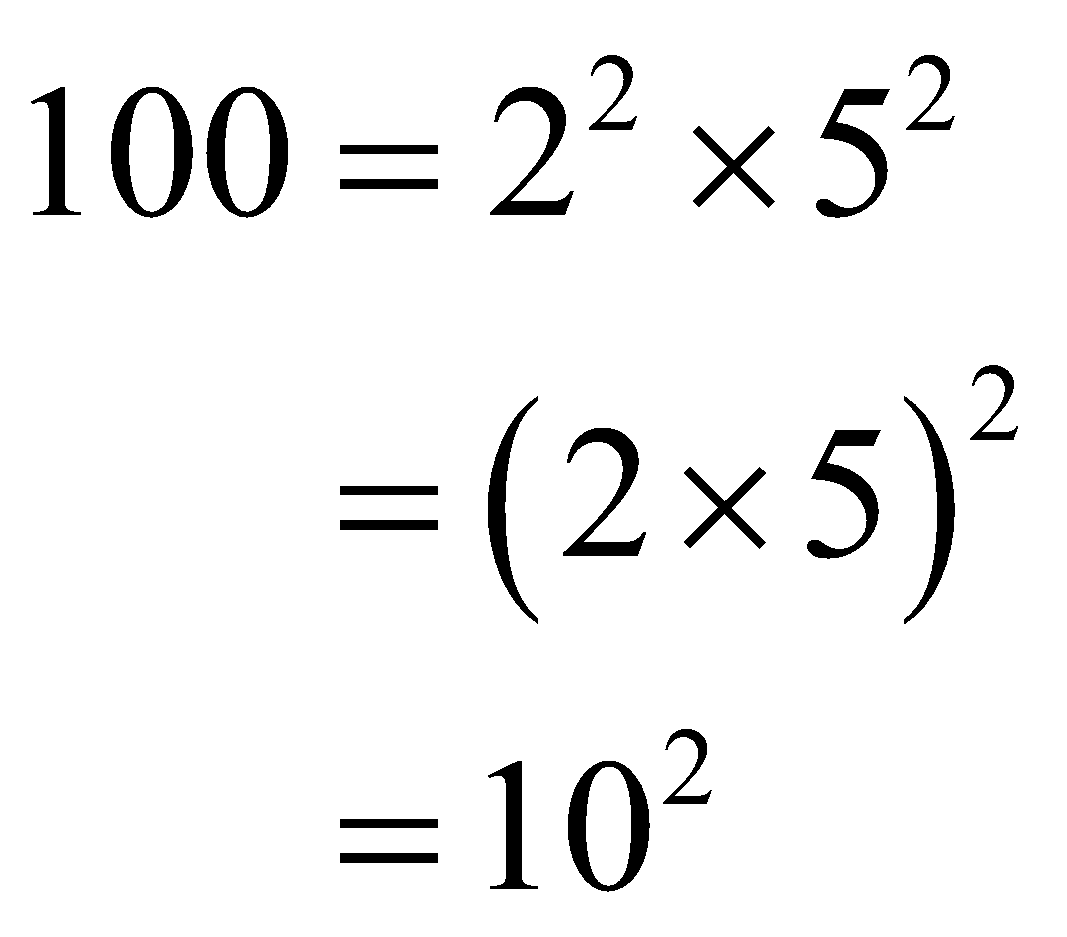
See that "100" is exactly equal to the product of two equal integers. Hence, you infer that the number "100" is, definitely, a “perfect square”.
Do You Know If "100" is A Prime Or A Composite Number?
Any particular number that on factorizing yields only two factors (“1”, and the number itself), is a “prime number”.
Conversely, when you find a number reflecting more than two factors, you call it a “composite number”.
Here, you observe the following way.
\[\text{Factors of 1}00=\ 1,\ 2,\ 4,\ 5,\ 10,\ \ldots \ 100\]
![]()
So, you find that the number of factors of "100" is more than two. This follows that "100" is not a prime number, but is, definitely, a “composite number”.
Is The Number "100" Cardinal?
Whenever you find any natural or counting number, you can, definitely, call it a “cardinal number”.
Here, the number "100" is, definitely, a specific counting number, so it falls under the category of a “cardinal number”.
Frequently Asked Questions (FAQs)
Yes, the number "100" is a “rational number”. This is because you can express "100" in terms of the fraction “p/q”, where p and q belong to integers and q is non-zero.
\[\begin{align}
100=\frac{100}{1} \\
=\frac{p}{q};\text{ where }p=100,\ q=1\ne 0
\end{align}\]
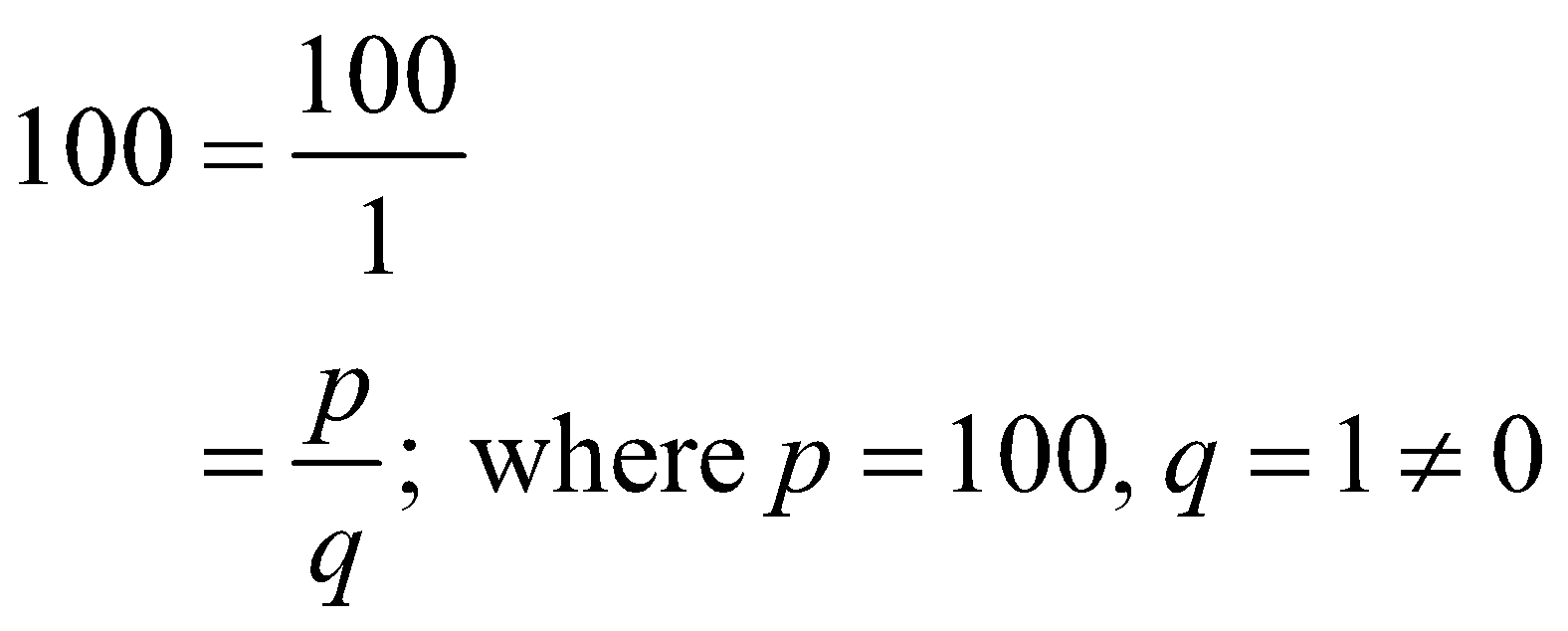
Yes, you can, here, represent "100" as a “complex number”; where "100" is the real part and “0” appears as its imaginary part.
\[100=100+i.0\]
![]()
Yes, the number "100" is an “integer” as you find it in the set of the integers of the number line.
\[Z=\left\{ -\infty ,\ \ldots ,\ -3,\ -2,\ -1,\ 0,\ 1,\ 2,\ 3,\ \ldots +\infty \right\}\]
![]()
Yes, the number "100" is a “natural number” as it appears in the number line under the collection of natural numbers.
\[\begin{align}
N=\left\{ \ 1,\ 2,\ 3,\ \ldots +\infty \right\},\text{ where} \\
\infty \text{ denotes infinity and + indicates positive value or direction} \\
\end{align}\]
![]()
A “perfect cube” is the resultant number that you get by multiplying a number by itself twice. So, you can express “a perfect cube” as the product of three equal integers.
Expand “100” in the following way.
\[\begin{align}
100={{2}^{2}}\times {{5}^{2}} \\
={{\left( 2\times 5 \right)}^{2}} \\
={{10}^{2}}
\end{align}\]
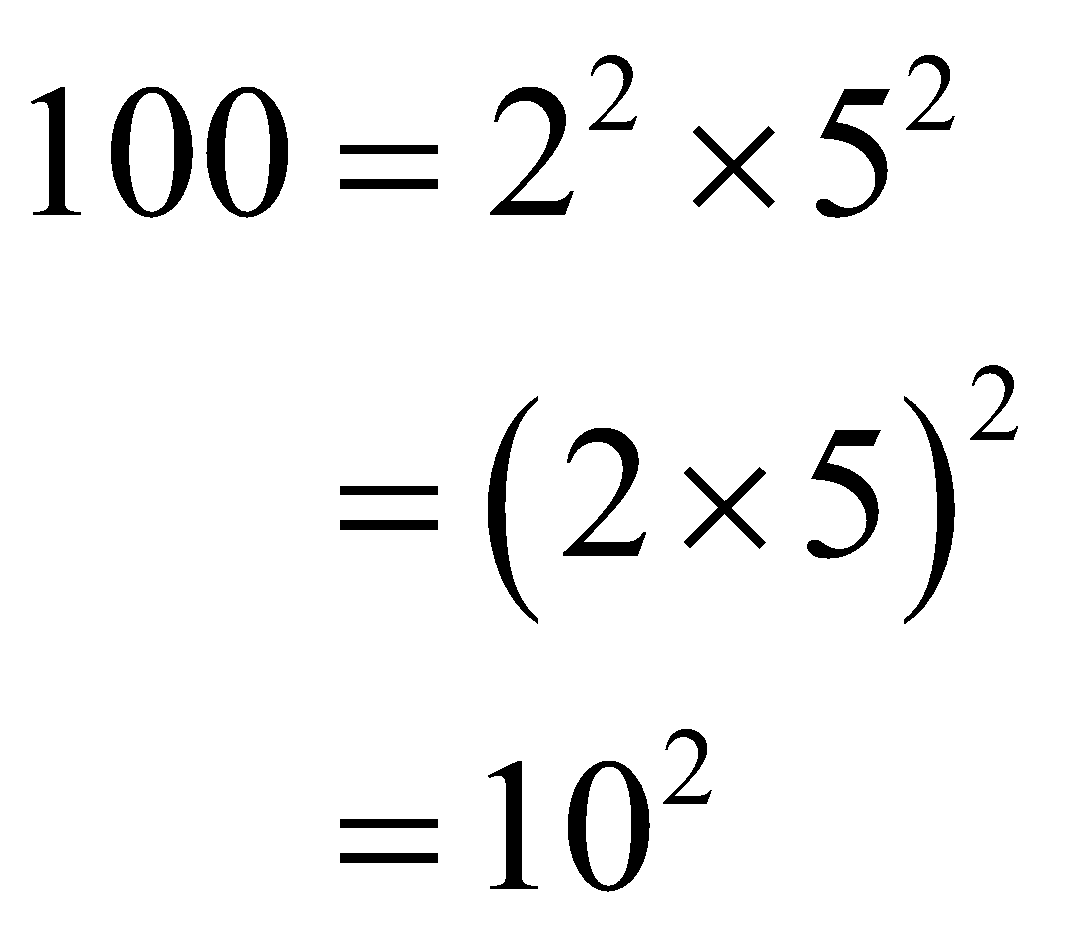
Here you get that "100" is not equal to the resultant product of the multiplication of a number by itself twice only. Hence, the number "100" is not a perfect cube.
Applications for Admissions are open.
This ebook serves as a valuable study guide for NEET 2025 exam.
NEET Previous 10 Year Questions
Get nowThis e-book offers NEET PYQ and serves as an indispensable NEET study material.
JEE Main Important Physics formulas
Get nowAs per latest syllabus. Physics formulas, equations, & laws of class 11 & 12th chapters
JEE Main Important Chemistry formulas
Get nowAs per latest syllabus. Chemistry formulas, equations, & laws of class 11 & 12th chapters
JEE Main high scoring chapters and topics
Get nowAs per latest 2024 syllabus. Study 40% syllabus and score upto 100% marks in JEE
JEE Main Important Mathematics Formulas
Get nowAs per latest syllabus. Maths formulas, equations, & theorems of class 11 & 12th chapters