Additive Inverse (Definition, Properties & Examples)

Additive inverse is a concept that is defined in mathematics at the elementary level of schooling. We are introduced with this concept in junior classes. Additive inverse means that a number which we add to another number to get the final result as 0. It has wide range of applications in daily life such as banking and finance, physics and engineering, etc. In this article we will cover additive inverse meaning, along with various examples and properties.

JEE Main: Study Materials | High Scoring Topics | Preparation Guide

JEE Main: Syllabus | Sample Papers | Mock Tests | PYQs

This Story also Contains

1. What is Additive Inverse?
2. Additive Inverse of Different Numbers
3. Difference Between Additive Inverse and Multiplicative Inverse
4. Additive Inverse Examples

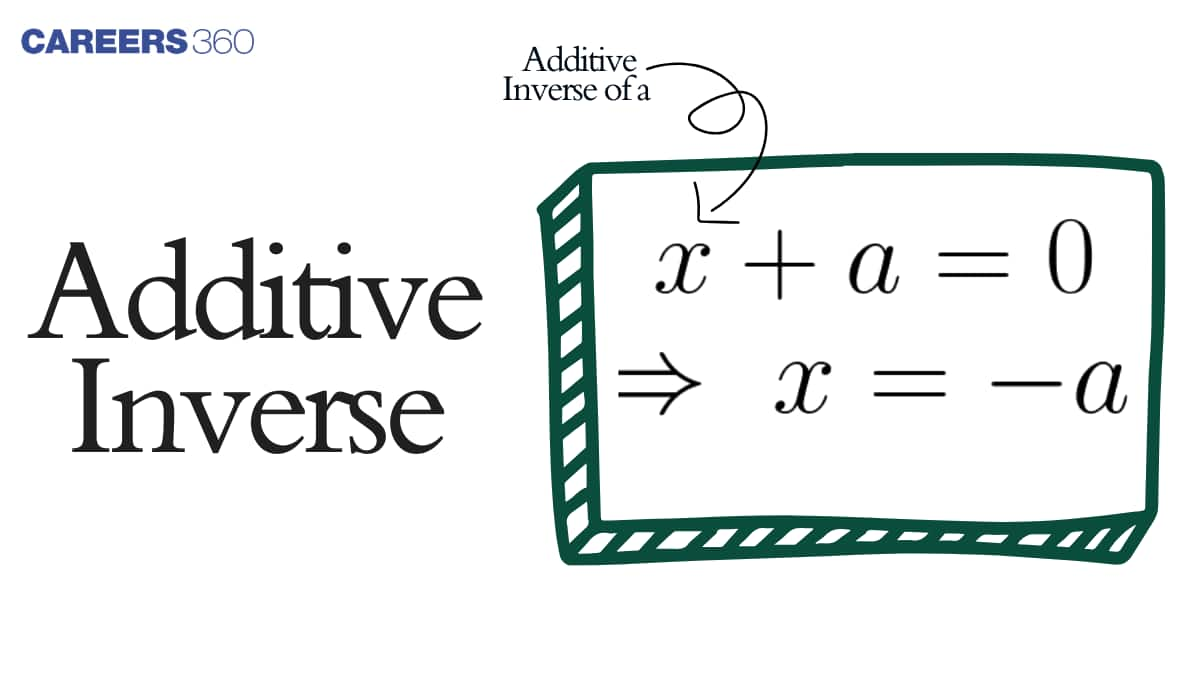
What is Additive Inverse?

The additive inverse of a number is the value, which if we add with the original number results in 0. The additive inverse is also called the opposite of the number. Additive inverse means negation of number or changed sign of original number. We suppose, $p$ is the original number, then its additive inverse will be minus of $p$ i.e.,$-p$, such that;

$p+(-p)=p-p=0$

The additive inverse of any given number can be found by changing the sign of it. The additive inverse of a positive number will be negative, whereas the additive inverse of a negative number will be positive. We never change the numeric value of the number except the sign. For example, the additive inverse of 30 is -30.

Additive Inverse Examples:

Additive inverse of 20 is -20 , as $20+(-20)=0$
Additive inverse of 0 is 0
Additive inverse of -19 is 19 , as $(-19)+19=0$

It is also called the opposite of the number or the negation of number.

Additive inverse of -1 is 1
Additive inverse of -5 is 5
Additive inverse of 2/3 is -2/3
Additive inverse of $\frac{-5}{9}$ is $\frac{5}{9}$

Note: Additive inverse of 0 is 0 always.

Additive Inverse Property

According to definition of additive inverse, it is changing the sign of a number, then add it to the original number to get an answer equal to 0.

Let us take $q$ the original number, then its additive inverse is given by $-q$. Following are the properties of additive inverse:

1. $-(-q)=q$
2. $(-q{)}^2=q^2$
3. $-(q+y)=(-q)+(-y)$
4. $-(q-y)=y-q$
5. $q-(-y)=q+y$
6. $(-q)\times y=q\times (-y)=-(q\times y)$
7. $(-q)\times (-y)=q\times y$

Additive Inverse of Different Numbers

In this topic of additive inverse of different numbers, let us look into the additive inverse of natural or whole numbers, rational numbers, complex numbers and algebraic expressions.

Additive Inverse of Natural or Whole Numbers

$$\begin{array}{|ll|}\hline \text{ Whole numbers/Natural numbers }& \text{ Additive Inverse Result }\\ 0& 0& 0+0=0\\ 5& -5& 5+(-5)=0\\ 7& -7& 7+(-7)=0\\ 3& -3& 3+(-3)=0\\ \hline\end{array}$$

Additive Inverse of Complex Numbers

Complex numbers are the combination of real numbers and imaginary numbers. $\mathrm{X}+iY$ is a complex number, where $X$ is the real number and $Y$ is the imaginary number.

Hence, the additive inverse of $X+iY$ should be a value, that on adding it with a given complex number, we get a result as zero. Therefore, it will be - $(\mathrm{X}+\mathrm{iY})$

Example: Additive inverse of $9+4i$ is $-(9+4i)$

$\begin{array}{rl}& 9+4i+[-(9+4i)]\\ & =9+4i-9-4i\\ & =0\end{array}$

Additive Inverse of Rational Numbers

We suppose $\frac{r}{s}$ is a rational number such that the additive inverse of $\frac{r}{s}$ is $-\frac{r}{s}$ and vice versa.

Additive Inverse in Algebraic Expressions

The additive inverse of an algebraic expression is one that makes the sum of all the terms zero. Therefore, the additive inverse of the expression is -(expression). The additive inverse of $x^2+30$ is $-(x^2+30)=-x^2-30$

This is the meaning of additive inverse in algebra.

For example, the additive inverse of $100a+3b$ is $-100a-3b$, thus making the sum of all the elements zero.

Difference Between Additive Inverse and Multiplicative Inverse

Additive Inverse Examples

Example 1: What is the additive inverse of $5/3$ ?
Solution: Given number is $5/3$.
We know that the additive inverse of $\frac{p}{q}$ is $\frac{-p}{q}$.
Hence, the additive inverse of $\frac{5}{3}$ is $\frac{-5}{3}$.

Example 2: What is the additive inverse of -4/9?
Solution: Given number is $\frac{-4}{9}$.
We know that the additive inverse of $\frac{a}{b}$ is $\frac{-a}{b}$.
Hence, the additive inverse of $\frac{-4}{9}$ is $\frac{4}{9}$.

Example 3: What is the additive inverse of $-6/20$ ?
Solution: Let $z$ be the additive inverse.

$\begin{array}{rl}& -6/20+z=0\\ & z=6/20\end{array}$

Example 4: What is the additive inverse of the expression $12x+45y-9z$ ?
Solution: It can be calculated by multiplying the whole equation by -1 .

$-1(12x+45y-9z)=-12x-45y+9z$

Example 5: Find the additive inverse of the fraction $-61/-51$.
Solution: Additive inverse of $\frac{-61}{-51}$ is $-(\frac{-4}{9})=-\frac{61}{51}$

List of Topics Related to Additive Inverse